- Location
- Wisconsin
- Occupation
- PE (Retired) - Power Systems
rattus said:Quote:
Originally Posted by jim dungar
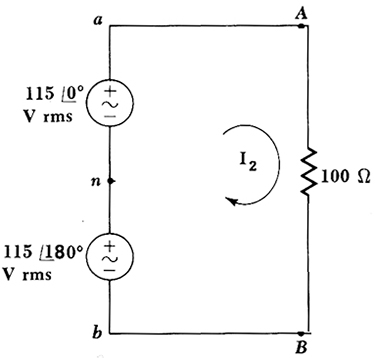
If the current is I12 and it is in phase with one source V1n then it must also be in phase with the other source.
I expect resistive current to be in phase with its source voltage. Yours can not be, especially if the source is a center tapped transformer referenced as you have.
Jim,
But Jim, it can be, e.g.,
I12 = (V1n - V2n)/R = 240/R @ 0
Now V2n = 120 @ 180 which is out of phase with I12.
Compute I21 and its phase angle is 180 degrees.
The basis of the question I posed was: Two voltage sources are in series there is nothing connected to the common reference point "n" except the sources. There is a single resistor connected between points 1 and 2.
So are you saying a single source current I12 which is out of phase with your reference V2n, simply needs to be inverted into I21 so that it is back in phase? If yes, then isn't the new source current I21 now out of phase with V1n?
Or are you saying that the current flowing into a source is always out of phase with the source?
There is a single load resistor, so isn't there a single source current either flowing from 1->2 or from 2->1. The fact that you are using phasors should not change basic 2-wire circuit analysis.
Please save the issue of single phase wye circuits for another discussion.