My question involves an apparent violation of the laws of physics when summing single-pole, 3 wire, loads with other single-pole, 2 wire loads and 3-phase loads. Here is my example, maybe someone can explain the apparent disrepency.
Sample Loads on 3-phase, 208/120VAC, Panel ABC
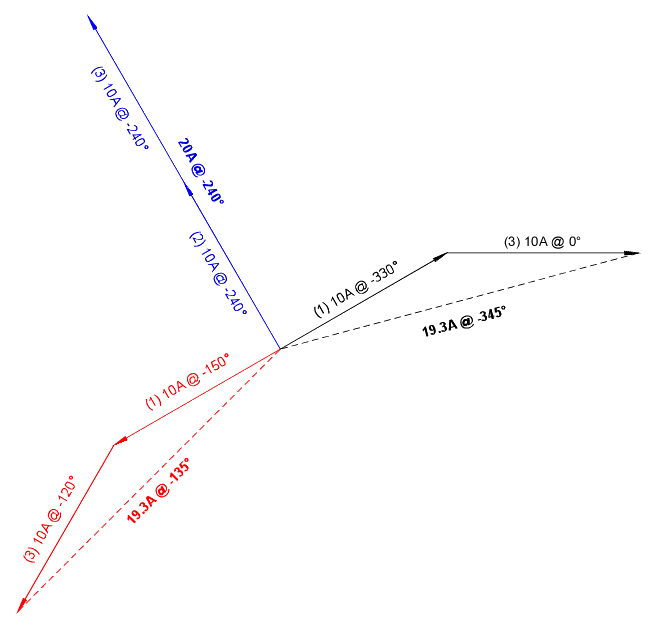
1. One 2080VA @ 208V, single-phase, 3 wire load
2. One 1200VA @ 120V, single-phase, 2 wire load
3. One 3600VA @ 208V, three-phase, 3 wire load
TOTAL Load: 2080+1200+3600 = 6880VA
Ampere Method
Panel A B C
1. 10 10 -
2. - - 10
3. 10 10 10
TOTAL 20 20 20 = (3)(120)(20) = 7200VA
How can there be two different values for the total load on Panel ABC for the same list of equipment?
I hope I explained my point and at least made some of you think =) Let me know what you think?
-Ed
Sample Loads on 3-phase, 208/120VAC, Panel ABC
1. One 2080VA @ 208V, single-phase, 3 wire load
2. One 1200VA @ 120V, single-phase, 2 wire load
3. One 3600VA @ 208V, three-phase, 3 wire load
TOTAL Load: 2080+1200+3600 = 6880VA
Ampere Method
Panel A B C
1. 10 10 -
2. - - 10
3. 10 10 10
TOTAL 20 20 20 = (3)(120)(20) = 7200VA
How can there be two different values for the total load on Panel ABC for the same list of equipment?
I hope I explained my point and at least made some of you think =) Let me know what you think?
-Ed