very helpful!!
very helpful!!
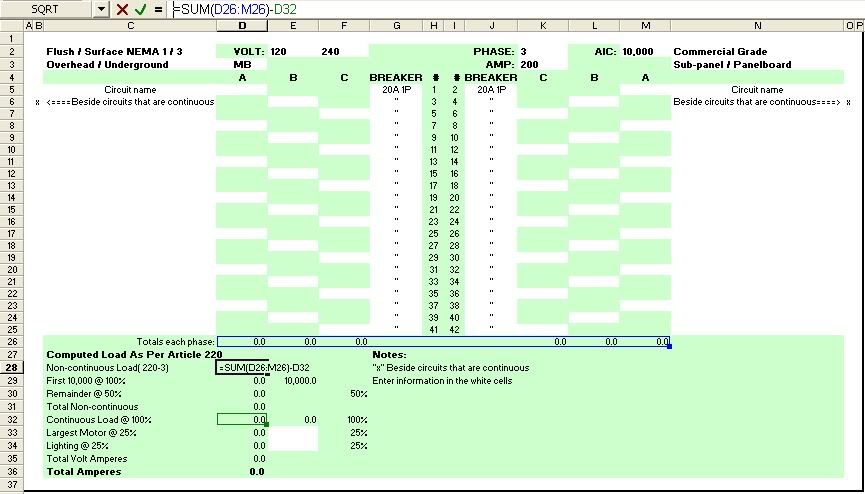
That is where I was going with my question. If you balance the load each phase carries 1.73 times the single phase current.
You also only need to calculate with actual load not MCA marked on the unit. The MCA is for sizing the conductors for the branch circuit and is likely 125% of the compressor RLA plus all other loads in the unit or very close to that. Your feeder will need to be at least 125% of largest plus all others
Lets assume your 30 amps or 6240 VA is the actual load and you have 6 of the same units evenly balanced across all three phases. You have a total 37440 VA.
Divide by 208 gives you total single phase amps of 180 amps. Since you are balancing this across three phases you need to divide that by the square root of 3 (1.73) to get the amps per phase. Which will be about 104 amps. Now your seventh unit will be an additional 30 amps connected to each of the two phases you put it on, so you will have something like
Phase A = 134 amps
Phase B = 134 amps
Phase C = 104 amps
Remember you used the MCA to get your individual load of 30 amps. The actual full load of the unit is probably closer to 21-24 amps. If your seven units are not all the same size you will want to connect them to the phases in a way that comes closest to balancing the loads between the phases for best results as well as allowing a smaller feeder to supply them.
This has been very instructive, but I'm not sure I've got it. If you don't mind, I am going to run thru the whole calculation ...
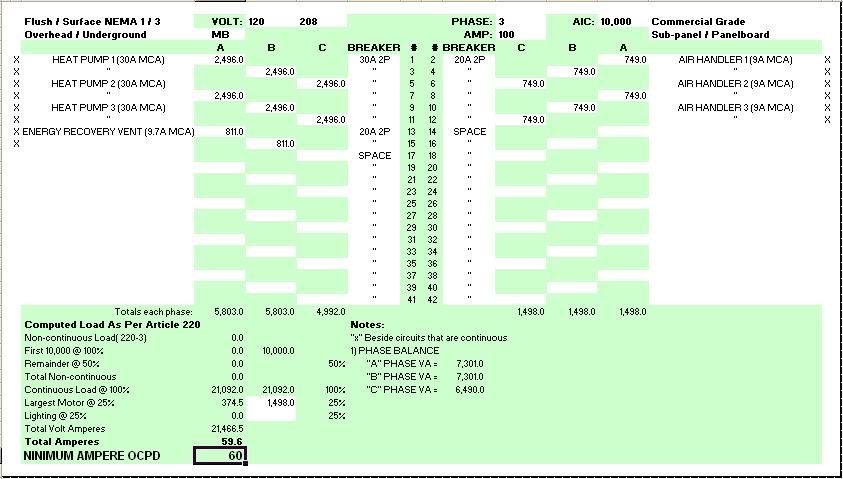
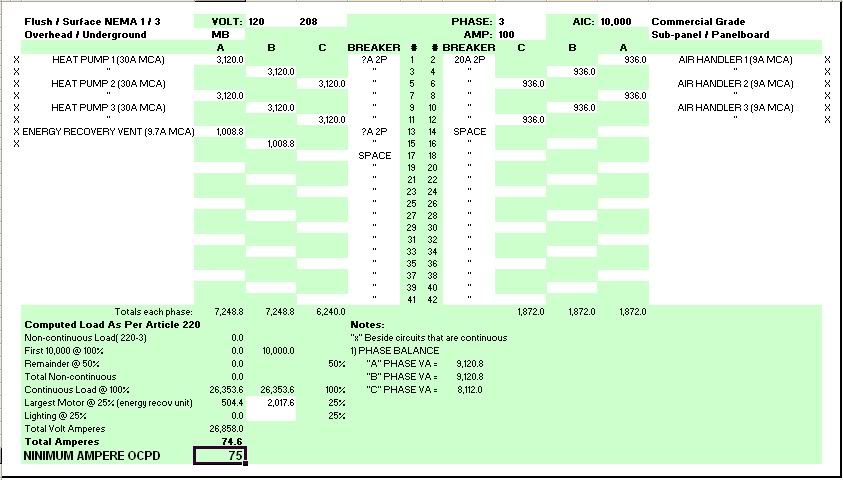
There are (3) 208V/1ph Heat Pumps rated 30 MCA. If I reduce them by .8, and then multiply by 208V, that will give me a 4992VA load to each of two phases.
Next, I have (3) 208V/1ph Air Handlers at 9 MCA. Same calculation results in 1498VA per phase.
Finally, one 208V/1 ph Energy Recovery Ventilator at 9.7 MCA adds 1622VA to each phase connected, which are A & B.
The HPs and AHUs can all be connected to result in a balanced load across all 3 phases. The ERV will produce a larger imbalance in the two phases (A & B) to which it is connected.
Therefore ...
Phase A = 4992+4992+1498+1498+1622 = 14,602VA
Phase B = (same as A) 14,602VA
Phase C = 4992+4992+1498+1498 = 12,980VA
Calculating the balanced portion of the load ...
12,980VA / 208 / 1.73 = 36A on each phase A, B, & C
the unbalanced portion ...
1622VA / 208 = 7.8A on phase A & B.
Compiling balanced & unbalanced amperage results in ...
A = 43.8, B = 43.8, C = 36
Final note ... add in 25% of largest motor of sizing feeder.
The final numbers seem low to me. Did I miss something??