System Input Wattage for Lighting

[AllenC]

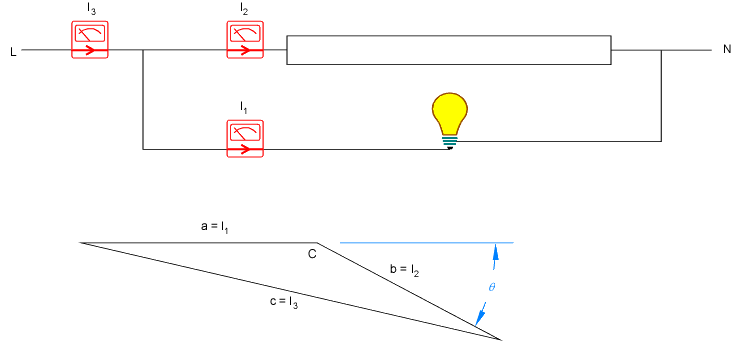
Hi,

I'm confused with how to calculate the actual system input wattage, say for a typical 36W T8 (G13 base) fluorescent lamp with conventional magnetic ballast. The ballast says 0.43A printed on it.

Using a clamp meter, we get 0.43A reading. I'm from Philippines and our voltage is 220VAC.

Input Wattage = Amps x Volts (x Power Factor?)
= 0.43A x 220V = 94.6W

My questions:
1) Do I need to factor in the PF?
2) 94.6W seem a too high power consumption for a typical 36W T8 FL. Is this normal?

Can anyone pls clarify? Thanks a lot!

 

[AllenC]

OK, I've started a new thread topic "System Input Wattage for Lighting".
(Sorry about this. Pls reply using the new thread.)

I'm confused. I need to estimate the actual cost of energy for a particular lighting setup. So I need to get the kWh and use the Watts = Volts x Amps x PF, right?

I'm based in the Philippines and most conventional ballasts here don't have a model number. If I don't have the data from the manufacturer, will my method (using the clamp meter to get the actual amps) and formulas mentioned give me an accurate KWh rate?

Another problem is that I don't have the PF of the ballast. Lets say I'm computing for the actual utility cost of a client's lighting setup and I want to compare the cost when a capacitor is added to correct the PF. So, for the client's present setup, can I get the PF from their latest utility bill and for the setup with capacitor can I just assume a conservative 0.9 PF? Using the formulas mentioned, I'll use the 0.43A for the setup without capacitor, and use 0.22A for the one with capacitor (based on our own testing using a clamp meter). Is this method valid?

Also, I'm still wondering if 0.43A is too high (90+ watts per 36W T8 lamp).

Thanks.

First, you should start your own thread topic.



Yes. Check out the ballast mfrs web site for actual watts.



Yes, if you want watts, not VA. Watts = Volts x Amps x PF



You use VA to size the wiring. You use watts to figure (kilo)watthours that will appear on the utility bill. You shouldn't need to run your own test. The data should be available from major manufacturers.

 

[W6SJK]

All utilities meter kwh so you need to know watts. You need a meter to measure watts, or get the PF by measurement or data from the mfr and use it to multiply V x A x PF.

You are measuring 0.43A at 220 which gives 94.6VA. You need to get the PF somehow, in order to calc the watts.

Why are you concerned with PF? Does your utility penalize for low PF? If not, you will not save on the utility bill by adding capacitors. You will reduce the VA, not the watts. Reducing VA helps regain some capacity in panels to add more loads, but it doesn't affect kW or kWh.

 

[robbietan]

sir allenC,

the 0.43A you measured includes the consumption of the magnetic ballast. the p.f. in your utility bill is the monthly average for the whole load in the plant / building. with the kWHr and kVARHr available, you can get an accurate idea of how much a said amount of capacitance would be needed to boost the p.f. of the plant / building.

and if you live in the Philippines capital city, you may need at least 5 years before a capacitor pays for itself due to the lower discounts offered by the utility on p.f.

 

[AllenC]

All utilities meter kwh so you need to know watts. You need a meter to measure watts, or get the PF by measurement or data from the mfr and use it to multiply V x A x PF.

You are measuring 0.43A at 220 which gives 94.6VA. You need to get the PF somehow, in order to calc the watts.

Is there a meter to measure actuall watts and PF? Or can I just use the average PF stated in the latest billing statement just for estimation purposes?

That is, refering to a single lighting system (i.e. a single 36W T8 lamp and a ballast), I can get the amps using the clamp meter at the client site, assume 220V and get the PF from latest billing. Will this be alright?

Why are you concerned with PF? Does your utility penalize for low PF? If not, you will not save on the utility bill by adding capacitors. You will reduce the VA, not the watts. Reducing VA helps regain some capacity in panels to add more loads, but it doesn't affect kW or kWh.

Am not actually concerned with PF. But I have questions related to PF that need answers:

1) Trying to get an estimate energy consumption for an existing lightin system (as described above) and the savings when changing to a high-power-factor (or electronic) ballast.

2) I'm confused whether to factor in the PF when computing for the Kwh (I guess you already answered this).

3) 94.6W seems too high for a typical lighting fixture. But you said this should be VA and I need to factor in the PF to get Watts, right?

Pls clarify. Thanks for your help, Sparkie!

AllenC

 

[AllenC]

sir allenC,

the 0.43A you measured includes the consumption of the magnetic ballast. the p.f. in your utility bill is the monthly average for the whole load in the plant / building. with the kWHr and kVARHr available, you can get an accurate idea of how much a said amount of capacitance would be needed to boost the p.f. of the plant / building.

and if you live in the Philippines capital city, you may need at least 5 years before a capacitor pays for itself due to the lower discounts offered by the utility on p.f.

Yes, the incentive of having a high PF is small. But will adding a capacitor in the lighting system (or using a HPF ballast or electronic ballast) lower the amps used, therefore translating to energy savings?

Pls take a look at my formula is they are correct at estimating the energy savings:

I metered for the actual amps (using a clamp meter) from a test lighting system (single lamp/conventional ballast) and I got 0.43A.

I metered for the actual amps (using a clamp meter) from a test lighting system (single lamp/conventional ballast with capacitor) and I got 0.21A.

Present System of Client:
36W T8 Fluorescent Lamp with Conventional Ballast
PF from latest utility billing = 74.40% (whole building)
Assuming steady AC voltage of 220V.

Energy Used (Kwh) = V x A x PF = 220V x 0.43A x 74.40% x hours used

Proposed System:
36W T8 Fluorescent Lamp with Conventional Ballast and Capacitor
Assuming high PF = 90%
Assuming steady AC voltage of 220V.

Energy Used (Kwh) = V x A x PF = 220V x 0.21A x 90% x hours used


Then, energy savings = (Present KWh - Proposed KWh) x energy rate x # of lamps.

Is this valid?

Thanks very much for your help.


AllenC

 

[AllenC]

sir allenC,

the 0.43A you measured includes the consumption of the magnetic ballast. the p.f. in your utility bill is the monthly average for the whole load in the plant / building. with the kWHr and kVARHr available, you can get an accurate idea of how much a said amount of capacitance would be needed to boost the p.f. of the plant / building.

and if you live in the Philippines capital city, you may need at least 5 years before a capacitor pays for itself due to the lower discounts offered by the utility on p.f.

Yes, the incentive of having a high PF is small. But will adding a capacitor in the lighting system (or using a HPF ballast or electronic ballast) lower the amps used, therefore translating to energy savings?

Pls take a look at my formula is they are correct at estimating the energy savings:

I metered for the actual amps (using a clamp meter) from a test lighting system (single lamp/conventional ballast) and I got 0.43A.

I metered for the actual amps (using a clamp meter) from a test lighting system (single lamp/conventional ballast with capacitor) and I got 0.21A.

Present System of Client:
36W T8 Fluorescent Lamp with Conventional Ballast
PF from latest utility billing = 74.40% (whole building)
Assuming steady AC voltage of 220V.

Energy Used (Kwh) = V x A x PF = 220V x 0.43A x 74.40% x hours used

Proposed System:
36W T8 Fluorescent Lamp with Conventional Ballast and Capacitor
Assuming high PF = 90%
Assuming steady AC voltage of 220V.

Energy Used (Kwh) = V x A x PF = 220V x 0.21A x 90% x hours used


Then, energy savings = (Present KWh - Proposed KWh) x energy rate x # of lamps.

Is this valid?

Thanks very much for your help.


AllenC

 

[Smart $]

Pls take a look at my formula is they are correct ...
PF from latest utility billing = 74.40% (whole building)

You cannot use the pf of the whole building as it is not representative of the lighting system pf.

Short of having a "true" wattmeter, you can get a single fixture pf through a test setup. Connect in parallel a resistive-only load of near-equal current (best for result accuracy)... in this case, a 100W incandescent will do. Measure individual and combined currents as in the following diagram, then substitute the values into the triangle diagram. Use the Law of Cosines to determine the angle C. Its supplementary angle is θ, where cos θ = pf of the flourescent fixture.

 

[winnie]

Short of having a "true" wattmeter, you can get a single fixture pf through a test setup. Connect in parallel a resistive-only load of near-equal current (best for result accuracy)

Bravo! A test setup that uses equipment reasonably expected to be available. This does depend upon using a 'true RMS' current clamp, and probably doesn't deal well with harmonic current, but it certainly would be the right first test.

A longer term, more expensive and more time consuming, but more reliable test would be to get a kWh meter and mount it to supply a _single_ lamp, and measure the number of kWh used over a given time period, say 1-10 hours.

AllenC: Power factor is a method used to represent the fact that in an AC circuit the current that flows is not necessarily perfectly in step with the voltage applied.

Remember that when you measure the 'voltage' or 'current' in an AC circuit, you are measuring averages over time. Only if the voltage and current are exactly in step will V * I = {average power}

For 'inductive' loads such as motors, the current flow will be a nice sine wave, simply out of step with the voltage. These loads actually store some energy in a magnetic field during part of the AC cycle, and deliver this energy back to the power line during another part of the AC cycle. This energy flowing back and forth is the 'reactive power', and the reason that the current flow is higher than needed to deliver 'real power'. Inductive loads can have their power factor corrected using a capacitor.

However other loads, in particular electronic loads, can have current flow that is not a nice clean sine wave. These loads also have a 'power factor', in that V * I does not equal {average power}, however correcting the power factor of such loads cannot be done with a simple capacitor.

-Jon

 

[AllenC]

You cannot use the pf of the whole building as it is not representative of the lighting system pf.

That's what I was afraid of.

So let me know if I got this right: getting lower amps (using a clamp meter) does not necessarily translate to lower energy consumption???

Is there any other way to get an estimate on energy savings in using a capacitor or HPF ballast using the given data I mentioned above?

I also need to explain in more simpler terms (and calculations) to the client.


Thanks a lot!
Allen

 

[Smart $]

That's what I was afraid of.

So let me know if I got this right: getting lower amps (using a clamp meter) does not necessarily translate to lower energy consumption???

That is correct. However, regarding your test with a capacitor, yes you most likely improved the power factor, and lowered the energy consumption a bit... but running a fixture with such power factor improvement will most likely lead to shortened ballast and lamp life. While I can't say from first hand experience, I'm going to assume a balance between ballast/lamp life and energy efficiency was engineered into the design.

Is there any other way to get an estimate on energy savings in using a capacitor or HPF ballast using the given data I mentioned above?

I also need to explain in more simpler terms (and calculations) to the client.

Well, the calculations aren't going to get any simpler... so you can make them and present them, but focus on the kWh usage, and costs?which, BTW, also include estimated maintenance costs over time for existing fixtures and ROI associated with the new eqiupment.

PS: the right side of your kilowatt-hour formulas above calculate out to watt-hours instead.

 

[AllenC]

OK, I got new ballasts to test. This time they have complete spec labelled on it. Supposing 100 units of 2 x 36W fixtures for 1,000 hours usage:

Magnetic Ballast (for 1x36W):
I = 0.43A
PF = 0.50
KWh = 220V x 0.43A x 0.50 x 1,000 hrs x 200 lamps / 1000 = 9,460 KWh


Electronic Ballast (for 2x36W):
I = 0.32A
PF = 0.97
BF = 0.95
KWh = 220V x 0.32A x 0.97 x 1,000 hrs x 100 fixtures / 1000 = 6,828.80 KWh

To compute theoretical savings from using HPF electronic ballast:
= (9,460 - 6,828.80) x energy rate

Is everything alright above?

I did not use any actual clamp reading. Everything was taken from the ballast spec, except for the constant voltage supply which I am just assuming.

Thanks.

 

[Smart $]

...

Is everything alright above?

You may want to use a number of hours more typical of the billing. For instance, you are using 1000 hours and a billing month on average has 30 days, or 720 hours (at least it does in the US :grin: ), and that would be operating the lights 24/7. Personally, I'd want to see the final figure in how much on average I'll save per month and year.

[edit to add] Perhaps give all the numbers a unit. For example, the odd "1000" could be written as ?1000 Wh/kWh, or ?0.001 kWh/Wh ....and hours can be written, say 500 hrs/month... JMO