electrofelon
Senior Member
- Location
- Cherry Valley NY, Seattle, WA
- Occupation
- Electrician
I come up with 2/sqrt 6 (.8165) times rms equals peak voltage for three phase? Does anyone concur?
Snorks said:Hi Electrofelon,
For AC current; Vpeak = V * Sqrt(2)
electrofelon said:What would the peak voltage be on the dc side of a three phase full wave rectifier bridge?
That depends on the voltage reference. If the voltage reference is the 3? system neutral, the output of a full-wave rectifier will be out-of-phase positive "hills" and negative "valleys" at 180Hz.charlie b said:...So what the output signal will look like is a sine wave for which the negative half has been flipped to the positive side, giving you a series of hills.
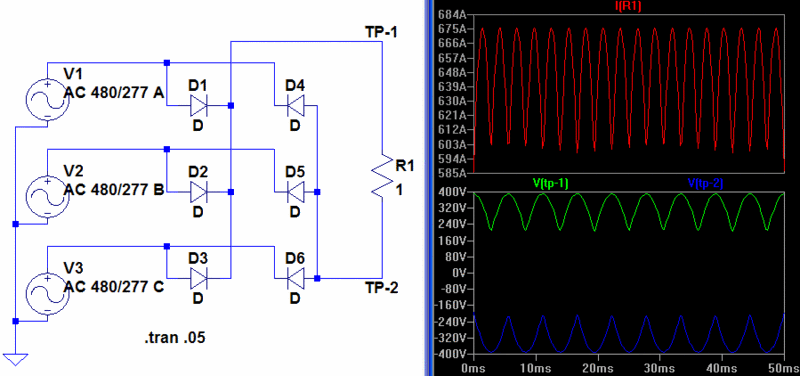
Smart $ said:That depends on the voltage reference. If the voltage reference is the 3? system neutral, the output of a full-wave rectifier will be out-of-phase positive "hills" and negative "valleys" at 180Hz.
If the output voltage is just measured across the output, it will be as you have said with the AC component at 360Hz.

Is there a reason you quoted my post? I was discussing waveform. How did we get back to calculations?rattus said:If we use ideal rectifiers, the peak voltage across the load, in this case, will be,
Vpkload = 480Vrms x sqrt(2) = 679V
Can't get away from sqrt(2)!
Yes, your diagram illustrates my point. Although peak voltage may be a misnomer here.Smart $ said:Is there a reason you quoted my post? I was discussing waveform. How did we get back to calculations?
