Unbalanced neutral Current

[ninocarollo]

Can you derive the unbanced neutral equation below. I am teaching a class and would like to derive the equation for the class. I believe it is the vector summation of Ia+Ib+IC but I am having a hard time deriving it..
Do I assume the Current phases are 0,120,240 ?


In=Sqare root(((Ia)squared+(Ib)squared+(Ic)squared)-((IaIb)+(IbIc)+(IcIa))

 

[charlie b]

Re: Unbalanced neutral Current

I asked that question several months ago. Some other member, and forgive me I do not recall who, posted the following "proof." I copied it to my hard drive, so that I could look at it in detail at my leasure. But I have not had a chance to go through it, so I cannot bear witness to its validity. But here it is. If its author sees this post, please feel free to jump in and claim credit (or take the blame, if it proves to be invalid ).

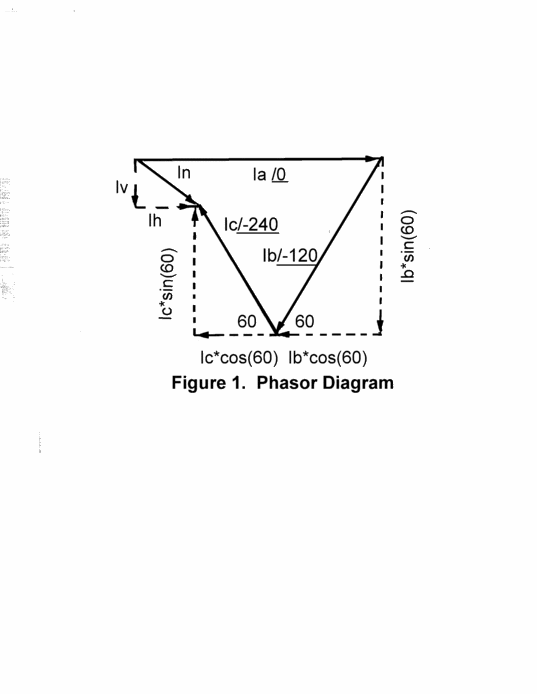
Derivation of formula for neutral current

The basis for the formula is the "vector" addition In = Ia + Ib + Ic. I put vector in quotes, because it is really a phasor. They can be represented in polar format (magnitude and angle) and rectangular format (real and imaginary) as complex numbers. The "vector" addition is really the addition of complex numbers. To get just the magnitude of In you take the square root of the real part squared plus the imaginary part squared.

The real part of X at Y degrees is X cos Y and the imaginary part is X sin Y. So the magnitude of In is (using capital for magnitude and small for the phase angle, and just ^ for squared):

sqrt( (Acosa+Bcosb+Ccosc)^ + (Asina+Bsinb+Csinc)^)

Then multiply the squares out to get terms like
A^cos^a + 2AcosaBcosb + etc

Then group the common parts for A, B, and C, like
A^(cos^a+sin^a) + 2AB(cosacosb+sinasinb) + etc

then use trig identities: sin^x + cos^x = 1
and sinxsiny+cosxcosy = cos(x-y)

and you get:

sqrt(A^ + B^ + C^ + 2ABcos(a-b) + 2BCcos(b-c) + 2ACcos(a-c) )

This is general for any angles. Assume the angles are a=0, b=120, and c=-120 (no attempt to match actual wiring practices for the angles ), plug those numbers in and you get (all the cos are -0.5):

sqrt(A^ + B^ + C^ - AB - BC - AC)

Q.E.D.

 

[rattus]

Re: Unbalanced neutral Current

Nino,

I have just been through this exercise and will post it later today. You can assume any set of phase angles you wish as long as they are separated by 120 degrees.

Rattus

 

[rattus]

Re: Unbalanced neutral Current

Here is my derivation:

This may be more than you want to know, but I am on a roll and can?t stop.

Derivation of the Neutral Current Formula

Assume three unequal phase currents, Ia, Ib, & Ic, with the same PF. That is, they are separated by 120 degrees. For convenience, we assign a zero phase angle to Ia, -120 to Ib, and ?240 to Ic. The phasor diagram then appears as in Figure 1. Note that the sum of the phasors is not zero. This sum is the neutral current, In.

Now we break Ib and Ic into their horizontal and vertical components. The sum of the horizontal components is,

1) Ih = Ia ?0.5(Ib + Ic)

The sum of the vertical components is,

2) Iv = (sqrt3/2)(Ic ? Ib)

The magnitude of In is then,

3) In = sqrt(Ih^2 + Iv^2)

If anyone is that interested, they should substitute eqns. 1 & 2 into eqn. 3 to check it out. You must watch your signs though. Sam, how about you?

 

[rattus]

Re: Unbalanced neutral Current

C. B.'s general formula appears to be correct, but in my opinion it is a bit clumsy. Since we have to use trig anyway to evaluate the general formula, I would prefer to do it this way:

For convenience we let the phase angle, a, of Ia be zero degrees. Then the horizontal (real) component of current is,

Ih = Ia*1 + Ib*cos(b) + Ic*cos(c)

and the vertical (imaginary) component is,

Iv = Ia*0 + Ib*sin(b) + Ic*sin(c)

where a, b and c are the actual phase angles of Ia, Ib, and Ic.

The magnitude of In can now be obtained from the Pythagorean Theorem,

In = sqrt(Ih^2 + Iv^2)

(In and its phase angle can also be determined with trig, but this is a bit tricky.)