You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Voltage Drop Formula
- Thread starter ault1910
- Start date
- Status
- Not open for further replies.
- Location
- Illinois
- Occupation
- retired electrician
Roger,
Those are on the high side for most applications. Those are the constants based on a 90?C operating temperature. At 60?C they would be 10.8 and 17.
Those are on the high side for most applications. Those are the constants based on a 90?C operating temperature. At 60?C they would be 10.8 and 17.
Don, I agree with you, I posted the numbers above because they are the general numbers used in most class rooms and exams.Roger,
Those are on the high side for most applications. Those are the constants based on a 90?C operating temperature. At 60?C they would be 10.8 and 17.
Roger
jumper
Senior Member
- Location
- 3 Hr 2 Min from Winged Horses
Don, I agree with you, I posted the numbers above because they are the general numbers used in most class rooms and exams.
Roger
Yep. Every one I have taken.
Roger,
Those are on the high side for most applications. Those are the constants based on a 90?C operating temperature. At 60?C they would be 10.8 and 17.
Is there a formula to calculate the resistivity constants based on temperature?
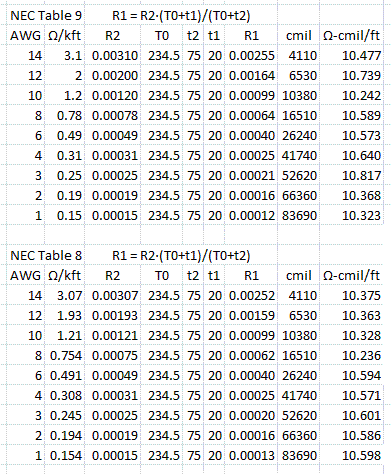
For general use, Copper is 10.371 ohm-circular mil/ft at 20?C plus/minus 0.0409 ohm-circular mil/ft. per ?C change.Is there a formula to calculate the resistivity constants based on temperature?
For general use, Copper is 10.371 ohm-circular mil/ft at 20?C plus/minus 0.0409 ohm-circular mil/ft. per ?C change.
I am not sure that is correct. I get 13.234 at 90?C
Well there has been some changes in values over the years. The current base value can range from 10.371 to 10.2. Also, many use 0.039 per ?C but that value does not account for the change in volume that occurs with a change in temperature.I am not sure that is correct. I get 13.234 at 90?C
10.2 + (90 - 20) ? 0.039 = 12.93
10.371 was the base value before those SI people started messing things up :jawdrop:
Last edited:
Hmmm.... and what are given as the values of R1 and t1.R2 = R1?(T0+t2)/(T0+t1)
Where T0 = 228 for aluminum, 234.5 for annealed copper, 241 for hard-drawing copper
t1 and t2 are in ?C
Source: Elements of Power System Analysis, William D. Stevenson, Jr., 2nd Edition, 1962.
PS: my source was http://library.bldrdoc.gov/docs/nbshb100.pdf
Hmmm.... and what are given as the values of R1 and t1.
R1 is the resistance at temperature t1. R2 is the resistance at temperature t2. The same would apply to resistivity or voltage drop constants that are proportional to resistivity. Maybe it is slightly different for resistivity because of thermal expansion, but not significantly.
See the notes at the end of Table 3 in your reference. The last equation is the same as Stevenson's, using the values of T in Table 3. T = 241 for 97.5% conductivity (hard-drawn) and 234.5 for 100% conductivity (annealed).
The advantage is if you know the resistance at some temperature other than 20?C, e.g. from NEC Table 9, Stevenson's equation is easier for calculating the resistance at a different temperature.
...and this leads to errors, as noted in the reference I linked. For example, if you backfigure R1@20?C from NEC Tables 8 and 9, then convert to ohm-cmil/ft, you see what I mean...R1 is the resistance at temperature t1. R2 is the resistance at temperature t2. The same would apply to resistivity or voltage drop constants that are proportional to resistivity. Maybe it is slightly different for resistivity because of thermal expansion, but not significantly.
See the notes at the end of Table 3 in your reference. The last equation is the same as Stevenson's, using the values of T in Table 3. T = 241 for 97.5% conductivity (hard-drawn) and 234.5 for 100% conductivity (annealed).
The advantage is if you know the resistance at some temperature other than 20?C, e.g. from NEC Table 9, Stevenson's equation is easier for calculating the resistance at a different temperature.
...and the question was, "Is there a formula to calculate the resistivity constants based on temperature?"
But the difference between your calculated values and 10.371 are very small, considering that the table values are only given to three significant figures, or can be explained by the fact that Table 9 is ac ohms and #6 AWG and larger are stranded in Table 8 (you have an error in ohm/kft for #8)....and this leads to errors, as noted in the reference I linked. For example, if you backfigure R1@20?C from NEC Tables 8 and 9, then convert to ohm-cmil/ft, you see what I mean...
The only values which correspond to a K of 10.371 are those for solid wire in Table 8. For stranded in Table 8, I wouldn't call a departure of 2% small, considering the implications of the result.But the difference between your calculated values and 10.371 are very small, considering that the table values are only given to three significant figures, or can be explained by the fact that Table 9 is ac ohms and #6 AWG and larger are stranded in Table 8 (you have an error in ohm/kft for #8).
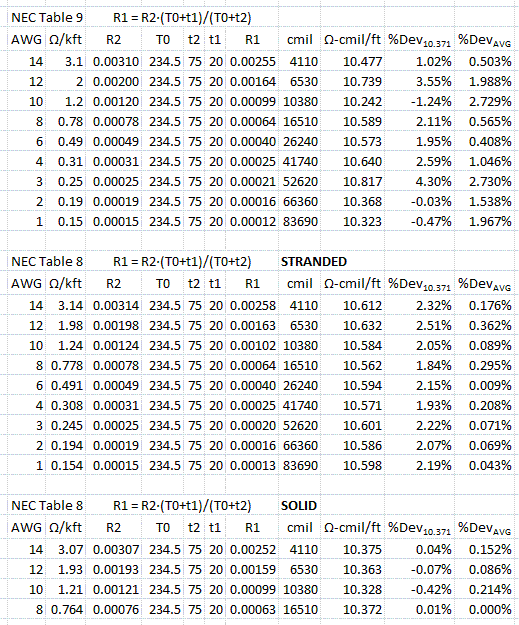
However, being in error depends on the final objective (i.e. values of K vs. the accuracy of a Vd calc result). The true anomaly is that "K" is not so constant as one is led to believe. The short of it is: for AC Vd calculations, using the "RID" formula and Table 9 R or R and Z values yields a more accurate result.
- Status
- Not open for further replies.

