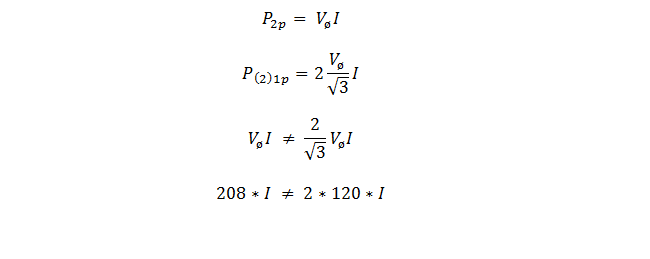Becca R
Member
- Location
- East Peoria, Illinois, United States
Is the wattage of two 120 V circuits the same as one 2 pole circuit with the same current? When I tried to work the math, it shows that the wattage of the 2 pole circuit does not equal the wattage of the two single pole circuits (see pic). You would think that if both single pole circuits had the same amperage as the 2 pole circuit, the power should be the same in the 2 pole circuit as in the two single pole circuits. Did I make a mistake with my math or are these two circuits not equivalent?