Mike,
See if these graphics help clarify what myself & others have been saying:
The first graphic shows the instantaneous forces in a transformer. For our discussion, let polarity marks (not shown) indicate the up direction as positive.
For half of the time the forces are in one direction, and for the other half the forces are in the opposite direction. The left side of the graphic shows the force from one end of the winding and how they sum across the winding to double the force. As you can see, the direction of force only agrees with the polarity mark half the time. As I have said, direction and polarity are not the same thing.
The right side shows the voltages taken from the center (required two graphs because the waves lay on top of each other). If you take them in the same direction, you get the same composite as the first graph.
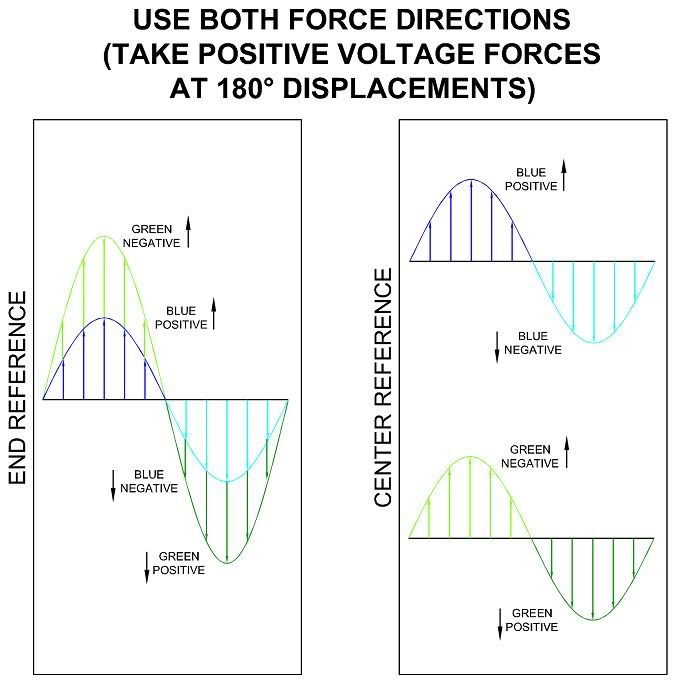
The next graphic shows what happens when we take the positive forces all in the same direction using the polarity marks. This gives us "positive forces" and "negative forces" (i.e., positive and negative voltages).
No matter what direction we take to be positive or negative, the forces in the same direction still give us double the force. In this case, we are saying the positives are 180? displaced from the negatives. As you can see, the direction we call positive always matches the polarity marks.
We take both positive voltages at the same wave point, so the voltages are "in-phase". They only match the force direction half the time.
This final graphic shows what happens when we take the "positive force" of half of the winding to be in one direction and the "positive force" of the other winding to be in the opposite direction (one with polarity, one against polarity). This gives us "positive forces" and "negative forces".
No matter what direction we take to be positive or negative (even though we take it differently for each winding half), the forces in the same direction still give us double the force.
In this case, we take one positive voltage 180? displaced from the other positive voltage so they are "phase-opposed". As you can see, the direction we call positive for one wave always matches the polarity mark. The direction we call negative for the other wave always matches the polarity mark.
No matter how we take the voltages from the transformer, or which direction (or directions) we call positive, the forces inside the transformer do the same as they would do otherwise.
We see that we can take either direction to be a positive direction because it is just a matter of timing. There is no universally "correct" direction.
We can produce these exact same forces with two generators in phase. Then we might claim there is only one correct direction.
We can produce these exact same forces with two phase-opposed generators. Then we might claim both directions are correct.
We can produce these exact same forces with two generators in phase but phase-opposed to other generators that are in phase. Then we might claim this new direction is the only one correct direction.
That is why the laws of electricity do not specify the "correct" direction for taking voltages because if they did, they would eventually produce a paradox. It just ain't going to happen and if anyone tries to make you believe the laws say you can only take voltages in one "correct" direction, they do not understand how things really work.
In truth, the direction is oscillating and our choice as to how we will take the voltages from the source is arbitrary. None of the directions we choose will contradict any electric law. If you think they do contradict the laws, you are trying to make the formulas or laws say something they do not say.