Defining Average Power
- Thread starter dnem
- Start date
- Status
Now you're talking my language.
Going all the way back to post #23 brings in my original question
Now you guys can continue to post formulas back and forth as much as you want but I would place money on the fact that they are sailing over many peoples heads, and I am definitely included in that group.
A German woman and a German man were biological parents of a baby but the baby wasn't German
Can this be explained without resorting to formulas or engineer talk.?
RMS current * RMS voltage gives average power.? Now youre talking like the text books. If you had said, average current * average voltage gives average power and average power is not a quantity that is of any use, that would end the conversation there. But when you say, RMS current * RMS voltage gives average power, my ears hear, a German woman and a German man were biological parents of a baby but the baby wasn't German.
Now you guys can continue to post formulas back and forth as much as you want but I would place money on the fact that they are sailing over many peoples heads, and I am definitely included in that group.
A German woman and a German man were biological parents of a baby but the baby wasn't German
Can this be explained without resorting to formulas or engineer talk.?
You can't just multiply Vrms and Irms and get power.
Carultch
Senior Member
- Location
- Massachusetts
"RMS current * RMS voltage gives average power" is only true for the special case of the simplest kind of AC load you could have. I need to resort to engineer speak to explain what I mean by simple. Identical waveform shapes with no phase shift or distortion between voltage and current, which means a resistive-only load.
"A German woman and a German man were biological parents of a baby but the baby wasn't German."
If German means citizenship and not ancestry, than of course this is possible. The parents were both German by citizenship, but the baby was born in a different country. It is common outside the English language, that identifying a person by a country refers to citizenship, and not ancestry.
Last edited:
- Location
- Placerville, CA, USA
- Occupation
- Retired PV System Designer
Closest I can come to avoiding formulas:
When you take an average of quantity, there are certain manipulations that you can make. For example, if I multiply all of the numbers by a constant, the average of the new numbers is identical to that constant times the average of the old number.
If I add two sets of numbers, the average of the sum is equal to the sum of the averages.
What is common to all of these is that I am performing linear operation on my starting numbers.
If, instead, I do the non-linear operation of multiplying two sets of numbers the product of the averages is not the average of the products.
Simple example. First set [1,-1] Second set [1, -1] .
The average of the first set is 0. The average of the second set is 0. Now multiply each number in the first set by the corresponding number in the second set.
That product gives us the set [1,1]. The average of the products is 1. The product of the averages is 0.
For now lets leave immigration and Germany out of it.
That's political. :angel:winnie
Senior Member
- Location
- Springfield, MA, USA
- Occupation
- Electric motor research
First remember that RMS current * RMS voltage = average power only in the specific case of a resistive load, like an incandescent lamp.
RMS is not some strange beastie; it is a specific type of average. 'mean' is a different type of average, what we commonly think of when we was 'average' These two different types of average are not so different. You are multiplying a type of average voltage times a type of average current and getting a different type of average power.
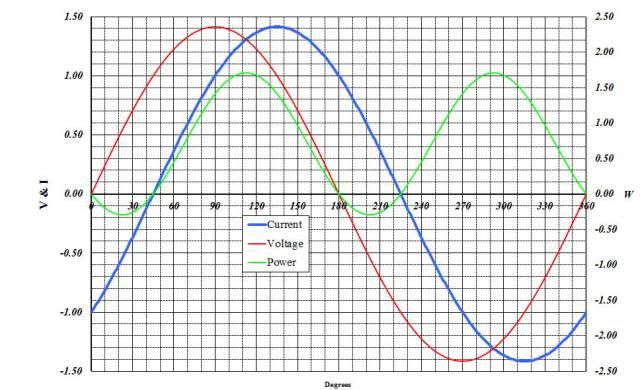
It would probably help to get rid of all of the sine curves and look at DC power to a resistor. The power delivered to a resistor is V^2/R; the power is proportional to the square of the voltage. 1V applied to a 1 ohm resistor causes 1A of current to flow and to deliver 1W to the load. Take the voltage up to 10V and you get 10A and 100W.
Now consider a simple 'changing DC' situation, say a system that alternates 1V for 1 second and 10V for 1 second, a 'square wave' going 1V...10V...1V...10V....
What is the average voltage over 60 seconds? 5.5V ( (30 * 1 + 30 * 10) / 60 )
What is the _average_ power delivered to a 1W resistor over 60 seconds? 50.5W ( (30 * 1 + 30 * 100) / 60 )
Notice that in figuring out the average power you have a the voltage _squared_ as one of the terms. Power is simply volts * amps, and in a resistor amps are proportional to volts, so power is proportional to volts^2.
In our example above, the RMS voltage? 7.106V ( sqrt( (30 * 1 + 30 * 100)/60) )
Notice the same average of the sum of squares ( (30 * 1 + 30 * 100)/60) in both the power and the RMS calculation. This is where RMS has use. The RMS average compensates for the square law scaling of output power with voltage. For a resistive load, the average power dissipated when a given RMS voltage is applied is the same as when the same DC voltage is applied.
Remember: this is only true for loads that look like resistors; the concept of RMS throws away phase information, so for loads that aren't resistors there are considerations of power factor and the like.
"The RMS average compensates for the square law scaling of output power with voltage"
I will have to "sit" on this phase for a few days and contemplate. At some point life will calm and I will really be able to think this thru. I believe that the key to unlocking this concept is in that phase you posted (and I quoted) above. Unlike the math oriented brain types (that get something out of staring at formulas), I need to ponder specific concepts to get to my "light bulb" moment.
- Location
- Placerville, CA, USA
- Occupation
- Retired PV System Designer
As long as you consider only the case of a resistive load, I think you will do just fine with your pondering.
Instantaneous Power=V2/R is a good start.
And since you get the time average of the instantaneous power by averaging V2/R, that is the same as dividing the average value of V2 by R.
And the average value of V2 is by definition VRMS2.
A 1W resistor can dissipate that amount of power for a minute...???
Perhaps you can fit I²R in there somewhere... :happyyes:
- Location
- Placerville, CA, USA
- Occupation
- Retired PV System Designer
Sure. I covered why to use RMS voltage.
Just substitute I2R for V2/R and the same argument works.
Now the tricky part comes when you take either result and see that since I=V/R then Irms = Vrms/R and then it is also true that Power = Vrms x Irms.
Carultch
Senior Member
- Location
- Massachusetts
This can be kind of confusing. Resistors are rated both in Watts and in Ohms, both of which are important to the application. And unfortunately, the Omega symbol for Ohms, is the letter W in the Greek alphabet font. So when that font doesn't come through, the letter that is supposed to be Omega, shows up as W.
The Ohm rating specifies resistance R, which is how voltage across the component determines the current through it. The proportionality constant between the two. The Watt rating on a resistor specifies how much total power can be safely dissipated in that component, before you can expect it to burn. The maximum value of V^2/R or I^2*R.
When an incandescent lighbtulb or resistance heater is rated in Watts, it really is only valid at the nominal voltage it is supposed to use for operation. They are giving you the value of V^2/R, so the actual resistance is R = Watts/Volts^2.When an incandescent lighbtulb or resistance heater is rated in Watts, it really is only valid at the nominal voltage it is supposed to use for operation. They are giving you the value of V^2/R, so the actual resistance is R = Watts/Volts^2.
>Ω<
Testing whether unicode "omega" come through? I did not set any font so should display in your browser's default font...
winnie
Senior Member
- Location
- Springfield, MA, USA
- Occupation
- Electric motor research
As has been noted, I fat-fingered the above. The question should read 'what is the _average_ power delivered to this 1 Ohm resistor over 60 seconds?'
-Jon
jumper
Senior Member
- Location
- 3 Hr 2 Min from Winged Horses
- Location
- Placerville, CA, USA
- Occupation
- Retired PV System Designer
There are several ways to represent non-Latin characters in a web page.
One is to change fonts to a font in which the character desired is a standard character (single byte representation.)
That runs into problems with some web servers and some browsers. It was once the only way to do it in Windows.
Another is to make use of so-called Upper ASCII byte values in a font which contains such extended characters. That does not always work either.
The most compatible is to represent that character using Unicode, in a variable width character representation such as UTF-8. Essentially all modern browsers will accept this and display it correctly, but not all web servers will allow you to enter characters in that format in the first place.
And finally, there is the "character entity" representation, which uses an escape sequence of many bytes to tell what the character is and leave it up to the browser to figure out how to display it. Again, not all web servers let you enter these, though they may use them to render a page.
Its a crap shoot.
jumper
Senior Member
- Location
- 3 Hr 2 Min from Winged Horses
- Location
- Placerville, CA, USA
- Occupation
- Retired PV System Designer
Or you can copy and paste like I did.
Works for me. As long as you have someplace to copy from.
jumper
Senior Member
- Location
- 3 Hr 2 Min from Winged Horses
Works for me. As long as you have someplace to copy from.
Me lazy.
Wiki_Greek_letters_used_in_mathematics,_science,_and_engineering
- Status