is this right?
- Thread starter jwest
- Start date
- Status
crossman
Senior Member
- Location
- Southeast Texas
Re: is this right?
Teach them both.
I calculated K from the NEC for several different wire sizes:
K = 12.87 for #14
12.88 for #6
12.87 for #2
12.85 for 3/0
12.88 for 800 kcmil
So..... using 12.87 for K in R = K x l/cma will give you a very close answer to what you would get using the Code tables.
Funny though, in my apprenticeship, I remember we used K for copper of 10.4 and sometimes 10.7
Teach them both.
I calculated K from the NEC for several different wire sizes:
K = 12.87 for #14
12.88 for #6
12.87 for #2
12.85 for 3/0
12.88 for 800 kcmil
So..... using 12.87 for K in R = K x l/cma will give you a very close answer to what you would get using the Code tables.
Funny though, in my apprenticeship, I remember we used K for copper of 10.4 and sometimes 10.7
crossman
Senior Member
- Location
- Southeast Texas
Re: is this right?
Take the code book "ohms per 1000 feet" and multiply it by the circular mil area and then divide by 1000
and in general for copper: K = 12.87 ohms x cma / feet
From right there^
Take the code book "ohms per 1000 feet" and multiply it by the circular mil area and then divide by 1000
and in general for copper: K = 12.87 ohms x cma / feet
crossman
Senior Member
- Location
- Southeast Texas
Re: is this right?
Okay Physis, I am going to stick this one out to the end. The unit for K is NOT ohms.
The unit for K is (ohms x circular mil area)/feet
The canceling effect of the units on the right hand side of the equation ends up giving ohms on the left side of the equation.
Okay Physis, I am going to stick this one out to the end. The unit for K is NOT ohms.
The unit for K is (ohms x circular mil area)/feet
The canceling effect of the units on the right hand side of the equation ends up giving ohms on the left side of the equation.
crossman
Senior Member
- Location
- Southeast Texas
Re: is this right?
Just to make sure....
Is it your assertion that K is in Ohms?
Just to make sure....
Is it your assertion that K is in Ohms?
crossman
Senior Member
- Location
- Southeast Texas
Re: is this right?
So if you are strictly defining K by
1) resistance
2) length
3) circular mil area
then would you believe that all three of those parameters must be in the definition of K and hence in the unit that defines K?
See, when you say that an arbitrary conductor or load or resistor has a resistance of x ohms, you are not making any statement about length, circular mil area, or anything else, an ohm is just an ohm.
But when you strictly define K to be the resistance of a 1 circular mil wire that is 1 foot long, then any use of K in a mathematical formula must include that precise definition.
For real.
I am working on a post to show exactly what is going on here... give me a while...
So if you are strictly defining K by
1) resistance
2) length
3) circular mil area
then would you believe that all three of those parameters must be in the definition of K and hence in the unit that defines K?
See, when you say that an arbitrary conductor or load or resistor has a resistance of x ohms, you are not making any statement about length, circular mil area, or anything else, an ohm is just an ohm.
But when you strictly define K to be the resistance of a 1 circular mil wire that is 1 foot long, then any use of K in a mathematical formula must include that precise definition.
For real.
I am working on a post to show exactly what is going on here... give me a while...
crossman
Senior Member
- Location
- Southeast Texas
Re: is this right?
Okay, let's do a sample problem.
Say we have a copper wire that is 920 feet long and has a cross sectional area 0f 26,500 circular mils.
Now it is tempting to ignore the units and just use the numbers
K = 12.87
L = 920
cma = 26,500
certainly we can do the math and ignore the units and the numbers will work out correctly because someone else has already taken the trouble to build the formula so that it works out properly.
On that note, if all you want to do is crank out the numbers and you don't mind missing out on the inner beauty of physical quantities and the math, that is fine and you can stop reading here.
But if you want to understand physical quantities a bit deeper, keep reading.
For the copper wire, we will say
K = 12.87
L = 920
cma = 26500
Now, in reality, the numbers given above are not simply numbers. They are physical quantities. And physical quantities must be defined by units. And these units must be included in the formulas.
So from above,
L = 920 FEET
cma = 26,500 circular mils
and let us just assume for the sake of demonstration that
K = 12.87 ohms
R = K x L / cma
R = 12.87 ohms x 920 feet / 26,500 circular mils
so R = .444 ohms x feet / circular mils
The feet and circular mils do not just magically diassappear when you do the math. No, they have to be accounted for. And since R on the right side should be in Ohms, not in ohms x feet /circular mils, it becomes obvious that K must have units other than ohms.
For the units to work out, K must have the unit of ohms x circular mils / feet
The equation is actually like this:
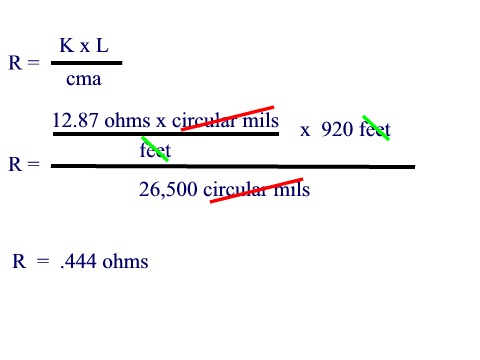
Notice how the FEET cancel out the FEET and the CIRCULAR MILS cancel out the CIRCULAR MILS leaving only OHMS.
This is how it really works. All proper equations involving physical quantities will work out like this. For real, they do.
One more non-electrical example:
How many feet is 179 centimeters?
Certainly a pesron can figure out how to do this and avoid the units in the equations all-together, but here is the proper strict method to do it:
We will make use of the equalities:
2.54 cm = 1 inch
12 inches = 1 foot
to convert cm to feet, we do this:
length (in feet) = 179cm x (1 inch/2.54cm) x (1 foot/12 inches)
length = 5.873 feet
notice how the units on the right side cancel out leaving only feet.
Units must always work out in a similar manner when doing math with physical quantities.
Okay, let's do a sample problem.
Say we have a copper wire that is 920 feet long and has a cross sectional area 0f 26,500 circular mils.
Now it is tempting to ignore the units and just use the numbers
K = 12.87
L = 920
cma = 26,500
certainly we can do the math and ignore the units and the numbers will work out correctly because someone else has already taken the trouble to build the formula so that it works out properly.
On that note, if all you want to do is crank out the numbers and you don't mind missing out on the inner beauty of physical quantities and the math, that is fine and you can stop reading here.
But if you want to understand physical quantities a bit deeper, keep reading.
For the copper wire, we will say
K = 12.87
L = 920
cma = 26500
Now, in reality, the numbers given above are not simply numbers. They are physical quantities. And physical quantities must be defined by units. And these units must be included in the formulas.
So from above,
L = 920 FEET
cma = 26,500 circular mils
and let us just assume for the sake of demonstration that
K = 12.87 ohms
R = K x L / cma
R = 12.87 ohms x 920 feet / 26,500 circular mils
so R = .444 ohms x feet / circular mils
The feet and circular mils do not just magically diassappear when you do the math. No, they have to be accounted for. And since R on the right side should be in Ohms, not in ohms x feet /circular mils, it becomes obvious that K must have units other than ohms.
For the units to work out, K must have the unit of ohms x circular mils / feet
The equation is actually like this:
Notice how the FEET cancel out the FEET and the CIRCULAR MILS cancel out the CIRCULAR MILS leaving only OHMS.
This is how it really works. All proper equations involving physical quantities will work out like this. For real, they do.
One more non-electrical example:
How many feet is 179 centimeters?
Certainly a pesron can figure out how to do this and avoid the units in the equations all-together, but here is the proper strict method to do it:
We will make use of the equalities:
2.54 cm = 1 inch
12 inches = 1 foot
to convert cm to feet, we do this:
length (in feet) = 179cm x (1 inch/2.54cm) x (1 foot/12 inches)
length = 5.873 feet
notice how the units on the right side cancel out leaving only feet.
Units must always work out in a similar manner when doing math with physical quantities.
physis
Senior Member
Re: is this right?
Yes Crossman.
The definition I reposted can be expressed mathmatically as K=((R/D)L)@T
R = Resistance
D = Diameter
L = Length
T = Temperature
That's K equals resistance per a diameter and multiplied by it's length at a given temperature.
Notice the quantity that gets operated on and who's resulting value becomes K.
Of course K represents parameters addition to resistance. I don't think anybody's missing that.
Yeah, you're a trouble maker Jwest.
Yes Crossman.
The definition I reposted can be expressed mathmatically as K=((R/D)L)@T
R = Resistance
D = Diameter
L = Length
T = Temperature
That's K equals resistance per a diameter and multiplied by it's length at a given temperature.
Notice the quantity that gets operated on and who's resulting value becomes K.
Of course K represents parameters addition to resistance. I don't think anybody's missing that.
Yeah, you're a trouble maker Jwest.
crossman
Senior Member
- Location
- Southeast Texas
Re: is this right?
Well all I did was make an innocent assertion that the unit for K is not Ohms. I am not taking the blame for this one! I blame it on Physis.
Well all I did was make an innocent assertion that the unit for K is not Ohms. I am not taking the blame for this one! I blame it on Physis.
crossman
Senior Member
- Location
- Southeast Texas
Re: is this right?
Well, it was a good discussion and hopefully there is someone out there who may have learned something from it. Thanks!
Well, it was a good discussion and hopefully there is someone out there who may have learned something from it.
Thanks!- Status