Law of Sines

Status
Not open for further replies.
physis

physis

Senior Member
I'm going to be facing a question on a test that requires the use of the law of sines and the law of cosines. Trouble is, I'm not able to make the law of sines work in all cases.

Is it my fault or is it the law of sines?

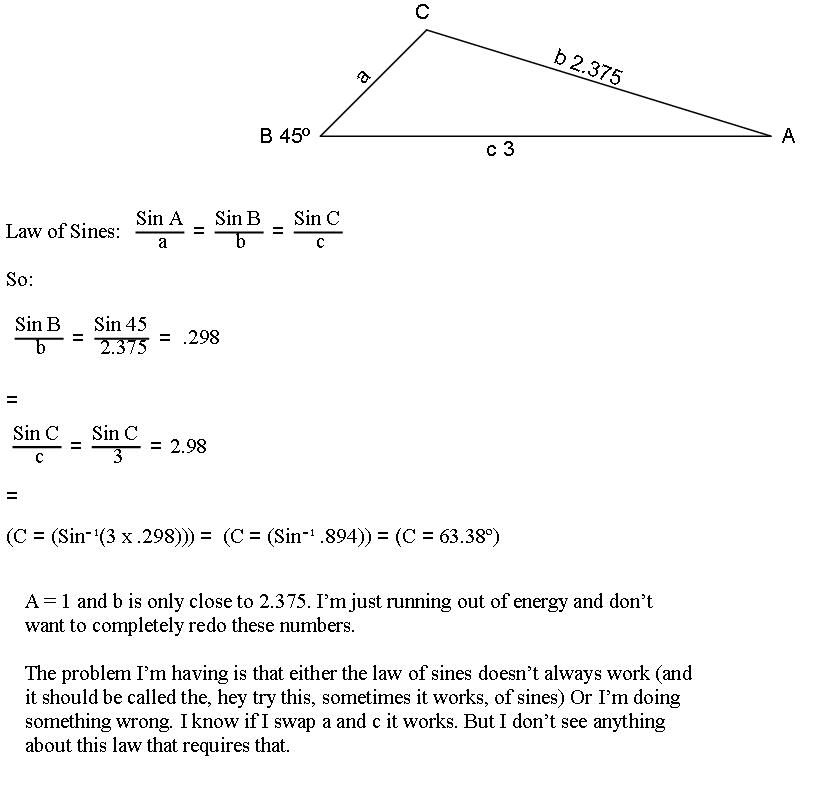
Lawofsines.jpg


By the way, I forgot to mention in the graphic that C is totally NOT 63.38?.

And a corrction. A = 1 should be a = 1.
 
Re: Law of Sines

Sam,

Be aware that arcsin 0.893 = 63.38 or 180 - 63.38 = 116.6!

Rattus

[ August 01, 2005, 04:28 PM: Message edited by: rattus ]
 
Re: Law of Sines

Part of the problem, it that the dimensions shown cannot represent a triangle; i.e., if a=1, either b is not 2.375 or A is not 45 degrees. As you are trying to compute it, b~2.399.

As long as there are no obtuse angles (those greater than 90) involved, the Law of Sines works quite well directly. When there are obtuse angles involved it is necessary to remember that the Sine function [Sin (X)] is a function only as it applies to an angle. Asin(X) is a "relationship," not a function;i.e., there is no unique angle for Asin(X). Sin 45 = Sin 135 = Sin 405 ...

So, C= 180 - Asin(Sin B *c/b)~117.8
 
Re: Law of Sines

The problem here is that you do not have a unique triangle. The Law of Sines requires that you have a triangle; you have two triangles, not one unique triangle.

I am going to start by ignoring your after-thought statement to the effect that a = 1 and that b = (close to) 2.375. I will start by looking at the problem as shown in the figure: a triangle having an unknown side "a," and two unknown angles "A" and "C."

Without doing any math, I quickly noted that this describes two triangles, and not one. I get that from the fact that when attempting to prove two triangles are congruent, you can use SSS, or SAS, or ASA, but not SSA. Your sketch is an SSA situation. You have two known sides, but the known angle is not between them. Therefore, you do not have a unique triangle.

You can construct the two triangles by laying out side "c" with a length of 3. You draw a ray (theoretically infinite in length), starting from the left hand side of "c," and going up at a 45 degree angle. Your side "a" will be somewhere along that ray. Finally, you start from the right hand side of "c," and draw a circle with radius 2.375. That circle will intersect the ray at two places. These will give you two triangles, both of which match your initial conditions.

I did the math (but do not have time now to check it), using the Law of Cosines: c^2 = a^2 + b^2 - 2ab(cos B). Using the known sides and the known angle, I found that side "a" can be either 1.058 or 3.189. If you look at the triangle that has "a" at 3.189, you will see that the angle "A" is 63.28 degrees. That is the solution that you obtained from the Law of Sines.
 
Re: Law of Sines

Ok.

Good, I'm not crazy.

I have noted that there are conditions associated with the use of the law of sines. However I can't find specifically what those rules are.

Can you guys clue me in on how to know exactly when I can't use the law of sines?

And Charlie, isn't cosines c^2 = a^2 + b^2 - 2ab(cos C) ?
 
Re: Law of Sines

Originally posted by physis:And Charlie, isn't cosines c^2 = a^2 + b^2 - 2ab(cos C) ?
Click to expand...
Yes. I was confusing the original statement of the Law with the known and unknown values in the sketch.
 
Re: Law of Sines

Didn't mean to pick on you Charlie, I'm just working on being clear on this so it's not possible to miss when I'm locked in a room somewhere and I can't ask you guys for help. :(
 
Re: Law of Sines

Originally posted by physis:I have noted that there are conditions associated with the use of the law of sines. However I can't find specifically what those rules are.
Click to expand...
There are no conditions. But you do have to take care when you use it. As Rattus and Rbalex have already pointed out, you must take care when you go from "Sin(C) = . . . " to "C = . . . ."

Given your sketch, and in particular given that C is an obtuse angle, I would go from "Sin (C) = 0.894" to "C = 117.8."
 
Re: Law of Sines

Well, I know that's the actual solution, and I know that the law of sines can sometimes yield two answers or worse. But how do I know when I can rely on the yielded solution? Or when I should question the results? :)
 
Re: Law of Sines

The day before yesterday you couldn't have convinced me that there could be anything difficult about this. :D

So Where Am I wrong?

The OP triangle doesn't comply. :confused:
 
Re: Law of Sines

Sam, the problem as stated has two equally valid solutions. One with an obtuse angle, and one with no obtuse angles.

rbalex,

sin(45) = 0.707 = sin(135)
sin(-45) = -0.707 = sin(-135)

But, I know that you know that!
 
Re: Law of Sines

The OP triangle does comply. You just have to recognize that it shows an obtuse angle, and look for the appropriate obtuse angle when you take the inverse sine.

Just mark it all down as "error M" (i.e., Monday). :D
 
Re: Law of Sines

Let me put this way.

Can I take 180? - 68.38? based on whether the angle is obtuse or acute?

That's an obvious distinction.

Is that all it amounts to?
 
Re: Law of Sines

Error M :D

You might end up regretting that Charlie. Edit: :D

[ August 01, 2005, 07:20 PM: Message edited by: physis ]
 
Re: Law of Sines

Originally posted by physis:
Let me put this way.

Can I take 180? - 68.38? based on whether the angle is obtuse or acute?

That's an obvious distinction.

Is that all it amounts to?
Click to expand...
Yep Sam, you have to decide which is the correct solution. Usually this is obvious--but not always.
 
Re: Law of Sines

I would go from "Sin (C) = 0.894" to "C = 117.8."
Click to expand...
Charlie, maybe there's a better technique I should be using, what method do you use to get to 117.8??
 
Re: Law of Sines

It's a matter of which set of numbers you use, and your tolerance for "round off error." In this case, I mixed two sets of numbers (i.e., those shown in the sketch, and Bob's correction to the lengths of the sides). I should have said:
I would go from "Sin (C) = 0.894" to "C = 116.62."
Click to expand...
The process, as others have described, is to take the inverse sine of 0.894, get an answer of 63.38, then observe that the angle is obtuse, and subtract 63.38 from 180, and get the "final answer" of 116.62 degrees.
 
Re: Law of Sines

I'm not checking your math Charlie. I trust you and we still know what value we're talking about. :cool: (The B and C was hard to miss)

So I guess I'm prepared then.

Thanks Charlie.
 
Status
Not open for further replies.
Top