basicbill
Member
- Location
- Western Canada
Hi,
I read through all 150 or so postings on the unbalanced neutral thread and I examined the xls calculators but I have a lingering question that I hope you all can answer.
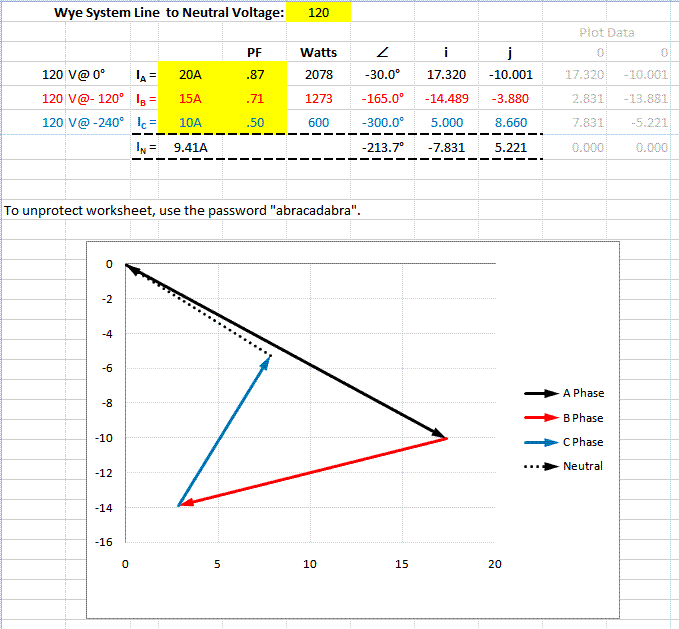
My scenario is this....120/208V, 3 phase 4 wire system. Three individual single phase motors, all at different pf's.
I plug the variables into the neutral calculator to get an answer.....no problem.
My question is this....why does the spreadsheet utilize the phase angles as leading the phase voltage? For instance if one of my motors has a pf of .707 on phase A, shouldn't the calculator use an angle of -45 degrees?
Thanks in advance!
BB
I read through all 150 or so postings on the unbalanced neutral thread and I examined the xls calculators but I have a lingering question that I hope you all can answer.
My scenario is this....120/208V, 3 phase 4 wire system. Three individual single phase motors, all at different pf's.
I plug the variables into the neutral calculator to get an answer.....no problem.
My question is this....why does the spreadsheet utilize the phase angles as leading the phase voltage? For instance if one of my motors has a pf of .707 on phase A, shouldn't the calculator use an angle of -45 degrees?
Thanks in advance!
BB