table 8

[kwired]

I think it is easier to think of circular mil area measurement as a ratio to the area of a one mil circle. See table below. Note the circular mil area is just the square of the diameter in mils. The resulting measure still covers the area of concern (the shape doesn't really need to be circular). In measuring multiple circular areas, such as stranded conductor crosssection, only the circular areas are measured. The gaps are not included.

mil diameter	area (in?)	circular mil area
1	0.000000785398	1
2	0.000003141593	4
3	0.000007068583	9
4	0.000012566371	16
5	0.000019634954	25
6	0.000028274334	36
7	0.000038484510	49
8	0.000050265482	64

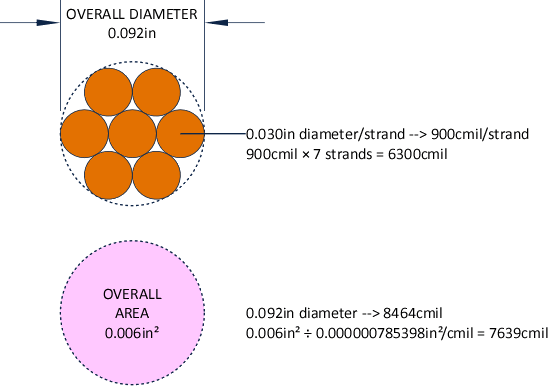
That makes the most sense to me. I was just blown away when looking at definition of what a circular mil is. The area of a circle that has a diameter of 1/1000 inches. Then got to thinking how would you stack a whole bunch of those inside another object without gaps and be able to determine the area of the larger object when you are not covering all of it? But just using a ratio to determine the area makes good sense to me. Everyone kept bringing up stranded conductors, and that is not what the question was or concerned, and besides we still determine the CSA of solid and compact conductors also, and they do end up being different diameters to get the same CSA of actual material composing the conductor.

 

[kimrichi]

I wasn't asking about stranded conductors. I was asking about the area of a circle in general.

If you are using square blocks as a measuring device you can lay one next to the other and fill all the area with no gaps between your measuring devices.

If you have 100 circles all the same size and lay them out in any dimension right next to each other you will have gaps within the area, how are these accounted for in the total circular mils of an object being measured?

Or if you were to assume several same sized circular objects as measuring gauges, does the amount of material cut off the gauges that shape the outer edge of the object being measured end up equaling the needed area to fill the remaining gaps inside the area being measured?

I am not that advanced of a math wiz, and just asking how you measure area with a base unit that can not be laid out with no gaps in between simple base units.

does not this mean that cir. mill for stranded conductor should be less than solid one and that is not true in the table [they are both same area]

 

[kwired]

does not this mean that cir. mill for stranded conductor should be less than solid one and that is not true in the table [they are both same area]

The idea is the same amount of copper will have same current carrying capability. A stranded conductor of same CSA as a solid conductor will have a larger overall diameter because not all the area is composed of copper, the gaps between the strands take up space that is not used for anything purposeful for the conductor, all that is counted in the CSA is the actual area composed of conductive material.

 

[ggunn]

Umm... I believe you have that backwards. The circular mil area of a stranded conductor is the sum of the circular mil area of the individual strands.

For example, a 12AWG copper conductor has the same amount of copper per length whether it is solid or stranded.

Then why does a 7 strand 12AWG conductor have a higher DC resistance than a solid one? They are both 6530 circular mils.

 

[Smart $]

Then why does a 7 strand 12AWG conductor have a higher DC resistance than a solid one? They are both 6530 circular mils.

At 75?C, the stranded version dissipates heat at a slightly slower rate, an effect caused by the [air] gaps between inner and outer strands. Air in the gaps has a substantially lower thermal conductivity than copper, and there are no gaps in the solid version.

 

[ggunn]

At 75?C, the stranded version dissipates heat at a slightly slower rate, an effect caused by the [air] gaps between inner and outer strands. Air in the gaps has a substantially lower thermal conductivity than copper, and there are no gaps in the solid version.

Do you know this from an accredited source or is it just something you have reasoned out? It seems to me that effect would be current dependent (the more the current, the more the heat generated, and the more the difference in resistance would be). I don't see anything in the table notes that says anything about current; what if it were 1 microamp? Note 1 says, "These resistance values are valid only [emphasis theirs] for the parameters as given." Current is not given, but temperature is (75 degrees C), which I take to mean homogeneous conductor temperature, however it got to be 75 degrees.

Additionally, Note 5 says (referring to stranding), " Its overall diameter and area [emphasis mine] is that is that of its circumscribing circle."

 

[Smart $]

Do you know this from an accredited source or is it just something you have reasoned out?

I'll say "reasoned out"... just to make my life easier

It seems to me that effect would be current dependent (the more the current, the more the heat generated, and the more the difference in resistance would be). I don't see anything in the table notes that says anything about current; what if it were 1 microamp? Note 1 says, "These resistance values are valid only [emphasis theirs] for the parameters as given." Current is not given, but temperature is (75 degrees C), which I take to mean homogeneous conductor temperature, however it got to be 75 degrees.

Additionally, Note 5 says (referring to stranding), " Its overall diameter and area [emphasis mine] is that is that of its circumscribing circle."

The effect is current dependent... but as you so noted, it is relevant to the conductor temperature... regardless of how it got to that temperature. A current of one micro-ampere is not going to take the conductor, stranded or solid, to 75?C when operating in 30?C ambient air. That's also why Note 2 has the equation for temperature change. Though not listed, compact conductors would have a resistance value closer to its solid counterpart.

 

[ggunn]

I'll say "reasoned out"... just to make my life easier



The effect is current dependent... but as you so noted, it is relevant to the conductor temperature... regardless of how it got to that temperature. A current of one micro-ampere is not going to take the conductor, stranded or solid, to 75?C when operating in 30?C ambient air. That's also why Note 2 has the equation for temperature change. Though not listed, compact conductors would have a resistance value closer to its solid counterpart.

Then, my opinion is that the reason for the higher resistance for stranded wire is that the total cross section of copper is lower because of those air gaps, not because of anything related to heat transfer. Note 5 clearly states that the area of stranded wire is that of its circumscribing circle. As you say, the resistance of a compacted conductor would be somewhere in between that of regular stranded and solid wire because more of its cross section is filled with copper.

 

[ggunn]

The areas of each individual strand in a multi-stranded conductor will collectively add up to a smaller number than the circular mil area of the conductor as a whole, for the very reason you name. The circular mill area of the conductor is not the sum of the areas of the strands, but rather the area of the smallest circle that can completely contain the entire conductor.

Correct, as Note 5 under Table 8 clearly states, "[Class B stranding] overall diameter and area is that of its circumscribing circle."

 

[Smart $]

Then, my opinion is that the reason for the higher resistance for stranded wire is that the total cross section of copper is lower because of those air gaps, not because of anything related to heat transfer. Note 5 clearly states that the area of stranded wire is that of its circumscribing circle. As you say, the resistance of a compacted conductor would be somewhere in between that of regular stranded and solid wire because more of its cross section is filled with copper.

BUT the toal cross-sectional area of the copper is [essentially] the same. For #12 stranded...

Note the sum of the seven strands' cross-sectional areas is closest to the 6530cmil table value. The difference is mostly due to rounding errors, some is due to allowable tolerance. If you back-figure 6530 to a diameter of seven strands, in inches...

SQRT(6530/7) = 30.543mils or 0.030543in

​

The table value is only 0.030in.

 

[ggunn]

BUT the toal cross-sectional area of the copper is [essentially] the same. For #12 stranded...

Note the sum of the seven strands' cross-sectional areas is closest to the 6530cmil table value. The difference is mostly due to rounding errors, some is due to allowable tolerance. If you back-figure 6530 to a diameter of seven strands, in inches...

SQRT(6530/7) = 30.543mils or 0.030543in

​

The table value is only 0.030in.

So how do you account for Note 5?

 

[Smart $]

So how do you account for Note 5?

What's to account for??? The overall diameter and circumscribed area is greater compared to that of solid... but it includes "air".

 

[ggunn]

BUT the toal cross-sectional area of the copper is [essentially] the same. For #12 stranded...

Note the sum of the seven strands' cross-sectional areas is closest to the 6530cmil table value. The difference is mostly due to rounding errors, some is due to allowable tolerance. If you back-figure 6530 to a diameter of seven strands, in inches...

SQRT(6530/7) = 30.543mils or 0.030543in

​

The table value is only 0.030in.

What's to account for??? The overall diameter and circumscribed area is greater compared to that of solid... but it includes "air".

Interesting. My error has been applying Note 5 to "Area" instead of "Overall Area", which is also clearly stated in the note.

By inspection from your diagram, clearly the diameter of a single strand is 1/3 the diameter of the circumscribed circle. If we let A1 be the area of the circumscribed circle and A2 be the combined area of the strands and do the algebra (r=d/2, A=(pi)*r^2), then A1 = (9/7)*A2.

If we look at column 9 on Table 8 (Conductors Overall Area in mm^2) for AWG 8, we see two numbers, one for solid (8.37) and one for stranded (10.76). 10.76 is indeed 9/7 times 8.37. I was under the mistaken impression that the diameter of a solid conductor and that of a stranded conductor of the same AWG were the same (all you electricians stop laughing at the ignorant engineer), but I see now that the overall area of a stranded conductor is 9/7 that of a solid conductor of the same gauge in order to compensate for the gaps.

All of which does not explain the approximately 2% higher DC resistance of stranded conductors over that of solid conductors, but I am not yet ready to concede that it is because of heat transfer because there is no mention of current in the governing parameters of the table and that difference would vary with the current. If the Table stated that the 75 degree conductor temperature was due to the conductor carrying whatever current is necessary to raise it to that temperature over some ambient, so that in a stranded wire the center conductor would have higher resistance due to it being hotter, in turn due to the lower heat transfer happening in the six air gaps around it, then OK, but it doesn't say that. But of course, that may be how the measurements were made...

 

[Smart $]

...

All of which does not explain the approximately 2% higher DC resistance of stranded conductors over that of solid conductors, but I am not yet ready to concede that it is because of heat transfer because there is no mention of current in the governing parameters of the table and that difference would vary with the current. If the Table stated that the 75 degree conductor temperature was due to the conductor carrying whatever current is necessary to raise it to that temperature over some ambient, so that in a stranded wire the center conductor would have higher resistance due to it being hotter, in turn due to the lower heat transfer happening in the six air gaps around it, then OK, but it doesn't say that. But of course, that may be how the measurements were made...

The Informational Note says the resistance is calculated in accordance with National Bureau of Standards Handbook 100, dated 1966, and Handbook 109, dated 1972. Can't find an online copy. Perhaps they calculated the resistance of one strand, then calculated parallel resistance...