Re: Transformer Primary Impedance
OK Ronald,
Imagine a toroid (donut shape) made of powdered iron. The toroid is the classic shape used in deriving magnetic formulas. Now wind a few turns of wire through the hole and call this the primary winding. We now have an inductor, and let us imagine that it is an ideal inductor?no resistance and no leakage flux, i.e., all magnetic flux lines are contained in the iron core. Also assume that the magnetic properties are linear, i.e., no hysteresis.
Here is a transformer made with a square donut.
Now apply a sinusoidal voltage. This creates a sinusoidal magnetizing current which creates a sinusoidal magnetic flux in the core which induces a ?back emf? in the primary equal and opposite to the applied voltage. This limits the magnetizing current.
Let me emphasize that the magnetic flux "flows" around the inside of the toroid much like an electrical current. There is, in this ideal case, no flux outside the iron!
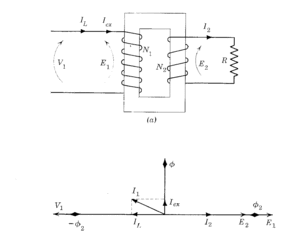
Now wind a separate winding through the donut and call it the secondary. The changing magnetic flux generated by the magnetizing current induces an emf in the secondary which is equal to:
E2 = E1 x N2/N1
Now apply a load to the secondary. The resulting secondary current will try to create additional magnetic flux in the core, but the primary current will increase as well and its magnetic flux will exactly cancel that of the secondary. The result is that the magnetizing current remains constant under all load conditions.
Let me also emphasize that, in this ideal case, both the primary and secondary windings see exactly the same magnetic flux created by the magnetizing current in the primary winding.
Now I cannot explain the unseen mechanism that makes all this work. It is like explaining gravity. It just happens.
Of course a real transformer behaves a bit differently, but the ideal transformer is quite useful in understanding the basics. When all this sinks in, I will post the equivalent circuit of a real transformer.
[ August 26, 2005, 06:21 PM: Message edited by: rattus ]