Smart $ said:Ummm... if you would be so kind as to give an expanation of the vectors in your diagram. As far as I can tell, they do not correlate to anything in the post you quoted.
Smart, he has scaled the drawings to 1A/inch.
Smart $ said:Ummm... if you would be so kind as to give an expanation of the vectors in your diagram. As far as I can tell, they do not correlate to anything in the post you quoted.
It's because you created the unbalance by shifting the neutral instead of extending the phases. You end up with angles between phases that are not 120?, which is a necessary condition for the sqrt(A?+B?+C?-A?B-B?C-A?C) equation to work.weressl said:Could somebody untagle why the vector solution gives 1.79333A
I assumed that. However, it didn't sink in what Laszlo was attempting to do until I revisited his diagram just now. He's plotting the equidistant point from the ends of the three vectors.rattus said:Smart, he has scaled the drawings to 1A/inch.
As mentioned in my response to rattus, I gather you are plotting the equidistant point from the end of the three vectors. It don't work :wink:weressl said:Could somebody untagle why the vector solution gives 1.79333A
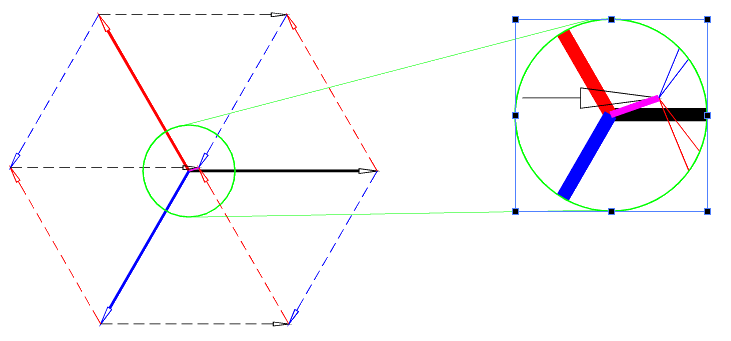
I did that, but at the same time showed it can be done six different ways!!!rattus said:Smart, I give you credit for figuring out what Laszlo was doing, but you are making this problem unbelievably complicated. All you need do is draw out the three phasors, head to tail, like a delta, then close the diagram with a fourth phasor which is In.
I have no idea where you are getting "the phasor sum of 1.732A @ 120 and 2A @ 0".But, this is a poor way to solve the problem because in this almost balanced system the neutral phasor is so short it is hard to measure. If you use graphics, you should reduce the problem as I have done. Then, you have the phasor sum of 1.732A @ 120 and 2A @ 0. The phase angle is clearly in the first quadrant. It is not 199 degrees.
To simply state IN as "the sum is the negative of In" does not prove such. I offered simple proof. Rejection of said proof is your prerogative, just like it is my prerogative to respectfully disagree regarding its correctness.rattus said:...You actually found the negative of In and took the liberty of negating it again to get the right phase angle.
"The sum is 2.5 + j0.866 and represents the point at the tail end of neutral vector. The head end of neutral vector is at 0 + j0."
This gets the right answer, but strictly speaking is incorrect. You should have said the sum is the negative of In and then negated it.
Same here.I believe though that we agree that the sum of ALL currents either into or out of the neutral node is zero. That is, In is that current which will satisfy Kirchoff's current law. We could have defined In as the sum of the phase currents. I am unaware of any rule or convention though. How about you?
Smart $ said:To simply state IN as "the sum is the negative of In" does not prove such. I offered simple proof. Rejection of said proof is your prerogative, just like it is my prerogative to respectfully disagree regarding its correctness.
Same here.
I'm OK with the above, as long as it is [mentioned and...] understood that your last formula is regarding the phasor relationship and not a simple algebraic equation. Without noting and comprehending such, someone could come along and calculate the problem as In = -(Ia + Ib + Ic) = -49A - 47A - 46A = -44A, which all us knowledgeable types know to be an incorrect result.rattus said:Smart, we agree on the result, right? I am simply saying that,
2.5 +j0.866 = 2.65A @ 19.1 = -In
Negate that phasor to obtain,
In = 2.65A @ 199.1
In other words, after applying Kirchoff's current law,
In = -(Ia + Ib + Ic)
I approached the problem by finding the the sum of the currents necessary for balance. Then I didn't have to negate.
Seems to me we're both coming up a little short on complete explanations :grin:You slipped the negation in without fully explaining it.
In my mind it wouldn't :wink:Your phasor diagram would look funny with the arrowhead at the origin.
I'm not sure exactly what it is you want me to diagram.rattus said:Smart, I see where you are coming from now. How about one of your nice diagrams to help us visualize the method?
Smart $ said:Anyway, rattus is wrong about the phase angle of the resulting neutral current vector?which is 2.65A @ 199.1?.
rattus said:Smart, I see where you are coming from now. How about one of your nice diagrams to help us visualize the method?
I didn't trick you... Laszlo did with his diagramrattus said:Smart, you tricked me. I was responding to Laszo's question where,
Ia = 46A
Ib = 47A
Ic = 49A
2.65A @ 40.9 degrees is correct.
You defined the currents as,
Ia = 49A
Ib = 47A
Ic = 46A
Magnitude doesn't change; angle does.
Gary Shumaker said:...For example, if phase A = 49A and phase B = 47A and phase C = 46A...
View attachment 1211rattus said:Just give us a phasor diagram of the 3A, 2A, 2.65A closed polygon. I could do it, but your diagrams are prettier.
Picture worth a kiloword!
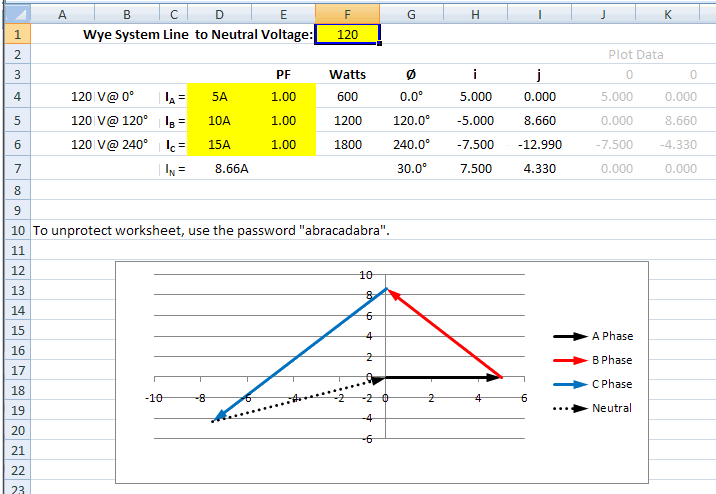
None. Calculations are performed by regular excel formulas.cschmid said:with all the add ons for Excel what one are you using for the math you are doing?
