Why is residential wiring known as single phase?

[jim dungar]

Why retract it when I can prove it?

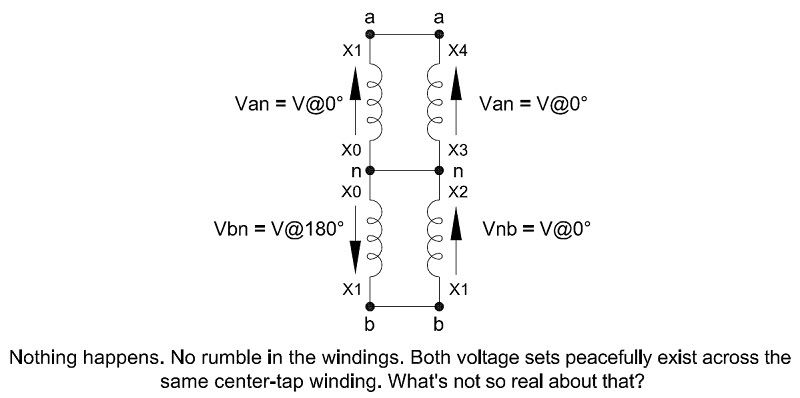
Finally, graphical proof that Vbn is in-phase with Vnb.

 

[mivey]

You would be the member that's posted about coal cars. And when I've mentioned trains it's been about current and the induced field. So from the above you've stated that we should not consider you a competent engineer.


Yea, I think I've mentioned electrons wiggling back and forth like they were part of a train. But the coal cars, and the "picking up" and "transporting" are all you Mivey.

Again, it would help if you could keep up with the string of posts you are involved in. You stated the power flows back and forth in different directions:

During the first half cycle the power flows from A to B and therefore the voltage gradient is from A to B in proportion to the power flow. During the second half cycle the flow is from B to A

I pointed out that you were in error because power is always directed towards the load:

The energy flows along both wires towards the load. It travels in the electromagetic field surrounding the wires, not riding in the wires like a line of coal cars traveling back and forth.

Because you lack understanding of how electricity works and that there is a difference in current and energy flow, you come up with the crazy idea that I said current was flowing outside the wire.

Are you gonna tell me you think the current travels OUTSIDE the copper as Mivey stated?
...
The copper acts as a wave guide channeling the current through the electromagnetic field surrounding the wires. (per Mivey)

When I said energy travels in the electromagnetic field surrounding the wire, you disagreed:

LoL, but a resounding NO. AC travels inside the copper to a depth determined by frequency.

I say the energy flows outside the wire in the electromagnetic field. That is all me.

The notion that saying energy travels outside the wire is the same as saying the current is outside the wire is all you.

The idea that energy is moving back and forth with the current is all you.

The idea that energy travels inside the wire is all you.

The lack of knowledge is all you.

 

[mivey]

Finally, graphical proof that Vbn is in-phase with Vnb.

Hardly. But if you want to ignore what the subscripts mean, I guess you can read it however you want.

 

[Rick Christopherson]

Why retract it when I can prove it?

Prove it? You didn't even read the question, did you? How does that example prove whether the phase shift is real or apparent? Go back and reread the posting. I'm not arguing whether the two waveforms are in-phase or not. I'm arguing about your comments that your phase shift is not just an apparent phase shift but a real phase shift.

 

[mivey]

Prove it? You didn't even read the question, did you? How does that example prove whether the phase shift is real or apparent? Go back and reread the posting. I'm not arguing whether the two waveforms are in-phase or not. I'm arguing about your comments that your phase shift is not just an apparent phase shift but a real phase shift.

You have both a 0? voltage and a 180? voltage occupying the same space. One voltage from the left comes from a phase-shifted voltage and is positive in the down direction. The other voltage from the right comes from a non-phase shifted voltage and is positive in the up direction.

What do you consider the significance of whether one is phase shifted or not? Do the physics change because we remove the voltage on the left? Do the physics change because we remove the voltage on the right?

No physics change. Both voltages are present in either case because they were there all along. Bringing in the voltage from the right or bringing in the voltage from the left only makes it obvious that both are there.

You have both a 0? voltage and a 180? voltage occupying the same space. Can't you see that both voltages were there all along, and that debating the existence of what you are calling a phase-shift does not change the fact that both a 0? voltage and a 180? voltage occupy the same space?

 

[gar]

120302-1055 EST

Rick:

For a "real phase shift" to occur do you require that it come from a device that produces a time delay, such as a delay line, or a split light or audio beam that travels two different distances?

If the answer is yes above, then how do you describe the phase shift from a resolver? I think the resolver and LVDT come closest to being equivalent to the center tapped secondary in that on an instantaneous basis the phase shift occurs without the assumption of a continuous wave.

.

 

[jim dungar]

Hardly. But if you want to ignore what the subscripts mean, I guess you can read it however you want.

Besoeker already stated that 'uneventful' paralleling of two windings can only be possible when the two windings are in-phase. Your graphic shows Vbn and Vnb in parallel.

 

[gar]

120302-1106 EST

Jim:

One of the voltages is labeled with @180. V_NB = V@0 then equals V_BN when V_BN is defined as V@180.

.

 

[mivey]

Besoeker already stated that 'uneventful' paralleling of two windings can only be possible when the two windings are in-phase.

You and I both know that a winding does not have a phase on its own. In this case, when we talk about the phase we are talking about the phase of a voltage waveform.

Your graphic shows Vbn and Vnb in parallel.

You and I both know that the positive peak of the Vbn waveform from the left is in phase with the negative peak of the Vnb waveform from the right.

 

[jim dungar]

120302-1106 EST

Jim:

One of the voltages is labeled with @180. V_NB = V@0 then equals V_BN when V_BN is defined as V@180.

.

How many times have I shouted?

Vbn=-Vbn


Major oops and an apology for editing a previous post:
Of course I meant i have been saying:

Vbn =-Vnb

 

[rbalex]

120302-1106 EST

Jim:

One of the voltages is labeled with @180. V_NB = V@0 then equals V_BN when V_BN is defined as V@180.

.

AHHH - So it's labeling that permits the Tribe to screw with the sign, but V sin (wt + 180?) = - V sin(wt) {or V *-sin(wt)} is invalid?

The function for both sides at t = 0 is -V, and for any other time t, the functions are identical since that’s how identities work. The phase for both sides is identically -sin (wt).

 

[rattus]

Finally, graphical proof that Vbn is in-phase with Vnb.

No Jim, even rbalex agrees that inverses cannot be in phase. It just shows that one expression is just as real as the other.

 

[rattus]

AHHH - So it's labeling that permits the Tribe to screw with the sign, but V sin (wt + 180?) = - V sin(wt) is invalid?

The phase for both sides at t = 0 is 1, and for any other time t, the phase are identical since that?s how identities work.

No, not at all. The identity is true, but the minus sign merely shifts the function by PI. We have known that since day one.

Just plot -sin(wt). The angular position is still (wt + PI). Same plot as sin(wt + PI). No change at all.

This discussion could be ended easily if someone would just provide a valid reference.

 

[Rick Christopherson]

What do you consider the significance of whether one is phase shifted or not?

The issue is that you emphatically stated that it was not an apparent phase shift, but a real phase shift: That it was not mathematical, but what is really there. As a refresher for what you said, here are your quotes again:

It isn't mathematically represented.
It's what happens in real life.
It's real.

So it is a physical fact, not just a mathematical equivalent, that we have 0? voltages and 180? voltages across the windings. The difference is which reference frame you use, but both are physical realities.

In other words, we have both in-phase voltages and phase-opposed voltages at the transformer.

No, we are not saying N must be used. We are saying that it is convenient and logical to do so, then V1n and V2n are as Bes says antiphase. They don't just appear to be so, they are. After all, V1n and V2n are what is seen on the bus bars in a residential panel.

If the phase shift is real, then the output will not be inverted, but shifted by 1/2T (half period). If the phase shift is apparent, then the output will be inverted, and not shifted.

To make the expressions easier to write, I assumed a unity, ideal transformer. The voltage functions are nondescript v(t)'s of period T. v_x(t) is a small signal positive function of period T and superimposed on the primary voltage function.

Therefore, if the phase shift is real, as you claim, then v_x(t) will appear in the positive half-cycle of the output and shifted by 1/2T. If the phase shift is apparent, as we all know, then v_x(t) will appear in the negative half-cycle and not shifted.

 

[rbalex]

No, not at all. The identity is true, but the minus sign merely shifts the function by PI. We have known that since day one.

Just plot -sin(wt). The angular position is still (wt + PI). Same plot as sin(wt + PI). No change at all.

This discussion could be ended easily if someone would just provide a valid reference.

Sorry, I cheated again; I edited after the fact, but the phase for every voltage function is identical.

 

[rbalex]

No, not at all. The identity is true, but the minus sign merely shifts the function by PI. We have known that since day one. No actually you didn't, because t₀ must be the same for all functions and there is no "phase shift" with regard to the start of the period.

Just plot -sin(wt). The angular position is still (wt + PI). Same plot as sin(wt + PI). No change at all. YES - the phase is identical throughout the "real" period 0 - 360^o for both wt and wt + 180^o

This discussion could be ended easily if someone would just provide a valid reference.

Look at your college Trig book on, identities and substitutions.

 

[rattus]

Where's the beef?

Where's the beef?

Look at your college Trig book on, identities and substitutions.

Why? I already know them. Doesn't define phase either.

The phase of -sin(wt) is [wt + 180], the angular position of the wave. It's that simple. According to you, every wave would have the same angular position. Clearly not the case.

If you would just provide a valid reference, we might believe you. If you can't we must assume you are just snowing us.

 

[rattus]

The issue is that you emphatically stated that it was not an apparent phase shift, but a real phase shift: That it was not mathematical, but what is really there. As a refresher for what you said, here are your quotes again:







If the phase shift is real, then the output will not be inverted, but shifted by 1/2T (half period). If the phase shift is apparent, then the output will be inverted, and not shifted.

To make the expressions easier to write, I assumed a unity, ideal transformer. The voltage functions are nondescript v(t)'s of period T. v_x(t) is a small signal positive function of period T and superimposed on the primary voltage function.

Therefore, if the phase shift is real, as you claim, then v_x(t) will appear in the positive half-cycle of the output and shifted by 1/2T. If the phase shift is apparent, as we all know, then v_x(t) will appear in the negative half-cycle and not shifted.

View attachment 6545

Reference? Even if we do call it apparent, it matters not.

 

[Rick Christopherson]

Reference? Even if we do call it apparent, it matters not.

The why have you habitually called it "Real" for the past several years that this discussion has taken place on this forum across multiple threads? Why have you refused to retract the statement when it was brought to your attention?

The answer is simple: it is not something trivial. As much as you try to claim it is trivial, it is quite important, otherwise you would have no problem backing away from your past statements.

If it is so trivial and doesn't matter to this discussion, then simply retract the statements and come out and say the phase shift is apparent and mathematical, but not real.

 

[rbalex]

Look at your college Trig book on, identities and substitutions.

This was the defintion you supplied:

Phase: Phase is the fractional part of a period through which time or the associated time angle wt has advanced from an arbitrary reference

[Kerchner and Corcoran, Alternating-Current Circuits, Wiley, 1951]

You do understand the conjunction or does not mean both, right? Nevertheless, assuming t₀ is the same for all functions and the periods are identical, (and they better be for a conventional 120/240V system) then the "fractional part of a period through which time or the associated time angle wt has advanced from an arbitrary reference" is identical for all phases. If "the associated time angle" (not the associated phase angle) ever has a negative value, you're up for a Nobel Prize in physics for discovering how to make time run backwards.