Geometry question

Status
Not open for further replies.
D

darekelec

Senior Member
Location
nyc
Hello.
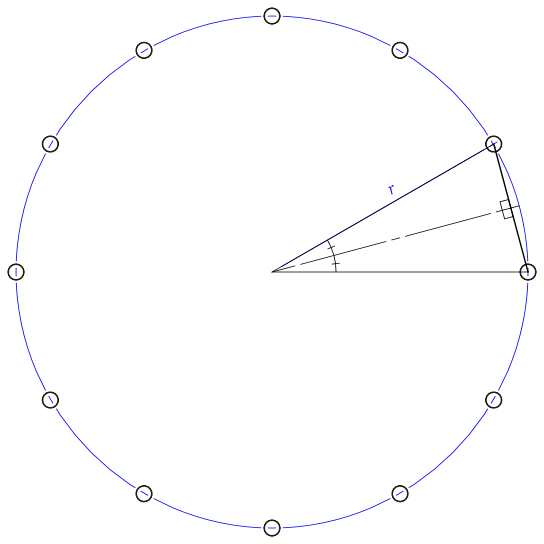
I have a situation where I have to lay out 12 hi heads in a shape of a circle.
Is it possible to come up with equation for me to know a distance between light fixture to the adjacent light fixture in straight line?
My field situation is that i need this dimension.
I would be able to come up with that when i was 12 years when i had geometry classes old but now can't figure it out having 1500$ worth of lasers. :). its so funny.
thank you in advance for your input
 
Smart $ said:
Radius times 0.51763809
Click to expand...
Agreed. You have an Isosceles triangle, with angles of 30, 75, and 75 degrees. From the Law of Sines, x/sin(x) = Y/sin(y), you obtain the length you want by multiplying the radius of the circle times (sin(30 degrees)/sin(75 degrees)). That is where Smart's formula comes from.

 
charlie b said:
Agreed. You have an Isosceles triangle, with angles of 30, 75, and 75 degrees. From the Law of Sines, x/sin(x) = Y/sin(y), you obtain the length you want by multiplying the radius of the circle times (sin(30 degrees)/sin(75 degrees)). That is where Smart's formula comes from.
Click to expand...
OK
Mine was for the distance round the ring rather than the linear distance.
 
The formula we used provided by 16 year old guy is
2r sin (c/2)
where c is angle in degrees of triangle at center of circle - 30 in my case

it it worked out well

thank you for replies
 
charlie b said:
That will give you the length of the arc between two lights. The question asked for the straight line distance between any two lights.
Click to expand...
Well, if if you laid the fixture out in a straight line, that would be the distance............:p
 
darekelec said:
The formula we used provided by 16 year old guy is
2r sin (c/2)
where c is angle in degrees of triangle at center of circle - 30 in my case

it it worked out well

thank you for replies
Click to expand...
2*sin(30?/2) = 0.5176380902050415246977976752481
 
darekelec said:
The formula we used provided by 16 year old guy is 2r sin (c/2) where c is angle in degrees of triangle at center of circle - 30 in my case
Click to expand...
I did a quick check of angles 10 through 100 degrees, in steps of 10 degrees, and the answers came out very close in all cases. I would have to do some refresher training on trig identities, in order to prove that it will always work. For anyone who wishes to take this on, what you would need to confirm is that 2r sin (c/2) is the same as (r)(sin(c)/sin((180-c)/2)).

 
Last edited:
No. Since the second expression does not have r in it, no they are not the same.
As for the trig identity, yes it is correct and can be derived from the half and double angle formulas.
Or you can just take the fact that both derivations are correct as proof of the identity.


Tapatalk!
 
Last edited:
charlie b said:
I did a quick check of angles 10 through 100 degrees, in steps of 10 degrees, and the answers came out very close in all cases. I would have to do some refresher training on trig identities, in order to prove that it will always work. For anyone who wishes to take this on, what you would need to confirm is that 2r sin (c/2) is the same as sin(c)/sin((180-C)/2).
Click to expand...
Don't believe that's necessary...

dodecagon.gif


At 180?, the triangle formed by two radials and the chord will not exist. All angles less than 180?, bisected, result in two equal angles of less than 90?. Therefore r ? sin(c/2) equals one half the subtending chord's length.
 
Last edited:
Smart $ said:
Don't believe that's necessary...
Click to expand...
But it is fun.

Start with the ?angle difference identity.?
(r)sin(c)/sin((180-c)/2) = (r)sin(c) / sin(90-c/2)
= (r)sin(c) / sin(90)cos(c/2) ? cos(90)sin(c/2)
= (r)sin(c) / (1)cos(c/2) ? (0)sin(c/2)
= (r)sin(c) / cos(c/2)

Now (temporarily) switch variable names.
Let X = c/2, so that c = 2X
That brings us to,
= (r)sin(2X) / cos(X)

Now apply the double angle identity
= (r){2sin(X)cos(x)} / cos(X)
= (r)2sin(X)

Finally, substitute back for X = c/2,
= (r)2sin(c/2)

Therefore, (r)sin(c)/sin((180-c)/2) = (r)2sin(c/2)
Q.E.D.

Or I could have taken the easier route, by looking at the Geometry section of the Engineering Mathematics Handbook I have at my desk. It states the same thing.

 
Smart $ said:
Radius times 0.51763809
Click to expand...

For some reason I've always found decimal approximations "inelegant," so I racked my brain trying to remember trig identities and what not, and determined that the "elegant" answer is:

Distance = Radius * sqrt(2) / (1 + sqrt(3))

Of course you'll end up putting it all in a calculator anyway, so might as well go with Smart $'s answer, but yeah, I'm feeling kinda proud of myself all the same for brushing up on high-school trig. :cool:
 
sinforoso said:
For some reason I've always found decimal approximations "inelegant," so I racked my brain trying to remember trig identities and what not, and determined that the "elegant" answer is:

Distance = Radius * sqrt(2) / (1 + sqrt(3))

Of course you'll end up putting it all in a calculator anyway, so might as well go with Smart $'s answer, but yeah, I'm feeling kinda proud of myself all the same for brushing up on high-school trig. :cool:
Click to expand...
Equivalent to, and perhaps slightly more "elegant" in some circles...

= 2r / (sqrt(2)+sqrt(6))

:D
 
Status
Not open for further replies.
Top