I know.And what do we Yanks designate that system per NEC/IEEE?
I was just making a point
I know.And what do we Yanks designate that system per NEC/IEEE?
I know.
I was just making a point

Well there are times where we do use a center tapped transformer to “split phases”. We are looking to have, on one secondary winding, the original waveform, and on the other one the same exact waveform with polarity inversion. We certainly don’t want something displaced in time by 180 degrees. Doesn’t matter that this isn’t electrical distribution as transformers don’t know and obey the same laws regardless.
Sometimes we do it the other way a round, a center tapped primary, AC grounded by cap with high voltage DC on it to feed two AC generators. It follows the “AC generators” are driven by previous center tapped transformer and operating in anti-phase/polarity. Under ideal conditions no AC is returned thorough the cap on center tap and DC currents are equal and opposite so core isn’t saturated.
Now what happens if one AC generator is “cut off”? What impedance does the remaining AC generator now see? Assume this generator is current limited and its transfer function is highly load dependent.
Now moving back to the discussion at hand, what impedance does the source see if we only have a L1 load on our residential electrical service? Would it be different if said supply was 120/208 secondary?
I agree with you on this 100% in the observation/measurement sense.For a single frequency sine wave there is absolutely no difference between inversion and a 180 degree displacement in time. None. Zilch. They are the same.
And go in opposite directions.The fact is that both observed sine waves begin simultaneously.
And go in opposite directions.
In the "causation" sense, it's the former; it's polarity, plain and simple.
The fact is that both observed sine waves begin simultaneously.
I agree that in the case of a single phase transformer the _mechanism_ is inversion, and that when (for example) the transformer is energized you would see the inversion rather than the time delay associated with a 180 degree 'shift'.
As soon as we are talking about energizing the transformer, however, we are no longer talking about single frequency sine waves.
So when we are doing our analysis based on the single frequency sine wave approximation, it makes perfect sense to use 0 and 180 degrees to talk about the two outputs.
Still a single phase transformer, still using inversion internally, and still _two_ phase angles for the purpose of analyzing the output.
Your opinion is noted........Which makes it the 180 degree output of the _same_ phase *grin*
-Jon
Three phase doesn't have a time delay either. No matter what the setup, a 'shift' in 'degrees' never actually means a delayed start for 2nd or 3rd phases in the real world. It's all just mathematical representation that allows us to do some types of engineering as long as real world waveforms resemble ideal sine waves within acceptable tolerances.
If the 120/240 service was the only kind of electrical service that existed, and if power factor and three-phase power weren't things needing to be analyzed, no one would ever have bothered to make phasor math part of electrical engineering education. I know that's a lot of 'ifs', but the point is, inversion really is all the math you need to analyze this service with resistive loads. It doesn't 'make sense' to use phasor math if a much simpler mathematical operation produces the same result that meets your needs. If you were programming software, for example,I believe you'd need a lot less computing power using inversion. (Not very important with today's devices, but I remember the TI-88.)
Now, I totally respect the position that says 'well, I'm trained to do phasor math for all these other applications so it's just natural for me to employ it here as well.' But there is nothing ontologically privileged about phasor math. It doesn't represent what the waveforms 'are'. The 'time delay' discussion pretty much proves that.
Mind you, I think we're on the same page here. I'm just quibbling with how your putting it. I'm 'just sayin'.
Winnie (and others), the circuits I were describing are tube based audio. Some may feel they aren’t relevant but a transformer doesn’t know that and acts the same regardless. I think it is easier to see the 180 vs -1 point when considering music. There you have no pure sine waves and R,C, and L are always in play.
Hmmmm.
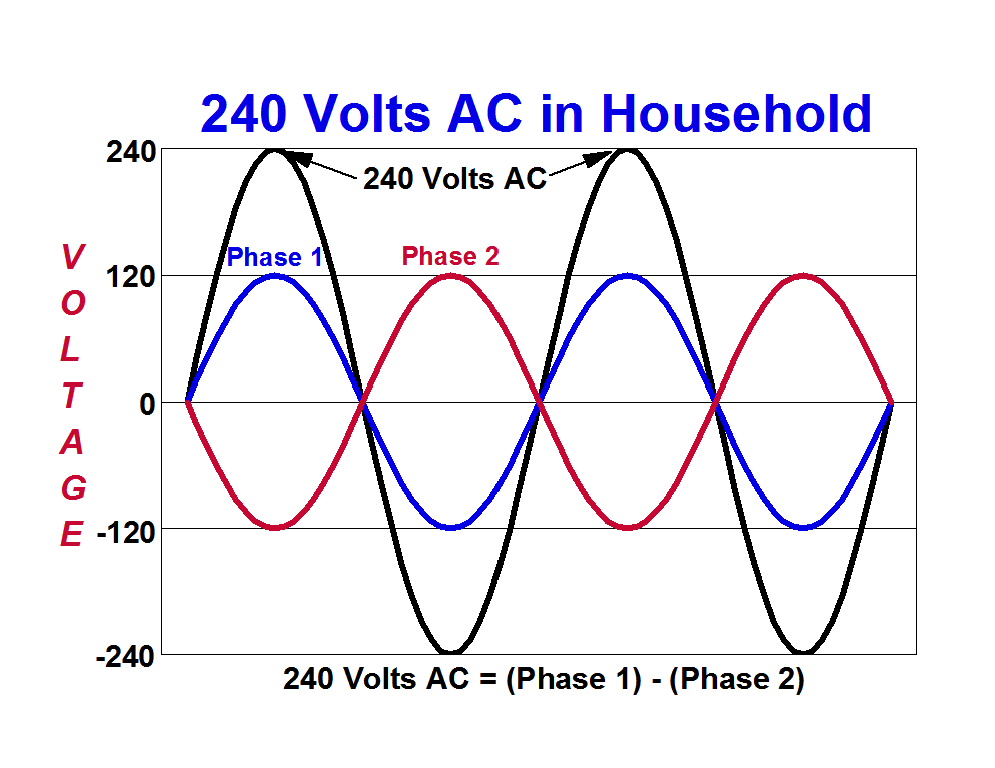

Both are types of common single phase services.
Math works both set ups. Two signals combined to create a larger one.:angel:
I, too, am familiar with audio and the center-trapped transformer used as a phase splitter to drive a push-pull output stage by a single-ended intermediate stage. If you think about it, this truly is a matter of signal inversion, and not a timing offset/delay.Winnie (and others), the circuits I were describing are tube based audio. Some may feel they aren’t relevant but a transformer doesn’t know that and acts the same regardless. I think it is easier to see the 180 vs -1 point when considering music. There you have no pure sine waves and R,C, and L are always in play.
The 120, the 240, the 208 Volts are RMS values, not peak as shown on the waveforms in the diagrams.Hmmmm.


Both are types of common single phase services.
Math works both set ups. Two signals combined to create a larger one.:angel:

