Power factor
- Thread starter mbrooke
- Start date
- Status
Back to this. Supply power generally comes from a power transformer. DC in that would be unusual. Saturated transformers and all that.
I have seen power circuits containing both DC and AC components but this has been the DC output of rectifier circuits. I was involved in the design of 6, 12, and 24-pulse rectifiers up to several MW.
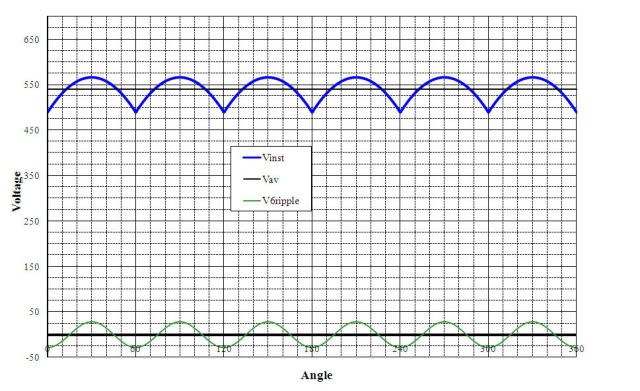
As shown, there is an AC component in the DC at six times supply frequency but the supply side of the bridge doesn't see a mixture of AC and DC.
it has a DC component
v = sinwt, note no dc
i = sinwt, note no dc
assume pf=1
v x i = sinwt^2 = 1/2 (1 - cos2wt), note now there is dc
the 1/2 x 1 is a DC offset component
It's not DC.
As shown, there is an AC component in the DC at six times supply frequency but the supply side of the bridge doesn't see a mixture of AC and DC.
Yes it does
you can measure the ripple
but you are mixing apples and oranges, rectifiers with none
we had a scenario where a ac relay was applied to detect a vfd dc bus fault
fault was limited to (1.35 x 480) /2 / 18.4 Ohm = 17.6 A by a NGR
if the relay (Bender 423 series) was turned down to 1 A it would trip
it was rated for > 30 Hz
it would not trip at >1 A
we scoped it
it was seeing the 4-6% ac ripple
mbrooke
Batteries Included
- Location
- United States
- Occupation
- Technician
The DC part has me now confused.
mbrooke
Batteries Included
- Location
- United States
- Occupation
- Technician
How though?
wwhitney
Senior Member
- Location
- Berkeley, CA
- Occupation
- Retired
You guys are using different terms to refer to the same math.
When the I and V waveforms have 0 average value (usual AC, no "DC" components), then the product I*V waveform typically will not have 0 average value. It will be, e.g., a sinewave with an offset, i.e. sin(a*t) + b. The disagreement is whether to call that offset a "DC" components to the power waveform or not.
Cheers, Wayne
When the I and V waveforms have 0 average value (usual AC, no "DC" components), then the product I*V waveform typically will not have 0 average value. It will be, e.g., a sinewave with an offset, i.e. sin(a*t) + b. The disagreement is whether to call that offset a "DC" components to the power waveform or not.
Cheers, Wayne
assume
pf = 1
all pu quantities
single phase
v = sinwt
i = sinwt
w = 2 Pi f
Power = v x i = sinwt x sinwt = sinwt^2 = 1/2(1- cos2wt)
simple trig identity
you now have a power signal:
Whose frequency is 2 x f
whose phase is shifted by 90 deg from v and i (sin vs cos)
whose AC component magnitude is 1/2 of either v or i (1/2cos2wt bs 1sinwt)
And who has a DC component offset of 1/2
mbrooke
Batteries Included
- Location
- United States
- Occupation
- Technician
Would this not be RMS power instead of DC?
No DC...
Yes, the sinusoidal waveform is offset (i.e. the mean value is not zero), but it is not "DC offset".
Net consumed power average. What most consumers get billed for.
RMS is waveform peak/(sqrr2)
wwhitney
Senior Member
- Location
- Berkeley, CA
- Occupation
- Retired
I think we can agree it has a constant component and a sinusoidal component. Apparently many find it objectionable to refer the the constant component of a power waveform as a DC component, since it is not a current waveform, and the current waveform in question has no DC component.
Cheers, Wayne
wwhitney
Senior Member
- Location
- Berkeley, CA
- Occupation
- Retired
but power, the product of v and i does
wwhitney
Senior Member
- Location
- Berkeley, CA
- Occupation
- Retired
Yes, it has a constant component, the disagreement is in terminology, should that constant component be called a "DC" component, when it arises strictly from AC? I would just call it a constant component.
Cheers, Wayne
- Status