You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Something easy but dont know
- Thread starter Timboe
- Start date
- Status
- Not open for further replies.
roger said:Rattus, you are right that 831 can not be measured anywhere and neither can 1.732 as a meaningful measurement, but 831 as a shortcut multiplier or divisor gets to the point. :roll:
Roger
Roger, it may get the right numbers, but it is not correct. Why not just use the complete formula for 3-phase power? For example, apparent power is given by,
Pa = 1.732 x Iline x Vline
Of course, the magic number cannot be measured directly because it is a unitless ratio. But, we do not need to measure it. We already know,
2 x cos(30) = 1.732
BTW, your line-to-line current should be 115.5A. not 200A.
Rattus, you are trying to take this to an engineering level which is completely unnecessary for this problem or the typical electrical exam.
Now back to the OP, the easiest way to prove the 200 amp phase to phase capacity of this breaker is to connect a load bank to it and load it to the gills, you will see it exceeds 115.5 amps.
Now as far as three phase calculation short cuts, use 360 for 208, 398 for 230, 797 for 460, 831 for 480, or what ever.
When taking exams short cuts are valuable tools.
One more point, there is no reason for electricians ( or engineers for that matter) to worry about absolute precission in feild calculations, the power dilevered is not constant long enough to worry about it, in otherwords, you would be continuously doing calculations at the speed of light to keep up, so averages or close numbers are fine. :wink:
Roger
Now back to the OP, the easiest way to prove the 200 amp phase to phase capacity of this breaker is to connect a load bank to it and load it to the gills, you will see it exceeds 115.5 amps.
Now as far as three phase calculation short cuts, use 360 for 208, 398 for 230, 797 for 460, 831 for 480, or what ever.
When taking exams short cuts are valuable tools.
One more point, there is no reason for electricians ( or engineers for that matter) to worry about absolute precission in feild calculations, the power dilevered is not constant long enough to worry about it, in otherwords, you would be continuously doing calculations at the speed of light to keep up, so averages or close numbers are fine. :wink:
Roger
tallgirl
Senior Member
- Location
- Glendale, WI
- Occupation
- Controls Systems firmware engineer
rattus said:Roger, it may get the right numbers, but it is not correct. Why not just use the complete formula for 3-phase power? For example, apparent power is given by,
Pa = 1.732 x Iline x Vline
Of course, the magic number cannot be measured directly because it is a unitless ratio. But, we do not need to measure it. We already know,
2 x cos(30) = 1.732
BTW, your line-to-line current should be 115.5A. not 200A.
If you're asked for an answer, Roger's shortcuts are the correct approach. If you're asked to show all your work, including formulas used, you're correct.
When it comes to test taking, speed and accuracy matter.
When it comes to the real world, accuracy is hard to achieve, and as Roger points out, you have to measure continually. Here's an example -- consider a purely resistive load where resistance increases with temperature. As resistance increases, voltage at the load decreases, power at the load decreases and (naively) temperature should go down and with it resistance, thereby undoing everything that got you here in the first place. There's an entire area of mathematics -- differential equations -- which is useful in answering these questions, but way beyond what is appropriate for this particular problem.
take it easy is the answer 200 on each leg
take it easy is the answer 200 on each leg
Ok dont confuse me. Is the basic answer that the 200 amp breaker is full when any one leg gets to 200 amps or is it 115.5? In fantasy land say i was allowed to run a 200 amp 3 phase breaker at 100% only and no more if i had on leg 1 199amps leg two 199amps leg three 199amps and yes thats with everything on. Would that be the answer or would it be 115.5 on each leg because the other guy says 115.5 x1.73=199.8.
take it easy is the answer 200 on each leg
Ok dont confuse me. Is the basic answer that the 200 amp breaker is full when any one leg gets to 200 amps or is it 115.5? In fantasy land say i was allowed to run a 200 amp 3 phase breaker at 100% only and no more if i had on leg 1 199amps leg two 199amps leg three 199amps and yes thats with everything on. Would that be the answer or would it be 115.5 on each leg because the other guy says 115.5 x1.73=199.8.
Timboe said:Ok dont confuse me. Is the basic answer that the 200 amp breaker is full when any one leg gets to 200 amps or is it 115.5? In fantasy land say i was allowed to run a 200 amp 3 phase breaker at 100% only and no more if i had on leg 1 199amps leg two 199amps leg three 199amps and yes thats with everything on. Would that be the answer or would it be 115.5 on each leg because the other guy says 115.5 x1.73=199.8.
Timboe, what I am saying is that, with a balanced delta load and a current of 115.5A in each of the three load elements, the line current in each line is 200A.
With a balanced wye load and a current of 200A in each load element, the current in each line is 200A.
In either case, the power is the same.
Smart $ said:Umm... I believe your second line is a little misleading, too. It is accurate when all three lines are conducting equally...
I_A-B = I_B-C = I_C-A = 115.5A
However, in a maximally unbalanced Line-to-Line situation...
I_L-L,max = I_L,max = 200A...where the Line-to-Line load(s) are across only one combination of three lines, say A-B, and may conduct up to 200A. Any additional load to either line in a maximally unbalanced state will exceed the trip rating. The other Line, in this case Line C, can still conduct 200A but no part of that 200A can be conducted through Line A or Line B. Therefore, the current has to return to the system through another conductor. In this case, that would be a neutral in a wye-configurated system...
I_A-B,max = I_C-N,max = 200A
What it all amounts to is, the vectorial sum of currents cannot exceed the trip rating on any one Line.
Smart, I already said balanced load, nuf sed.
tallgirl said:If you're asked for an answer, Roger's shortcuts are the correct approach. If you're asked to show all your work, including formulas used, you're correct.
When it comes to test taking, speed and accuracy matter.
When it comes to the real world, accuracy is hard to achieve, and as Roger points out, you have to measure continually. Here's an example -- consider a purely resistive load where resistance increases with temperature. As resistance increases, voltage at the load decreases, power at the load decreases and (naively) temperature should go down and with it resistance, thereby undoing everything that got you here in the first place. There's an entire area of mathematics -- differential equations -- which is useful in answering these questions, but way beyond what is appropriate for this particular problem.
Tallgirl, I see no need for a shortcut to a formula comprising two variables and a constant. It actually takes longer to do two calculations. Furthermore it is confusing to the readers to compute a non-existent voltage.
As for accuracy, one can easily achieve three significant figures knowing full well that the readings may change in a few seconds. And, it is true that this is more than enough for troubleshooting. Usually one waits for the system to stabilize in order to obtain a steady state reading.
No one is suggesting the use of any math higher than simple algebra. It is a simply a matter of evaluating proven formulas, however it is to one's advantage to understand the formulas to avoid using the wrong one.
Do you mean like this?rattus said:Smart, why don't you post a diagram of this problem showing the line and load currents for a balanced delta configuration? Your diagrams are so pretty.
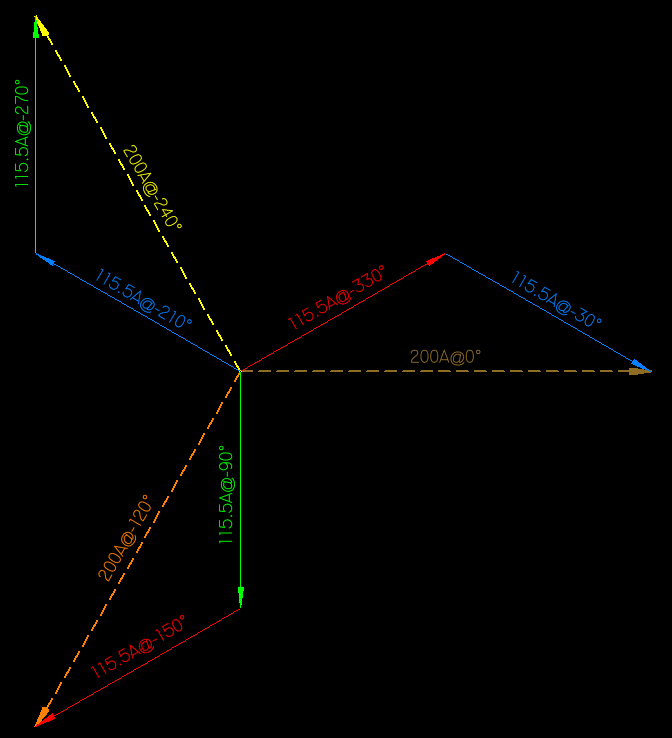
I know you did, but it was regarding kVA and was only inferred regarding amperage...rattus said:Smart, I already said balanced load, nuf sed.
I said it was confusing. I did not say it was incorrect or inaccurate.
Its not an either one or the other answer, as both are the same. It depends on whether you are measuring line or load current...Timboe said:Ok dont confuse me. Is the basic answer that the 200 amp breaker is full when any one leg gets to 200 amps or is it 115.5? In fantasy land say i was allowed to run a 200 amp 3 phase breaker at 100% only and no more if i had on leg 1 199amps leg two 199amps leg three 199amps and yes thats with everything on. Would that be the answer or would it be 115.5 on each leg because the other guy says 115.5 x1.73=199.8.
When you have two (2) Line-to-Line loads connected with only one Line in common, for example one A to B and the other A to C, and both loads conducting 115.5A, these loads would require 200A of current from the common Line, in this case Line A.
In the case of three such loads, forming a balanced delta-connected (Δ) configuration, each load will conduct 115.5A and each Line will provide 200A. This is also true of phase segments of a 3? load. See diagram above. As mentioned previously, load currents are added vectorially, not arithmetically.
FWIW, I agree. With computations, there should always be a direct indication of how you arrived at the solution.rattus said:Tallgirl, I see no need for a shortcut to a formula comprising two variables and a constant. It actually takes longer to do two calculations. Furthermore it is confusing to the readers to compute a non-existent voltage.
As for accuracy, one can easily achieve three significant figures knowing full well that the readings may change in a few seconds. And, it is true that this is more than enough for troubleshooting. Usually one waits for the system to stabilize in order to obtain a steady state reading.
No one is suggesting the use of any math higher than simple algebra. It is a simply a matter of evaluating proven formulas, however it is to one's advantage to understand the formulas to avoid using the wrong one.
Doesn't matter, the shortcut provides the correct answer.rattus said:Tallgirl, I see no need for a shortcut to a formula comprising two variables and a constant.
Help me out here and walk us through this statementrattus said:It actually takes longer to do two calculations.
The non-existent voltage is taught as a shortcut in many instructional courses, so it may only be confusing to you.rattus said:Furthermore it is confusing to the readers to compute a non-existent voltage.
I monitored voltage on a feeder at a hospital last month and had over 8,000 voltage changes in a 24 hour reading, so how long would one need to wait for the system stabilize to give us more than an approximate?rattus said:As for accuracy, one can easily achieve three significant figures knowing full well that the readings may change in a few seconds. And, it is true that this is more than enough for troubleshooting. Usually one waits for the system to stabilize in order to obtain a steady state reading.
And where is the wrong one in this thread?rattus said:No one is suggesting the use of any math higher than simple algebra. It is a simply a matter of evaluating proven formulas, however it is to one's advantage to understand the formulas to avoid using the wrong one.
Roger
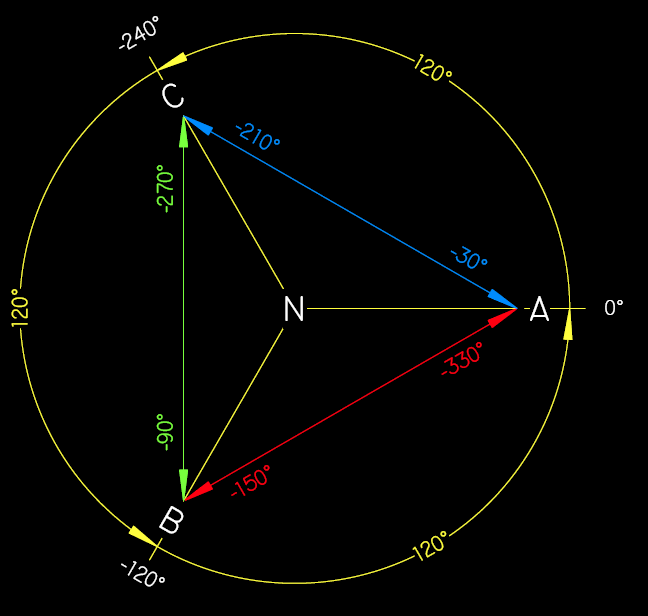
This one?rattus said:Smart, nice phasor diagram, now add a schematic to indicate the paths of the line and load currents with a balanced delta load.
- Location
- Wisconsin
- Occupation
- PE (Retired) - Power Systems
georgestolz said:When I suggested that a 60A breaker would last longer, I got a funny look. Was I wrong?
My thought is, a breaker has a thermal and a magnetic trip mechanism, right? If the breaker is loaded to 100% of it's rating for two hours at a time consistently, it seems to me it would fail (over time) faster than a 60A breaker under the same conditions, due to operating the breaker so close to it's rating.
Edit to add: I assume the thermal portion of the breaker would wear quicker.
George,
The thermal portion of a thermal-magnetic circuit breaker is usually (maybe 99.99999% of the time) a bi-metallic connection not drastically different than the one in some line-voltage thermostats. It can not be worn out by being operated at or near capacity. Even frequent operation does not wear out the thermal element in the breaker although the latching mechanism will probably suffer.
necnotevenclose
Senior Member
Timboe said:Hi,
To find the total amps on a 480volt three phase system. How do you find the total amps? Do you add the amps on the three legs together to come up with a total sum. Say i have a 200 amp breaker and put a meter on leg 1 and got 50 amps and the same on leg two and three would the total amps be 150? How many amps can i have on each leg saying all are equal to max out this 200 amp breaker.
thanks timboe
Timboe,
If you are adding load to an existing facility the NEC says you need at least the maximum demand over a one year period or the peak demand meter reading over a 30 day (not 29 3/4 days) then based on that peak demand meter reading which should be (1) number you can subtract the OCPD rating from the peak demand and find out how much load you can add. However, it has been my experiance that you just cannot look at the OCPD for the available rating at the panel you also have to look at what the wire size feeding that panel and if that panel is downstream from another panel does that "master panel" have an equal or higher rating. Worstcase Scenario: For example if you have a 200A main which is fed with only 175A feeder and this panel is a subfeed panel out of the master panel with only 150A then your new total demand load cannot exceed 150A. But to try and answer your email you state there is a 200A OCPD with 50A-PhA, 50A-PhB & 150A-PhC. Thats 250A on a 200A OCPD no load can be added. If I were you I would try to make sure that the meter was set up appropriately. Also try to contact the utility to get the peak demand over one year. Calculation for Amps first figure voltage "831V=1.732*277V then to figure amps 200A=166200VA/831V.
- Location
- Massachusetts
Timboe said:Ok dont confuse me. Is the basic answer that the 200 amp breaker is full when any one leg gets to 200 amps or is it 115.5?
200 amps.
When any of the 3 poles exceeds 200 amps the breaker should trip.
In fantasy land say i was allowed to run a 200 amp 3 phase breaker at 100% only and no more if i had on leg 1 199amps leg two 199amps leg three 199amps and yes thats with everything on.
First off that is not fantasy land, we can run breakers at 100% non-continuously (less than 3 hours at a stretch).
But other than that yes, a standard 200 amp breaker is designed to carry 200 amps on all three legs at the same time for up to 3 hours.
- Location
- Massachusetts
rattus said:Timboe, what I am saying is that, with a balanced delta load and a current of 115.5A in each of the three load elements, the line current in each line is 200A.
With a balanced Wye load and a current of 200A in each load element, the current in each line is 200A.
In either case, the power is the same.
Rattus I appreciate that you provided Timboe with such a clear answer.:roll:
roger said:Rattus, you are trying to take this to an engineering level which is completely unnecessary for this problem or the typical electrical exam.
Now back to the OP, the easiest way to prove the 200 amp phase to phase capacity of this breaker is to connect a load bank to it and load it to the gills, you will see it exceeds 115.5 amps.
I agree with both points completely.
Besides, I don't think this is even a question for a test, it appears to me that Timboe just wanted a straight answer to a question to satisfy his own curiosity.
Last edited:
- Location
- Massachusetts
rattus said:No one is suggesting the use of any math higher than simple algebra.
I don't recall the poster asking about how you calculate anything.
Last edited:
- Location
- Windsor, CO NEC: 2017
- Occupation
- Hospital Master Electrician
Thanks for the correction, Jim.jim dungar said:It can not be worn out by being operated at or near capacity.
- Status
- Not open for further replies.

