crossman
Senior Member
- Location
- Southeast Texas
Winnie, I see your point although I need to put more thought in it.
For now, concerning the helicopter, I just cannot see that there could be any other way of looking at this than the diagram drawn below. I have broken it down to its most elementary components.
The helicopter cannot be looked at as some isolated body unaffected by the charge in the earth.
Now, if we want some semantic mish-mash of words that a capacitor does not "really and truely" complete a circuit for current flow, then I will toatlly agree with you. However, in most academic circles, I would bet that the capacitor is considered to be a completion of the circuit.
Rattus, expanding on your thought:
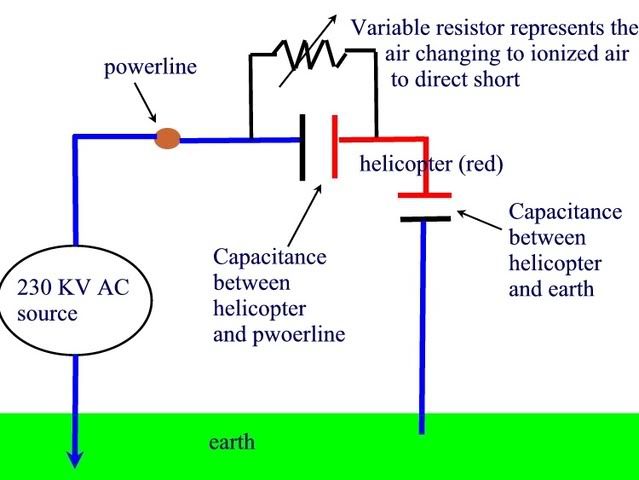
For now, concerning the helicopter, I just cannot see that there could be any other way of looking at this than the diagram drawn below. I have broken it down to its most elementary components.
The helicopter cannot be looked at as some isolated body unaffected by the charge in the earth.
Now, if we want some semantic mish-mash of words that a capacitor does not "really and truely" complete a circuit for current flow, then I will toatlly agree with you. However, in most academic circles, I would bet that the capacitor is considered to be a completion of the circuit.
Rattus, expanding on your thought:

Last edited:

