Just to clarify.

[rattus]

3-phase anyone?

3-phase anyone?

Are we ready to tackle something really hard? Like 120/208 Wye?

 

[jim dungar]

It certainly is confusing isn't it? Now, when computing average power, current must be LEAVING the source in question. We do that on paper just by reversing the arrow and shifting the phase by 180 degrees. The average power is positive as it has to be.

All in the way you look at it.

Only your method is confusing.


If current must be LEAVING the source then you should not define the sources as you have.

Please show how the current in the two wire circuit can LEAVE Van and also LEAVE Vbn.

Please show how simply adding a center tap connection changes the two wire source currents.

I agreed that the average power must be positive however it is your choice of voltage sources that makes it look negative. Again, you said that Vbn = -Van, so therefore it looks like P=-IVan

 

[jim dungar]

Are we ready to tackle something really hard? Like 120/208 Wye?

Not until you finish explaining the currents in a 3-wire balanced center tap and how they differ from the 2-wire circuit which was my starting point. Then I planned to look at my very original unbalanced 3 resistor circuit.

So far, I see that your method of describing these two 120 voltage sources as requiring additional circuit analysis steps rather than straight forward high school formulas, because as you have said "it is tricky". If your method makes a 2-wire circuit tricky what happens to complex ones?

 

[LarryFine]

Are we ready to tackle something really hard? Like 120/208 Wye?

Wye? Why? :-? grin




Did you hear about the new philosophic talk radio station? WYOY

 

[jghrist]

Jim, does this graphic help you out? If not, just tell me what needs to be modified. (I didn't feel like making a whole new drawing, so this is just photoshoped from the text book scan I took earlier. That's why the names are different.)

With the voltages as defined, there will be no current. Write the loop equation:

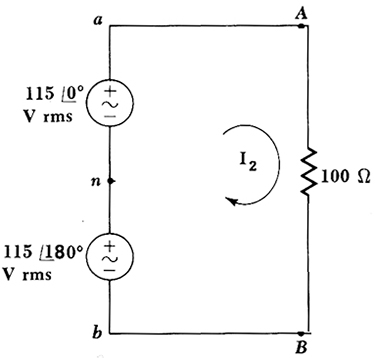
115V@180? + 115V@0? = I2?100ohm

115V@180? = -115V@0?

-115V@0? + 115V@0? = I2?100ohm

0V = I2?100ohm

I2 = 0A

 

[jim dungar]

With the voltages as defined, there will be no current. Write the loop equation:

You are forgetting that the method Rattus uses is to subtract the voltages, he said this is tricky. There are hundreds and hundreds of posts describing his reasoning.






Just because you can, doesn't mean you should.

 

[rattus]

Only your method is confusing.


1. If current must be LEAVING the source then you should not define the sources as you have.

2. Please show how the current in the two wire circuit can LEAVE Van and also LEAVE Vbn.

3. Please show how simply adding a center tap connection changes the two wire source currents.

4. I agreed that the average power must be positive however it is your choice of voltage sources that makes it look negative. Again, you said that Vbn = -Van, so therefore it looks like P=-IVan

1. If we use the neutral as a common reference, we have no choice. One voltage is the inverse of the other.

2. They cannot, the current has to ENTER the second source.

3. They do not change.

4. The fact that the current is entering one source allows us to slip in a negative sign to make the product positive.

 

[rattus]

Not so:

Not so:

With the voltages as defined, there will be no current. Write the loop equation:

115V@180? + 115V@0? = I2?100ohm

115V@180? = -115V@0?

-115V@0? + 115V@0? = I2?100ohm

0V = I2?100ohm

I2 = 0A

Remember these voltages are referenced to the common neutral. To obtain the voltage between them, we must SUBTRACT not add one to the other, e.g,

Vab = 115 @ 0 - Vab @ 180 = 115 @ 0 + 115 @ 0 = 230V @ 0. This is a critical point which can be demonstrated with a phasor diagram.

 

[rattus]

You are forgetting that the method Rattus uses is to subtract the voltages, he said this is tricky. There are hundreds and hundreds of posts describing his reasoning.


Just because you can, doesn't mean you should.

You don't have to unless you are computing L-L voltages in a wye.

 

[rattus]

Remember:

Remember:

BTW, this all got started because I insisted that the OP in another tread was told V1n and V2n were not separated by 180 degrees.

 

[jim dungar]

1. If we use the neutral as a common reference, we have no choice. One voltage is the inverse of the other.

2. They cannot, the current has to ENTER the second source.

3. They do not change.

4. The fact that the current is entering one source allows us to slip in a negative sign to make the product positive.

So, is this a correct interpretation?

1. The current must always leave the source.
2. The current cannot always leave the source.
3. The currents in source 1 is identical to the current in source 2.
4. If the current is not in phase with your chosen voltage, fix the math by changing the direction of that current only for the part of the circuit giving you problems rather than changing the way the voltage is referenced.

 

[Rick Christopherson]

4. The fact that the current is entering one source allows us to slip in a negative sign to make the product positive.

Slip In: verb, Insert casually; stealth; interject without notice; "She slipped in a negative sign without her boss noticing."

 

[quogueelectric]

The correct term is "fuzzy math"

 

[mivey]

no analysis impasse

no analysis impasse

I think (maybe?) I finally see what Jim is getting at (correct me if I'm wrong…and I know you will). He wants to be sure we have our signs correct before we start any analysis. I didn't see it made any difference because the positives and negatives carry through as needed. We can start any analysis by drawing the loop currents in the CW or CCW direction. The end result will show if the choice we made agrees with our definition of voltage polarity and current flow for positive values.

I was able to calculate the voltages and currents so couldn't see where there was any failing. I understand that Jim is not saying that the circuit analysis reaches an impasse, only that if you have made a "wrong" assumption about the directions in the beginning, you will have some negatives at the end. Is having a negative the whole problem? Is it really "wrong?

Are we in agreement about the following basic definitions of voltages and flows?

View attachment 1318

[edit: there should have been a note at the absorbed/delivered power definition that power is VI, of course]

 

[jghrist]

Remember these voltages are referenced to the common neutral. To obtain the voltage between them, we must SUBTRACT not add one to the other, e.g,

Vab = 115 @ 0 - Vab @ 180 = 115 @ 0 + 115 @ 0 = 230V @ 0. This is a critical point which can be demonstrated with a phasor diagram.

Then you need to reverse the + and - on the lower source symbol.

 

[mivey]

Slip In: verb, Insert casually; stealth; interject without notice; "She slipped in a negative sign without her boss noticing."

You so funny:grin:

 

[jim dungar]

Is it really "wrong?

I have been trying to point out the advantages and disadvantages of different presentations.

Making an adjustment (i.e. so that the voltages seem correct) can lead to having to make other adjustments later.





Just because you can doesn't mean you should.

 

[mivey]

Is having a negative the whole problem? Is it really "wrong?

BTW, I'm not being argumentative, this is a real question.

[edit: Jim, you were too fast!]

 

[mivey]

I have been trying to point out the advantages and disadvantages of different presentations.

Making an adjustment (i.e. so that the voltages seem correct) can lead to having to make other adjustments later.

No argument from me.

 

[LarryFine]

Remember these voltages are referenced to the common neutral.

You say that as if it's a universal requirement.

Slip In: verb, Insert casually; stealth; interject without notice; "She slipped in a negative sign without her boss noticing."

I slipped in a bathtub once; does that count?