Hameedulla-Ekhlas
Senior Member
- Location
- AFG
Yes, that is the simple HOW.
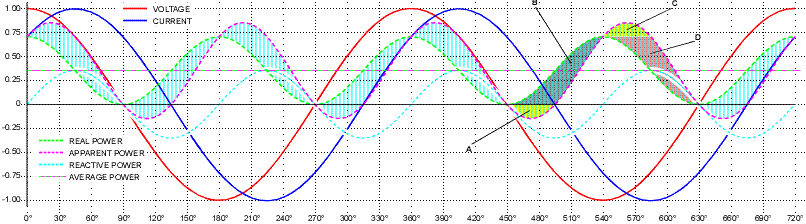
But I was hoping to see Ham' come up with the "proofing" answer, since he went to such degree for proofing the plot of power (when pf=1) based on Pavg. The exercise, per se, was more a question of proofing the relationship of scalar and vector approaches using S, P, and Q (hint).
Smart$:
Regarding to pf = 1 for instantaneous power is already stated by Fryze and I have mentioned many times 0v = 0i for you
"Fryze verified that the active power factor (f) reaches maximum ( f = 1 ) if and only if the instantaneous current is proportional to the instantaneous voltage, otherwise power factor ( f < 1 ). "
Please do not ask me, who is Fryze.