Hameedulla-Ekhlas
Senior Member
- Location
- AFG
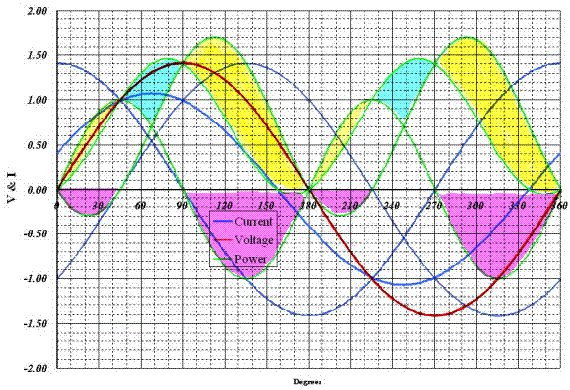
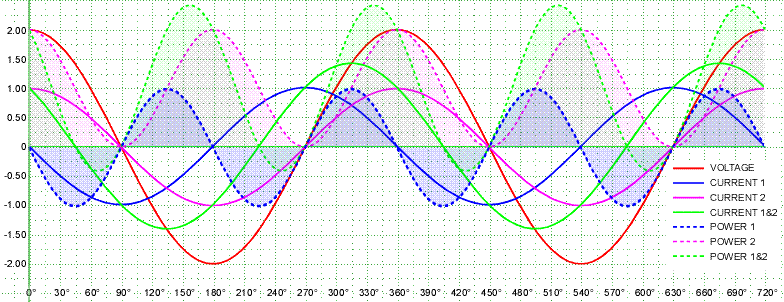
You guys are really arguing apples and oranges. What you say is correct from a statics standpoint, but what the other guys are talking about is from a dynamics perspective, and never the twain shall meet unless you are talking about a DC circuit.
Gunn:
Would you please give some information regarding to apples and oranges and the difference. :grin: