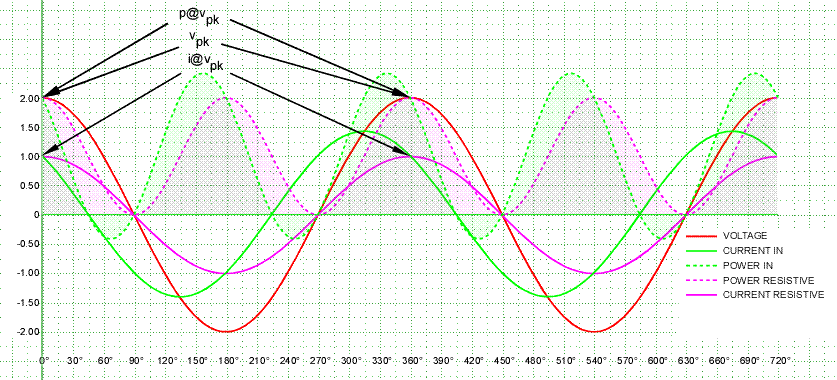
I don't disagree with any of that.N
But when there is a resistive load added to say your capacitive load, the instantaneous power changes. In the case of the loads being parallel, the power "input" is portioned to each load. All three?input, resistive load, and capcitive load?can be evaluated and plotted separately. As such, it can be determined that the equation for the input power is equal to the equation for the resistive load added to the capacitive load's equation.
Also note the peak instantaneous power of the three is at different points in time. The input instantaneous power's peak is somewhere between the capacitive load's and the resistive load's. When we analyze this shift in timing, we find the capacitive and resistive components of the input instantaneous power are related to the amplitude of the components' current and the shift between the each of the three power waveforms.
I'm gonna stop here, for I don't want to waste my time typing on deaf-eared eyes... continuation is dependent on your reply :roll:
The waveforms you posted show voltage, two currents, their sum, the resulting powers. When voltage and current are of the same polarity, power is shown as positive and it is negative when current and voltage are of opposite polarities. And of course zero when either current or voltage is zero.
It concurs with exactly what I have been saying over the past 500 or so posts.
Maybe persistence works.
:grin: