Question about calculating voltage drop on 3 phase inductive circuits
- Thread starter Crohnos01
- Start date
- Status
mivey
Senior Member
I should have noted that those were for 85% p.f.
At 85?F and 90% p.f. here are the copper R to effective Z percentages in three conduit types:
CU: PVC, Al, St
18-1: 110.6%, 110.6%, 110.5%
18-7: 110.6%, 110.6%, 110.5%
16-1: 110.4%, 110.4%, 110.2%
16-7: 110.4%, 110.4%, 110.2%
14-1: 109.9%, 109.9%, 109.6%
14-7: 109.9%, 109.9%, 109.6%
12-1: 109.4%, 109.4%, 109.0%
12-7: 109.4%, 109.4%, 109.0%
10-1: 108.5%, 108.5%, 107.9%
10-7: 108.5%, 108.5%, 107.9%
8-1: 107.1%, 107.1%, 106.1%
8-7: 107.1%, 107.1%, 106.1%
6-7: 104.9%, 104.9%, 103.4%
4-7: 102.1%, 102.1%, 100.1%
3-7: 100.4%, 100.4%, 98.0%
2-7: 97.9%, 98.5%, 95.6%
1-19: 94.6%, 95.5%, 92.4%
1/O-19: 92.0%, 93.2%, 88.2%
2/O-19: 89.3%, 89.3%, 85.0%
3/O-19: 84.8%, 86.1%, 80.9%
4/O-19: 80.8%, 82.5%, 76.1%
250-37: 76.7%, 78.9%, 71.8%
300-37: 72.7%, 75.3%, 67.6%
350-37: 69.5%, 72.7%, 64.3%
400-37: 65.8%, 69.5%, 61.9%
500-37: 61.0%, 65.7%, 57.3%
600-61: 56.6%, 62.0%, 53.2%
700-61: 53.4%, 59.5%, 49.9%
750-61: 52.0%, 58.5%, 48.4%
800-61: 50.8%, 57.3%, 47.7%
900-61: 48.4%, 54.9%, 46.4%
1000-61: 46.3%, 52.8%, 45.3%
1250-91: 41.5%, 47.9%, 42.3%
1500-91: 38.1%, 44.4%, 40.5%
1750-127: 35.4%, 41.5%, 39.0%
2000-127: 33.2%, 39.1%, 37.7%
of course, Z (not effective Z) does not change with p.f.
mivey
Senior Member
If you were here, I'd say you had not had time for coffee. I guess we will call it the effects of a long day since it is quittin' time over there.
mivey
Senior Member
It is from the approximate formula for voltage drop:
Vdrop = I*(RcosΘ + X sinΘ)
FWIW, the exact formula is:
Vdrop = Vsending + I*(RcosΘ + X sinΘ) - sqrt(Vsending2 - (IRcosΘ - IX sinΘ)2 )
While all values required for in the exact expression for Vdrop =I*Z, where I is load current and Z =[||Z|cosΘ +j|Z|sinΘ|]=[(R2+X2)1/2] is impedance of the circuit, can be obtained from the right hand side of the approximate expression Vdrop = I*(RcosΘ + X sinΘ), I wonder why the approximate expression need be used for calculation of Vdrop.
Crohnos01
Member
- Location
- BG, Washington
Wow, thanks guys. Good catch! I had originally used Table 430.250 and then... I don't know... too many times between version 1 and now went by and I dropped it somewhere. The project has seen this motor go from a 30 Hp, to a 10 Hp, to two 7.5 Hp, to a single 15 , to two 10 Hp, back to a single 15.... back and forth about 15 times. I think the M.E. is trying to give me a stroke by changing the dang thing faster than I can change socks. I finally gave up and decided to wait until he nailed down the number for sure and then restarted my calculations which is when I lost the table. If this was the only project I had on my desk that would be one thing, but you know the engineering gig.... feast or famine...The 50' run is definitely a blessing and honestly, I am being conservative in that number. If the electrician makes the run as the crow flies, it's probably only going to be about 30'.
Very interesting discussion regarding effective Z... I see now the change in the factors at about 1/0 you mentioned. I am going to have to go through these posts in a little more detail and make sure I soak up all this information.
Excellent discussion and information! Thanks everyone! :thumbsup:
Crohnos
Very interesting discussion regarding effective Z... I see now the change in the factors at about 1/0 you mentioned. I am going to have to go through these posts in a little more detail and make sure I soak up all this information.
Excellent discussion and information! Thanks everyone! :thumbsup:
Crohnos
All of which show R ≤ Z.
mivey
Senior Member
By definition that will always be the case. Not so for "effective Z" (NEC term) which is simply a voltage drop factor.
OK.
Voltage drop is IZ. Current times impedance. If R ≤ Z always, why, how, can IR ever exceed IZ?
Maybe an example of a practical application with actual numbers would be helpful.
Don't forget that when you calculate VD=IZ that you are removing the impedance of the load from the calculation. The load is the main constituent of the circuit's power factor. That's why you have to use ZEFFECTIVE. As to a practical application with real numbers...
mivey
Senior Member
Not quite. One is a voltage vector the other is a comparison of magnitudes.
You know the magnitude of I but you don't know the angle. Iterating should find the value.
With iteration I got a 5.223 volt drop instead of a 5.204 volt drop (the exact formula result). Not sure what I missed but the point is that you can't compare the vector difference with the magnitude difference. This is close:
Z = 0.4 + j0.0108 = 0.4<1.55?
Vs = 120<0?
I = 15<-30.345?
IZ = 6.0<-28.8?
Vr = Vs - IZ = 114.8<1.443?
VD = |Vs|-|Vr| = 120-114.8 = 5.2 volts /= 6.0<-28.8?
also,
Θ = Θ_I - Θ_Vr = -30.345? - 1.443? = -31.788?
Cos (Θ) = 0.85 = p.f.
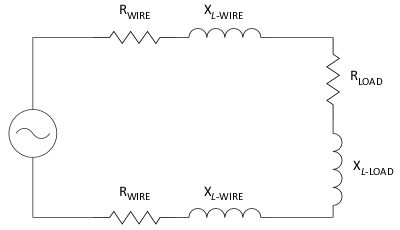
Per NEC Table 9, 8AWG copper conductors in steel conduit...... As to a practical application with real numbers...
XL = 0.065
R = 0.78
ZEFFECTIVE = 0.70 (0.85PF)
Last edited:
- Location
- Placerville, CA, USA
- Occupation
- Retired PV System Designer
Don't forget that when you calculate VD=IZ that you are removing the impedance of the load from the calculation. The load is the main constituent of the circuit's power factor. That's why you have to use ZEFFECTIVE. As to a practical application with real numbers...
I don't see the real numbers in your post. And I am also wondering whether another factor that is coming into play, since it is affecting the larger size conductors more severely, is that somehow the R value calculated for the conductor taking into account skin effect is not the same as the R value which ends up contributing to the VD?
If the only anomaly is taking into consideration or removing the load impedance, the observed difference for larger wires would have to come either from skin effect or the changing magnitude of XL-wire on the larger conductors.
And you're not going to. I'm leaving it as an exercise for the doubter(s)
Mivey gave the defintion/formula for Effective Z. Use the numbers I provided above and calculate it out.
I don't think skin effect is given any consideration.
(note to mivey: FWIW, and don't know if it makes much difference, but Table 9 was developed long before IEEE revised its voltage drop formula to the current "exact formula".)
Answer this, given the numbers above for 8AWG... How can XL cause a 0.85PF?
mivey
Senior Member
Formula typo. The correct formula is:
Vdrop = Vsending + I*(RcosΘ + X sinΘ) - sqrt(Vsending2 - (IXcosΘ - IRsinΘ)2
mivey
Senior Member
It does not matter since the approximate formula as used in the NEC is still used and given in the red book.
mivey
Senior Member
I figured out my mistake. I used the Θ from the voltage and current across the load instead of the load impedance. Now all is good. Latest calc:
Vs: 120.0000+j 0.0000 = 120.0000<0.0000?
Z: 0.4000+j 0.0108 = 0.4001<1.5466?
Zload: 6.5051+j 4.0315 = 7.6530<31.7883?
Θ: <31.7883? (85% p.f.)
Ztotal: 6.9051+j 4.0423 = 8.0013<30.3450?
Is: 12.9429+j -7.5769 = 14.9976<-30.3450?
Vl: 114.7410+j 2.8910 = 114.7774<1.4433?
Vdrop (vector): 5.2226
Vdrop (exact): 5.2226
PS: The angle should have been the same but I fat-fingered something in the other post.
Last edited:
- Status