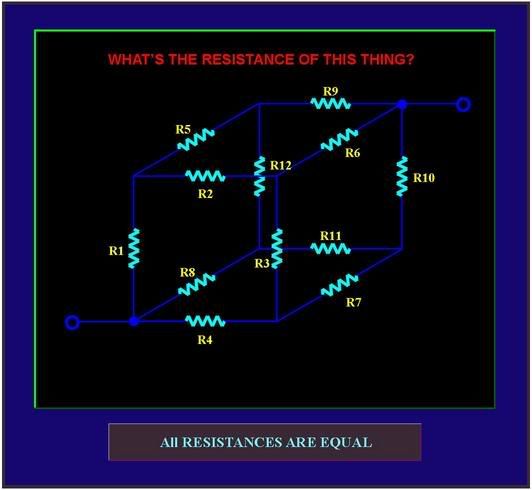
Resistance Cube

[physis]

About 15 years ago a friend of mine brought this up. I haven't tried in a long time but I haven't been able to figure this thing out.

Edit: I'm having to work on the image size.

[ June 10, 2005, 12:02 AM: Message edited by: physis ]

 

[bphgravity]

Re: Resistance Cube

The total resistance is the resistance of any one resistor?

 

[hardworkingstiff]

Re: Resistance Cube

Originally posted by bphgravity:
The total resistance is the resistance of any one resistor?

I won't argue against this, but can you prove it?

 

[bphgravity]

Re: Resistance Cube

Actually, I want to change my first guess. At a closer look, I have determined there are 3 resistors in parallel that are in series with 6 more resistors in parallel that are in series with the remaining 3 resistors in parallel.

Based on that, the resistance between the two points will vary. When all is said and done, I beloeve the answer is: RT= 5R/6

 

[hardworkingstiff]

Re: Resistance Cube

I anxiously await the real answer.

[ June 10, 2005, 08:05 AM: Message edited by: hardworkingstiff ]

 

[physis]

Re: Resistance Cube

I'll say two things.

It is a complex of series and paralell reisistances. Although that's obvious, it became excruatingly obvious after failing to solve it a number of times. I found it much easier to deal with if I converted the diagram to two dimentions.

And. nothing varies.

 

[rattus]

Re: Resistance Cube

Gents, this thing is symmetrical, so you can short the equipotential points and very easily see that the total is 5R/6.

 

[physis]

Re: Resistance Cube

I finally couldn't stand it anymore so i built one and measured it.

You know what is? cause I don't remember.

 

[physis]

Re: Resistance Cube

Alright, I'm not gonna be happy if it gets solved right away, but I'll live.

But anyway, let's see the math.

 

[physis]

Re: Resistance Cube

I think I see what you're saying Rattus. without actually doing it I think I can get an answer that way.

 

[rattus]

Re: Resistance Cube

Sam, you can either short the equipotential points or slice the cube apart to obtain a flat network which can be analyzed easily.

Also, you had better look out! I just got DSL installed today. No more wife nagging me to get off the phone.

 

[bphgravity]

Re: Resistance Cube

This is what I used to gewt my answer:

RT= (R/3) + (R/6) + (R/3) = R(1/3 + 1/6 + 1/3) = 5R/6. What do I win?

 

[physis]

Re: Resistance Cube

I just realized that the R's should be numbered.

I haven't given it much time yet, but so far, I haven't gotten two symetrical resisistances.

I'll put numbers on it and look at it some more.

 

[rattus]

Re: Resistance Cube

Sam, you don't need numbers! Just use "R".

BTW, bph, your solution is about as straightforward as can be.

 

[physis]

Re: Resistance Cube

Well I must be dense.

I'm not disagreeing, but I haven't identified how it relates.

Don't need numbers. You don't need numbers when everybody's on the same page.

Let me see see if I can find R/6, R/3 and R/6.

[ June 10, 2005, 12:48 AM: Message edited by: physis ]

 

[physis]

Re: Resistance Cube

By the way Rattus, you're gonna like DSL. Especially if you had AOL.

 

[physis]

Re: Resistance Cube

And Bryan, nobody wins until I understand.

 

[physis]

Re: Resistance Cube

Ok. The reason I'm having trouble with this explaination is that the six resistances in the middle are not configured as six simple paralell resistances.

 

[rattus]

Re: Resistance Cube

Sam, draw the diagram in a way that shows the symmetry. You will have resistors cross each other, and the intersections indicate the equipotential points.

 

[sandsnow]

Re: Resistance Cube

I don't see it at all, literally. Where's the picture??????