Re: Resistance Cube
By Rattus:
There are no atoms--just solid material with the resisitivity of copper
That?s probably why these poor copper atoms were just hanging out on some electrode in some electrolysis tank somewhere.
Out of work because they?ve been replaced by actors with pointy hats. (These atoms are not bonded in the form of a lattice by the way, I tried, but couldn?t find an image of that)
So anyway, as far as I can tell, the difficulty in answering the question is, amusingly, where to start.
That's the point of the cube.
Because that's the interface. And for all the other obvious reasons.
The bad news is, like it or not, there is a solution. The other bad news is that it relies heavily on detail and accuracy. The good news is that after the first two layers (I think I can keep it to two layers) of atoms the rest of it can be deduced using macro-math and tape measures again, and, the accuracy requirements shouldn't be atypical from that point to the base of the pyramid.
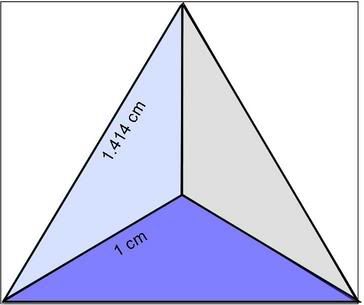
The pyramid I'm refering to is the three sided one at either end of the cube. The cube 'll be 1 cm for convenience. For the time being I'm only concerned with this pyramid.
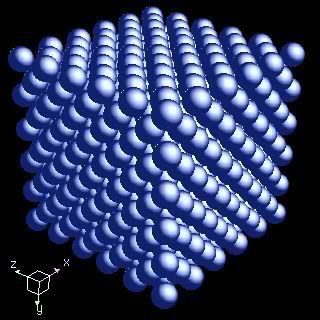
These are the only two images of a three dimensional copper lattice I've been able to find so far. They're both from the same place. What I'm concerned with is the corner. They both indicate that, from the corner toward the base of the pyramid, the structure transitions from one atom to seven atoms.
I confess, I'm no expert. And a lot of what I'm getting into I've had to look up or learn about.
]
Just based on the geometry my contention is that there's an intermediate layer of three atoms between the one and the seven shown in the first two models.
So far, this is the best imformation I have and my confidence in it is good. None the less, it has to be verified. This corner is the foundation of the equation and it's not only hidiously sensitive to error all by itself, but it has an identical twin on the opposite side that'll double the error.
So I'm gonna post what I have so far.
A cell, or unit cell, is the smallest fundamental (sort of redundant) configuration of atoms that an atomic or molecular lattice is built from. A lattice is built from cells just like the cell is built from atoms. The same cell pattern is repeated in the lattice just like the same atoms are repeated in the cell.
There are interface characteristics at the boundaries of cells just like there are between individual atoms. Fortunately in the case of copper, it?s lattice being cubic, has the same parameters in all three axis and those are 90? from each other. Murphy must be out of town on this one.
So the geometry of a copper lattice, being cubic, allows us to use the same math, ratios and angles that apply to any other cube for most considerations. Although I can?t deny Rattus? point that you usually can?t have an intersection pointier than the radius of an outer electron orbital.
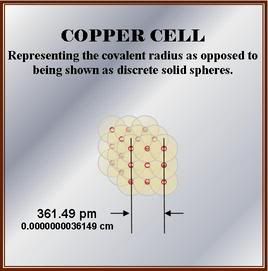
The first representation is unfortunately the most common. The problem with it is that the covalent radius is ignored. The covalent radius is half the distance between two adjacent identical nuclei.
When someone talks about the number of atoms there are across something like the thickness of a penny, (at least every example that I can remember) it?s always presented as something being divided by the diameter of an atom. Which is fine. But that number doesn?t represent the number of atoms you?ll find on a straight line through whatever the object is.
I can?t seem to find the same value for anything in any two places on the internet. But even allowing for some error, if you use as an example the 256 picometers I have for the diameter of a copper atom, and multiply it by two, you can see that you wont get a distance corresponding to that between two copper atoms in it?s lattice structure.
Instead, taking the reported dimensions of a unit cell (that are equal in all three axis remember), and dividing an axis to find the distance between the center of two nucleui, you'll see that, because the atoms are overlapping their electron orbitals, the number of copper atoms in a straight line across it's lattice corresponds to 180.745 picometers instead of the diameter of a single copper atom, 256 picometers. Dismissing any rough value errors I have from source inaccuracies.
So what's gonna happen is that after I decide how to deal with the interface at the corner of the cube, which will be capable of supporting sufficient current to actually measure the resistance in the real world, (It's very interesting why I believe it's possible, any guesses?) the pyramid will be treated as two resitors in series.
The first resistor is what all the atomic stuff is about. The second one is whatever's left of the pyramid. The area of the conductor is proportional to the distance from the corner. So the resistance is inversely proportional.
There's something about the teeny tiny corner that I think actully allows it to work
instead of burn up.