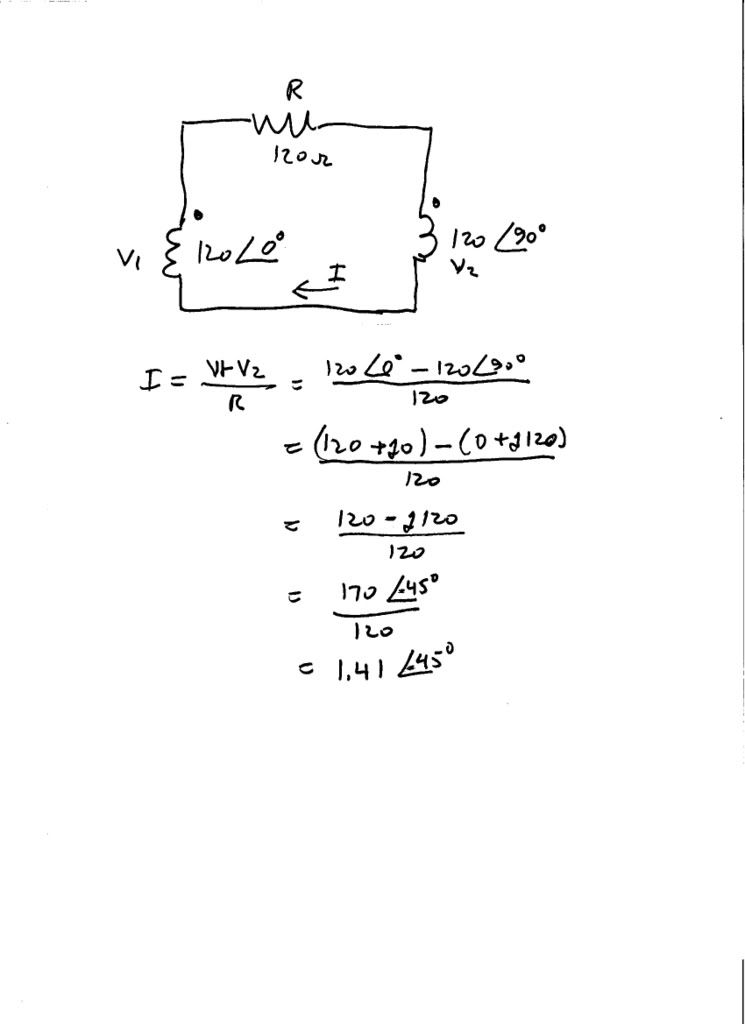
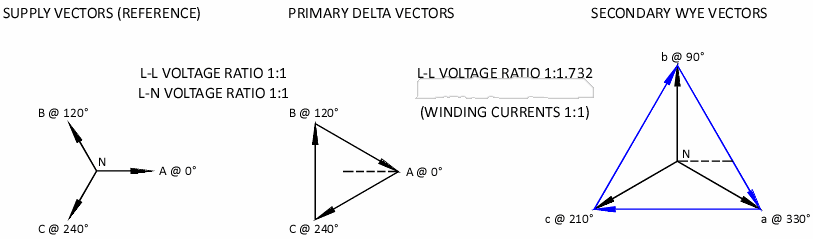
hardworkingstiff said:If I understand this question, you are talking about line to line load (no neutral connection) of a 120/208 'single-phase' circuit.
Delta/Wye would have current on all 3 primary conductors and 2 secondary conductors, but current would only be flowing through 2 primary coils and 2 secondary coils.
Wye/Wye would have current on 2 primary conductors (and the neutral of the primary, but not the 3rd phase conductor) and 2 secondary conductors (with no current on the neutral of the secondary), current on 2 primary coils and 2 secondary coils.
Right/Wrong?
Mostly right, but let's see what others have to say, especially about the relative magnitudes of the currents in the delta-wye case.