single vs. 3 phase

[Smart $]

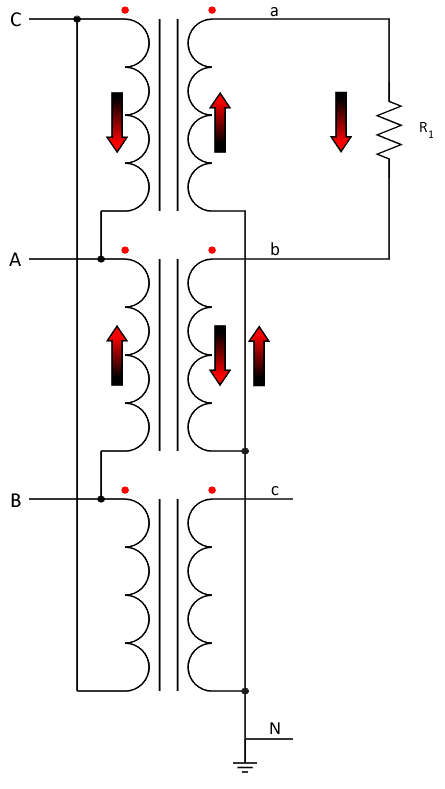
If we let Van=120<0, Vbn=120<-120, Vcn=120<120 then the current through a resistor connected from a to b is (Van-Vbn)/R = 207.85<30 / R. The ANSI/IEEE standard is that the high voltage leads the low voltage by 30 degrees for delta-wye or wye-delta banks. In the field, it may not always be the case. But that was not the point of the illustration. Pick whatever current angle you want (I picked zero for simplicity's sake), and work through the problem and see what you get. I'll check my chicken scratch again but do not have the time right now.

I'm not sure what you are doing with the rotation. I'll read through it again later but it must be the terminology you are using that is throwing me off.

My earlier diagrams have the low voltage leading the high. To have high lead low, the primary coils must be connected reverse polarity from your earlier description (i.e. connected CA?an, AB?bn, and BC?cn).

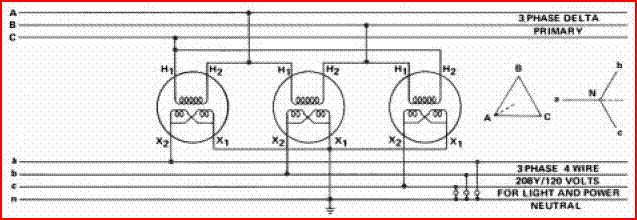
However, this does not change the basic premise of anything I have wrote. I have redrawn my diagram to match the standard delta-wye bank configuration and symbology above.

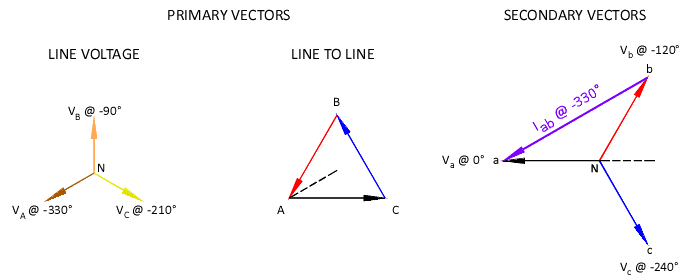
Now, when you talk current vectors primary versus secondary, you have to reverse polarity. So where I note Iab@-330 on the secondary, it transfers to the primary with a phase angle of -150?... the exact opposite angle of what I said earlier... but the common node is no longer Line B, but Line A@-330?, also the exact opposite angle of what I said earlier.

By conforming to this hi lead lo voltage standard has definitely made your numbers wrong. But the question of 'what is right?' still remains...!!!

 

[Lxnxjxhx]

the question of 'what is right?' still remains...!!!

the question of 'what is right?' still remains...!!!

Whatever analysis using whatever conventions that gives you the same answers as the real world.

 

[mivey]

...However, this does not change the basic premise of anything I have wrote

I was referring to the notion that your original drawing would indicate a negative rotation. So this statement is what I was questioning and I'm not sure if you were saying the first and second diagram indicated the same rotation but just had a notation difference:

Sorry, but they are not. I'm using positive phase angle notation in the diagram.

I regret having mentioned it now as it appears to be a distraction from the original point which was the magnitude of the currents, not the phase angles. If it makes it easier, change the resistance to an impedance or use the 30 degree angle, the analysis is the same.

...By conforming to this hi lead lo voltage standard has definitely made your numbers wrong. But the question of 'what is right?' still remains...!!!

Which numbers? If you mean the angles, I should have left them out as it seems to be causing you a problem. If you mean the current magnitudes, what is the math error? Give me something to refer back to so I can have a starting point when I get a chance to look at this in more detail later.

 

[rattus]

How about this?

How about this?

Take a look at the attachment.

 

[mivey]

Take a look at the attachment.

Good! An example with no distracting angles (I wish I had done that instead in post #438).:smile:

This would be a good exercise for Smart $. I have not had a chance to draw a graphic but he should get:
Iab=Ild
Ibc=-Ild
IA=Iab+Ica=Iab=Ild
IB=-Iab+Ibc=-Ild-Ild=-2Ild
IC=-Ibc+Ica=-Ibc=Ild

[edit: rattus, are you trying to be sneaky with the coil orientations? :smile: It should help clear up the point.]

 

[rattus]

KISS: Keep It Simple Silly!

KISS: Keep It Simple Silly!

[edit: rattus, are you trying to be sneaky with the coil orientations? :smile: It should help clear up the point.]

Not sneaky! Just an example of the proper use of polarity dots. This is also a real world example of incorrect initial assumptions which are corrected in the process. Makes a neater diagram too!

 

[rattus]

No!

No!

Smart, your phasor diagrams are wrong! For example, Vb @ -90 points downwards--always!

 

[mivey]

...Makes a neater diagram too!

Certainly does for this example, and it does drive the polarity point home. My chicken scratch had the lines crossing = less neat, but easier for me since I'm not as advanced as you.:smile:

 

[Smart $]

Smart, your phasor diagrams are wrong! For example, Vb @ -90 points downwards--always!

Tell that to whoever decided the orientation of standard transformer diagrams. I just aligned my phasors to the diagrams to help clarify the issue... or so I thought!!!

 

[Smart $]

Certainly does for this example, and it does drive the polarity point home. My chicken scratch had the lines crossing = less neat, but easier for me since I'm not as advanced as you.:smile:

Actually I think it confuses the issue, as does the current flow indicators, for the current flow changes at different times on both "sides" of the core.

 

[rattus]

Tell that to whoever decided the orientation of standard transformer diagrams. I just aligned my phasors to the diagrams to help clarify the issue... or so I thought!!!

Smart,

By definition, phasor angles measured in a CCW direction are positive. Phasor angles measured in a CW direction are negative. Therefore, a phasor @ 90 points straight up. A phasor @ -90 points straight down.

Sorry, but this clouds the issue rather than clarifying it.

 

[Smart $]

I was referring to the notion that your original drawing would indicate a negative rotation. So this statement is what I was questioning and I'm not sure if you were saying the first and second diagram indicated the same rotation but just had a notation difference:

Yes, the first and second diagram have the same rotation: electrically counterclockwise. The difference is the first uses positive angle notation (which is commonplace but rotation ends up appearing clockwise) and the second uses negative (φ).

I regret having mentioned it now as it appears to be a distraction from the original point which was the magnitude of the currents, not the phase angles. If it makes it easier, change the resistance to an impedance or use the 30 degree angle, the analysis is the same.

I don't see how changing resistance to impedance will make it any easier, because then we bring power factor into the discussion. FWIW, I have been using the same angle all along... only the notation has changed in an attempt to bring the discussion to your terms!

Which numbers? If you mean the angles, I should have left them out as it seems to be causing you a problem. If you mean the current magnitudes, what is the math error? Give me something to refer back to so I can have a starting point when I get a chance to look at this in more detail later.

The numbers for primary line currents. When you specified High voltage must lead low volatge across a delta-wye transformer bank, you changed the common node termination on the primary. Prior to this, the common node was B, but having chnaged the relationship, the common node is now A.

I believe the following graphic is more in line with how you are describing the situation.

The light bulb finally went "on" in my head regarding the 2x current through the common conductor. This is because winding currents are 180? out of phase from "core to core". However, going by the IEEE standard you mentioned earlier (HV leads LV by 30?), the currents would be Primary Line Conductors: A=2x, B=1x, C=1x. (Note this may change the numbers of your Line-Neutral solution above, too.)

 

[Smart $]

Smart,

By definition, phasor angles measured in a CCW direction are positive. Phasor angles measured in a CW direction are negative. Therefore, a phasor @ 90 points straight up. A phasor @ -90 points straight down.

Sorry, but this clouds the issue rather than clarifying it.

By rotation I mean phasors rotate in a CCW direction, not the angle measurement system. C lags B lags A by 120? each. In the CCW angle measurement system, the phasor angles are therefore negative from reference: 0?, -120?, and -240?.

 

[rattus]

Not really:

Not really:

Actually I think it confuses the issue, as does the current flow indicators, for the current flow changes at different times on both "sides" of the core.

We can stay with our original assumptions on current sense. Let Ild be at 0 degrees, then Iab is also at 0 degrees, then Ibc is at 180 degrees. We then subtract Iab from Ibc to obtain Ib. Or, we can define Icb which is in phase with Iab, therefore we add their magnitudes to obtain Ib. There are more than one correct way.

 

[mivey]

Actually I think it confuses the issue, as does the current flow indicators, for the current flow changes at different times on both "sides" of the core.

I meant the diagram was neater in that it did not have lines crossing like you would have with the polarity dots at the top and the common points on the bottom for the wye.

The idea was that if you could work your way through the diagram and resolve the currents, you would understand why the numbers I gave in #425 were correct.

As it seems the angles I used in #438 were confusing to you, let me re-state it:

...Draw three secondary coils oriented in a vertical stack. Let the top coil be A, the middle coil be B, and the bottom coil be C. Connect the bottoms of the coils together and bring a line out and call it N. The tops of the coils will be the A, B, & C line conductors. Bring a line out from A, through a load and back to B.

The current X leaves A and goes through the load, and enters the top of the B coil (Ia = -Ib). This current goes down through the B coil and exits at the common connection. We know X must enter the bottom of the A coil since it also leaves the top of the A coil. There is no remaining current left to run in the neutral so it has 0 current. C has zero current because it is open.

Now for the delta primary side (a,b,c with 1:1 ratio and no phase shift). Line up the primary coils next to the secondary coils and connect them with the bottom of a to the top of b, the bottom of b to the top of c, and the bottom of c back to the top of a. Let the line conductor a, b, c come in at the top of the respective coils.

Use the polarity such that coil a has X entering the top because coil A has X leaving the top. Coil b has X leaving because coil B has X entering. Line conductor b current (into the coils) = current entering bottom of coil a (-X) plus the current entering the top of coil b (-X) = -2X. X enters the bottom of coil b and since coil c has no current, the line conductor c has X coming into the coils. Also, since the coil c has no current, the line a conductor current is the same as the current entering coil a or X...

Try to follow this. I can still draw a picture if you think it will help but if you can work rattus' Brain_Twister, this should be cake.

[edit: I just noticed #452 has been posted. I'll catch up.]

 

[rattus]

Almost:

Almost:

[/QUOTE]

Smart, there is one problem with your diagram, The primary and secondary currents must oppose each other so that the load current has no effect on the magnetic flux.

 

[mivey]

Yes, the first and second diagram have the same rotation: electrically counterclockwise. The difference is the first uses positive angle notation (which is commonplace but rotation ends up appearing clockwise) and the second uses negative (φ).

If it is ok with you, it is ok with me, it is just different from the way I'm used to. Where have you seen this used in the electric industry? Do you have some books that use this method?

I don't see how changing resistance to impedance will make it any easier, because then we bring power factor into the discussion. FWIW, I have been using the same angle all along... only the notation has changed in an attempt to bring the discussion to your terms!

See #455 where I got rid of the angles. A non-resistive load can cause a power factor change and you can get whatever current angle makes you happy.

The numbers for primary line currents. When you specified High voltage must lead low volatge across a delta-wye transformer bank, you changed the common node termination on the primary. Prior to this, the common node was B, but having chnaged the relationship, the common node is now A.

I believe the following graphic is more in line with how you are describing the situation.

I'm not sure what you are saying about the common node, but I had A-B-C going down the left side, not C-A-B. BTW, very nice graphic. The secondary currents look good, the primary currents need work.

The light bulb finally went "on" in my head regarding the 2x current through the common conductor. This is because winding currents are 180? out of phase from "core to core". However, going by the IEEE standard you mentioned earlier (HV leads LV by 30?), the currents would be Primary Line Conductors: A=2x, B=1x, C=1x. (Note this may change the numbers of your Line-Neutral solution above, too.)

I have not changed my mind about my numbers in #425.

[edit: This may help: current flowing into a polarity dot on one winding flows out of the polarity dot on the other winding and they are essentially in phase]
[edit2: this too: the voltage drop from polarity to nonpolarity across one winding is essentially in phase with the voltage drop from polarity to nonpolarity across the other winding]

 

[rattus]

Rotation?

Rotation?

By rotation I mean phasors rotate in a CCW direction, not the angle measurement system. C lags B lags A by 120? each. In the CCW angle measurement system, the phasor angles are therefore negative from reference: 0?, -120?, and -240?.

Smart,

These are not rotating phasors! The length of the arrow is proportional to the RMS value of the voltage or current, and the phase angle represents the lead or lag of the waveform relative to the reference axis.

These are fixed (static) phasors. They are complex, constant numbers.

Rotating phasors are functions of time. We are working with steady state solutions.

 

[Smart $]

Smart,

These are not rotating phasors! The length of the arrow is proportional to the RMS value of the voltage or current, and the phase angle represents the lead or lag of the waveform relative to the reference axis.

These are fixed (static) phasors. They are complex, constant numbers.

Rotating phasors are functions of time. We are working with steady state solutions.

...and in those steady-state solutions, rotation must still be taken into consideration. Simply put, I cannot make a phasor rotate when I draw it on paper, can I? (i.e. without rotating the paper :grin: ) ...yet rotation still occurs in the system, right?

Why confuse the issue? You're trying to tell me things I already know!!!

 

[Smart $]

Smart, there is one problem with your diagram, The primary and secondary currents must oppose each other so that the load current has no effect on the magnetic flux.

Image replaced. Since it is linked, the updated image shows in the original post.

Does it conform now?

BTW, your Brain Teaser diagram has a problem too. You show clockwise current flow on both primary windings. It is impossible to have 2x current on Line B if each primary winding has 1x current going downward at B connection node!