Hameedulla-Ekhlas
Senior Member
- Location
- AFG
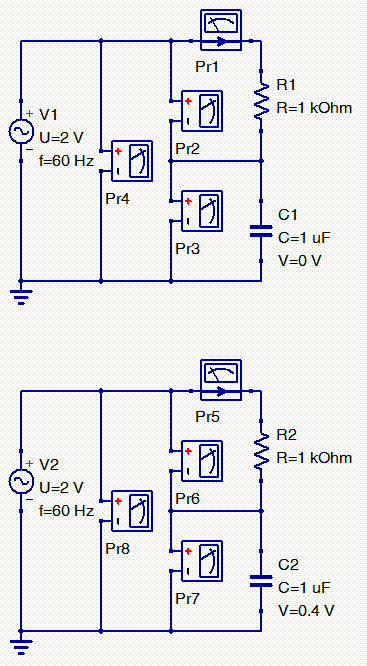
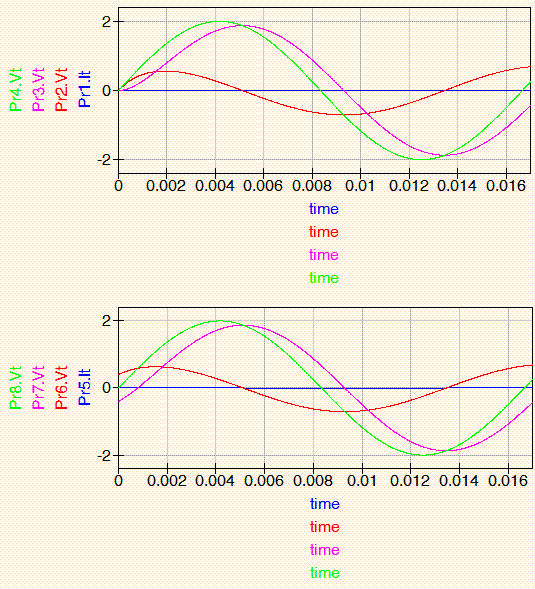
Ham, v(t) and i(t) are not constants; they are functions of time.
For the RC series circuit example, you could use any values of R and C you wish, e.g.,
R = 1/wC would provide a 45 degree leading current, but that would not be obvious if v(t) is a step function.
In such a circuit, the capacitor should have a initial charge or just without charge.