Good question. I have not defined it. It could also be defined in terms of (synchronous speed -rotation speed)/sync speed I suppose.
I see it used in one of models, but without a definition attached.
yepper
same thing
Good question. I have not defined it. It could also be defined in terms of (synchronous speed -rotation speed)/sync speed I suppose.
I see it used in one of models, but without a definition attached.
i add R' in parallel to an existing Z. what is I and XL in right gram?Wrong again. When you add more resistance in parallel the j term in the overall Z actually decreases. Look at my example again (j compared to j/2).

yepper
same thing
It seems to me that the definition you posted would be affected by motor efficiency unless you are very careful how you define Welec.
W = 2 Pi f
elec is field rotational speed 120 x freq/poles
mech is actual rotor rotational speed

Wmech = 2 Pi freq
Wmech = 2 Pi n, n is mech rev/sec of rotor
elec is field rotational speed 120 x freq/poles in rev/min
mech is actual rotor rotational speed in rev/min
No problem, understandableSorry. I forgot that there is a problem with Greek letters on the forum and W has nothing to do with Work.
Do you need help with the math?i add R' in parallel to an existing Z. what is I and XL in right gram?
i add R' in parallel to an existing Z. what is I and XL in right gram?
so are you saying that the R' that shows up related to 1/s reduces jX in some way?

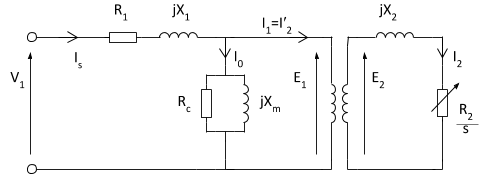
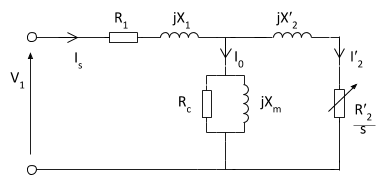
It is in series with X2 but in parallel with the whole left side of the model.
The network on the left side of the transformer(including the inductance of the transformer primary) is the only impedance seen when slip is zero, and therefore can be roughly considered to represent the no-load impedance of the motor, which is very crudely modeled as a fixed inductance in series with a fixed resistance (mostly the winding resistance).
What the network is approximated by in a given motor depends on the relative magnitude of the various R and X terms.
but that term shows s in bottom. when motor s goes down the torque goes up, so why is it R2'/s ??
In the condition of induction motor slip, the voltage remains the maximum and slip is 100% as the motor starts rotating but both slip as well as voltage reduce as soon as the rotor starts to turn. Frequency is directly proportional to the slip, i.e. frequency decreases with decrease in slip. Inductive reactance of an induction motor depends on both frequency as well as slip. When the rotor is stationary, the frequency, slip, and inductive reactance are at the maximum level. When the rotor turns, the inductive reactance remains low and power factor reaches to 1. The inductive reactance changes with slip because the rotor is the summation of constant resistance and changeable inductive reactance.
interesting how reactance changes.
As slip goes down the work done by the motor decreases. This is consistent with the 1/s resistive term approaching infinity.
How that corresponds to the available torque is slightly more complex, but basically torque also decreases to zero as the speed approaches synchronous speed (for an induction motor).
If you do not put a load on the motor, it cannot be supplying any torque, right?
How the torque varies in the vicinity of locked rotor (with s approximately 1) is an entirely different question.
What is that quote from?
It seems to me that the "frequency" being referred to is the rotational speed of the magnetic field relative to the rotor material, not a frequency in the same sense as the applied line frequency.
In that sense, at zero slip the rotor sees a static magnetic field in its own rotating reference frame and therefore there are no magnetic or circulating current losses in the rotor.
all textbooks about induction motors, thats where. the input reactance will decrease when motor is loaded, thus why a motor with no load can have very poor PF, but when loaded the PF can approach 1. see Steinmetz model.
NO!! Change your word reactance to resistance and it is correct!
that is the whole point! You keep wanting to morph the imaginary, 90 degree, Xm reactance into resistance to change it into in phase current increase with load. NO.
we keep telling u Xm, REACTANCE does NOT change with load.
Or maybe not. Look at the spreadsheet I offered.interesting how reactance changes.
Or maybe not. Look at the spreadsheet I offered.
Real machine, real application.

