Why is residential wiring known as single phase?

[Rick Christopherson]

No so. Treat them like force vectors and the opposing phases of the split phase system cancel--not expected.

Treat them like phasors and the opposing phases, subtracted phasorially, yield 240V-- as expected.

As expected by you! You've taken so many shortcuts over the years that you have forgotten that this is not the way vector math works. Your tail-to-tail representation of subtraction is a shortcut that you have taken. Through repetition and complacency, you have omitted the intermediate step of inverting the direction of a vector when performing subtraction. That much is more or less normal for most of us too. We do things so often that we forget about all of the proper steps in between.

 

[Besoeker]

I thought you understood how to different voltages could be interconnected?

Given that I design this sort of kit on a fairly sizable scale, then I think I can maybe reasonably claim to have at least a little inkling of how they work.
As it happens, one of my guys is on site at this very moment commissioning a 7,000A hexaphase SCR rectifier.

Va is connected to an SCR that is triggered to allow current to flow on the "1st half' of the cycle.
Vb is connected to an SCR that is triggered to allow current to flow on the "2nd half" of the cycle.

A cycle of a sine wave voltage has a positive half cycle and a negative half cycle. If the first half of the cycle is positive it follows that the second half is negative.
Now the SCR is a unidirectional device so it wouldn't conduct in the negative second half of the cycle.

So where does that pesky second pulse of current come from if all you have is single phase?
I have a sneaking suspicion that it might just be because another phase has somehow snuck in there.

 

[jim dungar]

But inversion does create a phase DIFFERENCE, therefore the waveforms are NOT identical. AC waves must have the same magnitude and phase to be identical.

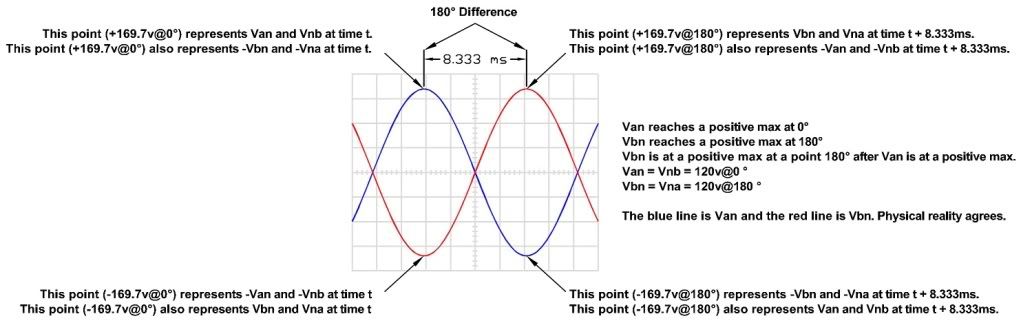
Van carries a phase of (wt)

Vbn carries a phase of (wt + PI)

I didn't add anything to the phase constant, the phase constant of Vbn is PI, period.

Again, a deceptive answer and a lecture on transformers.

What did I say that is deceptive?
I have never hidden the fact that I am focusing my participation, in this thread, on the case of a single center-tapped winding which produces a standard 120/240V system.
I thought by maintaining this consistency, it would be clear that I have not changed topics in 'midstream'.

In this post your are asking about two different waveforms Van and Vbn. Their relationship is indeterminate without a common reference.
In previous posts you used Vbn and -Vnb.

By choosing the single center-tapped winding, per the OP, we have two voltages with the relationship of V1=V2. This is a statement of fact. It is an equality based on physics. There are several other ways to name, identify, or assign direction to these voltages, but V1=V2 is a fact while their physical relation remains unchanged.

If you want to discuss other two voltage systems and their waveform analysis, you may do so in a different thread.

It has been mentioned to you several times that you are effectively creating a double negative, do you see why this has point has been raised?
Using the subscript conventions we discussed previously:
Vxy can only become Vyx be swapping, or inverting, the reference from point Y to point X, effectively changing the direction of the voltage.
V@0? can only become V@180? by adding/subtracting PI, or 180? to the phase constant, effectively changing the direction of the voltage.
Using Vyx@180? effectively changes the direction of the changed direction, resulting in no direction change at all.

I am patiently waiting for you to post the results of performing a subtraction (240V-120V=??) with your phasors.
But I am also waiting for someone, maybe Besoeker, to post the waveforms of Mivey's circuit of Vbn@180? sharing a common point with Vnb@0?. Maybe this would help you answer your question, which you accuse me of avoiding.

 

[rattus]

In this post your are asking about two different waveforms Van and Vbn. Their relationship is indeterminate without a common reference.

The common reference is the neutral as indicated by the second subscript. You surely know that. You are just stalling.

Fact is Van and Vbn are out of phase by PI radians which makes them not of the same phase. You know that too, or do you?

 

[gar]

120321-1354 EDT

Rick:

I gave you a reference in response to your request

I sure would like to see a reference that says phasors are not vectors.

Then you come back and still want to call them vectors. The point of Stout's discussion is that the thinking of electrical vectors, as had been done in years past, in the same way as mechanical vectors was creating confusion. Thus, a new way of naming and thinking was needed. Obviously the change started before 1952 and at that time was still in the process of evolution.

I do not want to go off on the tangent of arguing whether a phasor is a vector or not. There is far too much trouble trying to discuss the center tapped secondary.

.

 

[Rick Christopherson]

120321-1354 EDT

Rick:

I gave you a reference in response to your request

Then you come back and still want to call them vectors.

Understanding the English language must not be a prerequisite where you come from. As I already pointed out, your reference did not say that phasors are not vectors. Maybe you should reread it a third time.

 

[gar]

120321-1416 EDT

Jim:

Your statement

By choosing the single center-tapped winding, per the OP, we have two voltages with the relationship of V1=V2. This is a statement of fact. It is an equality based on physics. There are several other ways to name, identify, or assign direction to these voltages, but V1=V2 is a fact while their physical relation remains unchanged.

is only correct relative to magnitude. Your voltages are undefined relative to phase. The instantaneous voltage v_AN is just not equal to v_BN, and the subscripts do provide orientation information.

In a general AC circuit analysis there is no way you can just treat voltages as magnitudes only.

Why are some general purpose electricians confused by the occurrence in some circuits by 120 V from each leg to neutral, and leg to leg they do not read 240 V? Their meter is simply a magnitude meter, as is an incandescent light bulb or resistance heater. Similar problems occur measuring EGC to neutral voltages, or earth, and different points on earth.

.

 

[mivey]

Here is are some exercises:

a) Start with the given result of Vab@0?
Vab = |-------------------->

now subtract your voltage of 120@180?
Vbn = <----------|

Could you show us your resulting phasor and the steps it took you to get there?

b) Start with the given result of Vab@0?
Vab = |-------------------->

now subtract your voltage of 120@0?
Van = |---------->

Could you show us your resulting phasor and the steps it took you to get there?

Pure obfuscation. You know as well as the rest of us that it is not proper to do that. Would you do the same thing with the 208 line-line voltage and the associated line-neutral voltages?

Also, as rattus noted, you shouldn't use the angle and double subscripts together.

Actually I stated the fact, that a center-tapped winding is physically connected like a delta, 'identified terminal' connected to 'unidentified terminal' (i.e. X2 to X1) versus a wye where all of the 'identified terminals' or all of the 'unidentified terminals' are connected together (i.e. X2-X2).

Yes there are other possible connections of two isolated windings,but not of a center-tapped one.

More obfuscation. Why don't you try using a transformer that is like the center-tap, like the ones in Besoeker's hexaphase configuration or in the 5-wire quadrature phase configuration?

Inverting a waveform does not shift it in time.

As I have noted, a time shift is not required for you to have a phase shift.

You just don't seem to agree that you are the one adding PI, 180?, to the phase constnat and then you claim you have a different wave.

Nothing has been added. We started with Van and Vbn. We did not start with Van then shift it to get Vbn.

Besides, who is doing the real shifting? How do you know that Vbn and Vna are not the real un-shifted voltages? After all, it is likely that Van is the result of an inversion phase shift to negate the primary to secondary 180? phase shift in the first place. In other words, V@180? may be the true un-shifted voltage in the first place. Your "adding 180?" argument is hollow.

I am patiently waiting for you to post the results of performing a subtraction (240V-120V=??) with your phasors.

To what end? I may have missed a few posts, but is there really any question about how this should be done?

But I am also waiting for someone, maybe Besoeker, to post the waveforms of Mivey's circuit of Vbn@180? sharing a common point with Vnb@0?. Maybe this would help you answer your question, which you accuse me of avoiding.

The waveforms have been posted several times. I know Besoeker has in this thread but I did not feel like looking. But here is one I have handy from a prior thread:

 

[gar]

120321-1440 EDT

Rick:

If I have a mechanical force problem and I apply two forces to a point. Each force is 100 #. One is oriented at 0 degrees, and the other is at 90 deg. The resultant force is 144 # at 45 degrees.

If I draw a phasor diagram for a neutral based reference with two voltages, one is 100 V @ 0 deg, and the other is 100 V @ 90 deg, then the difference voltage between the two legs is 144 V @ -45 deg or 135 deg.

Quite different results.


Now change the 90 degree angle to 120 deg and the force result is 100 # @ 60 degrees.

For the electrical the result is 173 V @ -30 deg or 150 deg.


Then to 180 deg. Now the force is 0 # at an indeterminate angle.

The electrical result is 200 V @ 0 or 180 deg.

.

 

[Rick Christopherson]

120321-1440 EDT

Rick:

If I have a mechanical force problem and I apply two forces to a point. Each force is 100 #. One is oriented at 0 degrees, and the other is at 90 deg. The resultant force is 144 # at 45 degrees.

If I draw a phasor diagram for a neutral based reference with two voltages, one is 100 V @ 0 deg, and the other is 100 V @ 90 deg, then the difference voltage between the two legs is 144 V @ -45 deg or 135 deg.

Quite different results.

If you really want to get on my bad side, being deliberately deceptive is the quickest way. If you can't make a point with honesty, then you shouldn't try at all.

 

[gar]

120321-1518 EDT

Rick:

How am I being dishonest? I am describing how mechanical vectors and electrical voltage phasors are used.

In a mechanical system we sum the forces at a point. In an electrical system we sum the voltages around a loop, and we sum the currents at a point. Between any two voltage points we measure the voltage difference.

.

 

[Rick Christopherson]

How am I being dishonest? I am describing how mechanical vectors and electrical voltage phasors are used.

In a mechanical system we sum the forces at a point. In an electrical system we sum the voltages around a loop, and we sum the currents at a point. Between any two voltage points we measure the voltage difference.

.

This part is true, but this is NOT what you just demonstrated above with your vector example. Your vector example was not finding the difference between two points.

In an electrical system we sum the voltages around a loop, and we sum the currents at a point.

And here specifically is where Rattus' (and now yours by assumption) violate the proper rules for vector mathematics. Rattus has claimed that as he sums the voltages around a loop, when he encounters two vectors drawn tail-to-tail, it automatically signifies subtraction.

 

[mivey]

And here specifically is where Rattus' (and now yours by assumption) violate the proper rules for vector mathematics. Rattus has claimed that as he sums the voltages around a loop, when he encounters two vectors drawn tail-to-tail, it automatically signifies subtraction.

Subtraction would be a fair assessment.

For graphical addition, two phasors are drawn head-to-tail, then you draw the resultant phasor's tail at the first phasor's tail and the resultant phasor's head at the second phasor's head.

For graphical subtraction, two phasors are drawn tail-to-tail, then you draw the resultant phasor's head at the first phasor's head and the resultant phasor's tail at the second phasor's head.

Why do you take issue with this basic operation?

 

[jim dungar]

A cycle of a sine wave voltage has a positive half cycle and a negative half cycle. If the first half of the cycle is positive it follows that the second half is negative.

And believe it or not if the first half is negative, then the second half is positive.

Now the SCR is a unidirectional device so it wouldn't conduct in the negative second half of the cycle.

And it won't conduct in a negative first half of the cycle either.

So where does that pesky second pulse of current come from if all you have is single phase?

I am at a loss as to why you think the second pulse is pesky. You have two voltages, you have two SCRs, you need two firing pulses. Honestly this isn't rocket science.

I have a sneaking suspicion that it might just be because another phase has somehow snuck in there.

What part of two voltages, therefore two pulses do you think I am not understanding?

It has nothing to do with the phases it has to do with the relationship between the terminals of the SCRs in your wiring scheme.
Could you create the same output by simply swapping the direction of an SCR, and modifying its trigger?

 

[gar]

120321-1608 EDT

Rick:

It is common practice in a multi-phase circuit with a common reference point to position all the phasors associated with that point as graphically having their tails at that point. The Y connection. To determine the voltage difference between two head points a new phasor is drawn between those points and the new phasor between those points is calculated, and that is the voltage difference between the points.

If we were discussing currents at a point, then the results are like the mechanical force determination.

.

 

[jim dungar]

The common reference is the neutral as indicated by the second subscript. You surely know that. You are just stalling.

Stalling on what.
Every answer I give is framed by the specific conditions of a single center-tapped transformer. Honestly I am never sure that you are consistent in your questions.

Fact is Van and Vbn are out of phase by PI radians which makes them not of the same phase. You know that too, or do you?

For the single center-tapped winding.
Van and Vbn have the same phase constant. Yes, you have added PI radians during your manipulation of the values, but that does not change the base of Van=Vnb and Vbn=-Vnb therefore Van=-Vbn. Darn, that pesky equality issue.

 

[Besoeker]

You have two voltages, you have two SCRs, you need two firing pulses.

I couldn't have put it better.

 

[jim dungar]

Pure obfuscation. You know as well as the rest of us that it is not proper to do that. Would you do the same thing with the 208 line-line voltage and the associated line-neutral voltages?

Are you serious?
It is obfuscation to ask that a mathematical equation be able to be solved in other permutations?

Yes, I would expect any student taking a course to be able to solve a single equation for a for a single unknown.

 

[Rick Christopherson]

120321-1608 EDT

Rick:

It is common practice ....

So now we're just reverting the discussion to, "well that's just the way we (you) do it..." as an answer. Taking your cues from Rattus I see.

Please find a reference that says the resultant of two vectors is found by drawing a vector between the two tips of the vectors.

 

[jim dungar]

Besides, who is doing the real shifting? How do you know that Vbn and Vna are not the real un-shifted voltages? After all, it is likely that Van is the result of an inversion phase shift to negate the primary to secondary 180? phase shift in the first place. In other words, V@180? may be the true un-shifted voltage in the first place. Your "adding 180?" argument is hollow.

I have stated repeatetly that you may assign directions any way you want. I have said the relationship between the outputs of the two halves of a single center-tapped winding are consistent based on their relationship the primary, but I have not said which directions to use.

Rattus is the one that keeps bring up the addition of 180 when he uses sine(wt) and sin(wt+PI). I have tried to be consistent with his usage.

To what end? I may have missed a few posts, but is there really any question about how this should be done?

The waveforms have been posted several times. I know Besoeker has in this thread but I did not feel like looking. But here is one I have handy from a prior thread:

That is not a posting of the waveforms from the circuit where you had {Vbn@180? connected in parallel with Vnb@0?} which was in series with a parallel connection of {Van@0?and Van@0?}. i have been asking to see the B to N waveforms at the same time.

I am surprise you would not have noticed the difference.