Sorry for the delayed response... seemed to have missed your reply on earlier views.
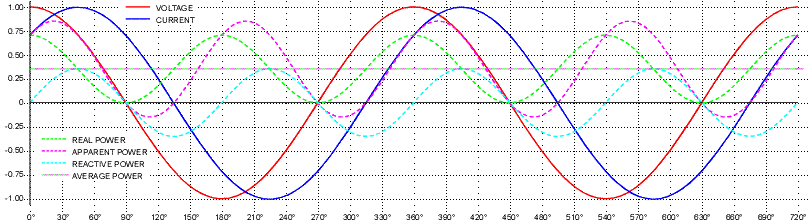
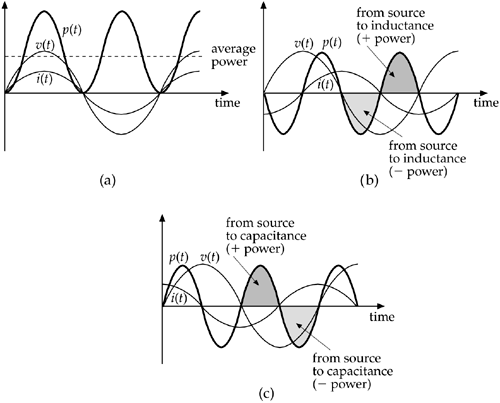
Anyway... I don't even recall how I got drawn into a Pavg discussion. When I mentioned the term "steady-state", I was referring to a flatline curve plot, i.e. always the same value when plotted vs. time. I believe somehow Pavg came up because you can't determine real and reactive components of instantaneous power without entering the time domain. This is because the waveform of both real and reactive power, though at double the frequency, are aligned to the voltage waveform (the powers' zero values occur at voltage's half- and quarter-cycle points, respectively) rather than aligned with the instantaneous power or current waveform. So the discussion of instantaneous real and reactive power values has to enter the time domain. Pavg, Vrms, and Irms are not in the picture.
First, I don't know why everyone keeps reverting to using plots of the sine function. Voltage and current waveforms, in discussing phase relationships and complex numbers, are determined and plotted with the cosine function.
That said it would be y = cos?(x) = (1+cos(2x))/2.
The reason we use rms values is because of the relationships P=EI, P=E?/R, and P=I?R, which are all the same formula with substitutions of Ohm's Law. For individual scenario, R is the same in the latter two. As such, P values are directly proportional to the values of E? and I?. Taking the mean value of instantaneous E? and I?, yields Vrms and Irms.
However, what we are discussing here is the basic P=EI, or rather P=VI, and being that real power is where V and Ireal are aligned sine waveforms, though possibly of differing values. We can although, determine the shape of the waveform in that each is essentially a scalar value (let's say "a" and "b" respectively) times cos(x). Thus no matter what their values are, the P waveform will be a?b?cos?(x), and this is where the sine function squared comes into play...