100415-1956 EST
Ham:
In the link you provided I have to disagree with the printed equation.
Make the phase angle 0, then the equation reduces to 1 + cos 2t. This wrong.
.
Using the attached two photos lets proof it where
p = P + Pcos2wt comes from .
p = vi --------1
This is instantaneous power is measured in watts when the
voltage is in volts and the vurrent is in ampere.
v = Vm cos(wt + 0v) ------2
i = Im cos(wt + 0i) ------3
0v voltage phase angle and 0i is current phase angle.
We are operating in the sinusoidal steady state, so we may
choose any convenient reference for zer time. Engineers
designing system that transfer large blocks of power have found
it convenient to use a zer time corresponding to the instant the
current is passing through a passitive maximum. This reference
system requies a shif of both the voltage and current by (0i)
thus eqs 2 and 3
v = Vm cos(wt + 0v - 0i),--------4
i = Im coswt.------------5
when we substitute Eqs 4 and 5 into Eq 1, the expression for the
instantaneous power becomes
p = Vm*Im cos(wt + 0v - 0i) coswt.----------6
Now by simply applying a couple trigonometric identities, we can
put Eq. 6 into a much more informative form.
we know this
cosx*cosB = 1*cos(x - B)/2 + 1*cos(x + B)/2
to expand equation 6 letting x = wt + 0v - 0i and B = wt gives
p = Vm*Im*cos(0v - 0i)/2 + Vm*Im*cos(2wt + 0v - 0i)/2 ----7
we know that
cos(x+B) = cosxcosB - sinxsinB
now we will expand the second term on the right hand side Eq. 7
which gives.
p = Vm*Im*cos(0v-0i)/2 + Vm*Im cos(0v - 0i)cos2wt/2 -
Vm*Im*sin(0v - 0i)sin2wt/2. ---------8
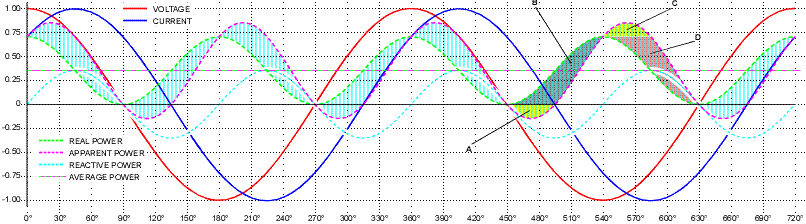
now see the attached graph file too.
graph depicts a representative relationship among v,i,and based
on the assumptions 0v=60 degre and 0i =0. You can see that the
frequencey of the instantaneous power is twice the frequency of
the voltage or current. this observation also follows directly
from the second two terms on the right hand side of Eq 8.
Therefore, the instantaneous power goes through two complete
cycles for every cycle of either the voltage or the curren. Also
note that the instantaneous power may be negative for a portion
of each cycle even if the network between the terminals is
passive. In a completely passive network, negative power implies
that energy stored in the inductors or capacitors is now being
extracted.
Now
We begin by noting that Eq 8 has threee terms which we can
rewrite as follows.
p = P + P*cos2wt - Q*sin2wt. -----------------9
P = Vm*Im*cos(0v - 0i)/2 -----------------10
Q = Vm*Im*sin(0v - 0i)/2 -----------------11
P is called the average power and Q is called the reactive
power.
If the circuit between the terminals is purely resistive the
voltage and current are in phase which means 0v = 0i. Equation 9
then reduces to
p = P + Pcos2wt.
This the method of how to prove the p = P + Pcos2wt.
Now if you are saying it is a wrong formula please proof it step by step and we all learn it.