Power factor and VA vs Watts

[Hameedulla-Ekhlas]

I have to add I disagree with the authors' implications in their figure (below)...

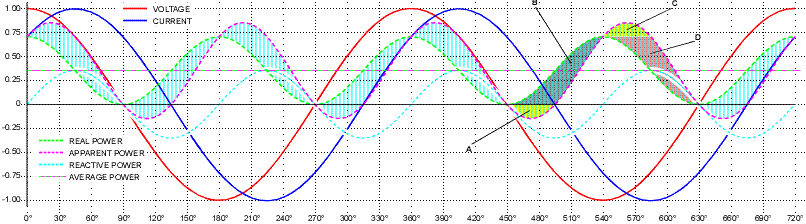
The authors state, "During the time interval corresponding to area "A", the source is delivering energy to the load,whereas during the time interval corresponding to area "B", a percentage of this amount of energy is being returned back to the source."

While their statement is not a complete fallacy, it erroneously implies that during the time interval corresponding to area "B" is the only time during which energy is being returned to the source. This implication is not true. At best, it is indicative only of some energy being returned to the source and is of the time interval of the leading half of energy being returned.

Referring to my latest chart below, my area "A" corresponds with the authors area "B". Pease note the overall hatched area with the line color cyan. The instantaneous magnitudes in this area are depicted by the "reactive power" waveform (dashed cyan). The time interval for both my areas "A" and "B" is the period which energy is being returned to the source, while the time interval corresponding to both my areas "C" and "D" is the period which energy is being stored by the load. There is only an instant between periods.

p = Vm*Im*cos(0v-0i)/2 + Vm*Im cos(0v - 0i)cos2wt/2 -

Vm*Im*sin(0v - 0i)sin2wt/2. ---------8

You can see that the

frequencey of the instantaneous power is twice the frequency of

the voltage or current. this observation also follows directly

from the second two terms on the right hand side of Eq 8.

Therefore, the instantaneous power goes through two complete

cycles for every cycle of either the voltage or the curren. Also

note that the instantaneous power may be negative for a portion

of each cycle even if the network between the terminals is

passive. In a completely passive network, negative power implies

that energy stored in the inductors or capacitors is now being

extracted.

 

[gar]

100416-0731 EST

Smart $ and Ham:

If you define the input voltage across a resistance as being a sine wave, then your equation with + is wrong. I you define the input waveform as being a cosine wave, then your equation is correct.

Ham when you originally posted the equation with the + I do not believe you defined that this was for a cosine waveform.

The trig identities for sine squared and cosine squared are mathematically provable and are correctly shown in many books.

The photo from the book that showed instantaneous power as what appeared to be approximately a full wave rectified sine wave is wrong.

.

 

[ggunn]

...and what you refer to as context is what I meant when I used the terms "instantaneous domain" and "RMS domain" a while back. Yeah, I told you to forget I mentioned it... but it's back

In a function, the domain is all the values that x can take on (the range is all the values y can have). I'm guessing that when you refer to the "instantaneous domain", you are referring to what I know as the "time domain", but I don't grasp what you mean by the "RMS domain". Can you define that for me, or if you have already somewhere in this thread, can you point me to it? Thanks.

 

[gar]

100416-0914 EST

Back two days ago I ask a question in post 191 and both steve066 in post 193 and LarryFine in post 195 responded and both answers were correct.

.

 

[Besoeker]

Okay... I'll not debate the matter with you on the condition you not debate it with me either.

There is nothing to debate.

 

[Smart $]

There is nothing to debate.

So now you want to debate about debating :roll:

 

[ggunn]

So now you want to debate about debating :roll:

I want to debate whether or not it's appropriate to debate about debating in this forum.

 

[Smart $]

In a function, the domain is all the values that x can take on (the range is all the values y can have). I'm guessing that when you refer to the "instantaneous domain", you are referring to what I know as the "time domain", but I don't grasp what you mean by the "RMS domain". Can you define that for me, or if you have already somewhere in this thread, can you point me to it? Thanks.

Well in the examination of this subject there are two trains of thought that go unmentioned. Regarding the word and implications of "instantaneous":


RMS domain is when we adhere to the concept power is a scalar physical quantity.

Instantaneous domain permits power to be described as a vector physical quantity.

 

[Smart $]

I want to debate whether or not it's appropriate to debate about debating in this forum.

There is nothing to debate :roll:

 

[LarryFine]

Well, at least there's one thing here I understand. :roll:

Back two days ago I ask a question in post 191 and both steve066 in post 193 and LarryFine in post 195 responded and both answers were correct.

Okay, make that two things.

 

[Smart $]

...

p = Vm*Im cos(wt + 0v - 0i) coswt.----------6

Now by simply applying a couple trigonometric identities, we can

put Eq. 6 into a much more informative form.

we know this

cosx*cosB = 1*cos(x - B)/2 + 1*cos(x + B)/2

...

I was following you until you got to here.

Last time I checked (which was just a couple minutes ago )...

cos(x)*cos(b) = [cos(x-b) + cos(x+b)]/2​

 

[Smart $]

Well, at least there's one thing here I understand. :roll:

A lot of people understand it as depicted... but certain authoritative figures say power is scalar, so applying vector math as depicted would be an invalid calculation

 

[Smart $]

I was following you until you got to here.

Nevermind... I see what you are doing.

 

[Smart $]

...In a completely passive network, negative power implies

that energy stored in the inductors or capacitors is now being

extracted.

Yes, the negative power portion accurately implies stored power is being released. However, after the negative portion of the instantaneuos power curve, not all stored power has been released. Releasing of power continues even after the instantaneous power curve goes positive, as I so indicated in my picture and post.

 

[ggunn]

Well in the examination of this subject there are two trains of thought that go unmentioned. Regarding the word and implications of "instantaneous":


RMS domain is when we adhere to the concept power is a scalar physical quantity.

Instantaneous domain permits power to be described as a vector physical quantity.

That does not define either one of them. The term "domain" means something in particular, i.e., the x axis in the Cartesian coordinate system. When plotting in the time domain (which is what I have deduced from context that you mean by "instantaneous domain", though I have never heard it referred to as such anywhere else), the x axis (the domain) is time, hence the name. The extent of the data in the y direction is the range. All those d/dt terms refer to the slope (the ratio of change within the range to the change in domain) of whatever data you are plotting on the y axis against time. This is all first semester calculus; forgive me if it's old news to you.

I haven't a clue as to what you mean by "RMS domain". Please enlighten me.

 

[Smart $]

That does not define either one of them. The term "domain" means something in particular, i.e., the x axis in the Cartesian coordinate system. When plotting in the time domain (which is what I have deduced from context that you mean by "instantaneous domain", though I have never heard it referred to as such anywhere else), the x axis (the domain) is time, hence the name. The extent of the data in the y direction is the range. All those d/dt terms refer to the slope (the ratio of change within the range to the change in domain) of whatever data you are plotting on the y axis against time. This is all first semester calculus; forgive me if it's old news to you.

I haven't a clue as to what you mean by "RMS domain". Please enlighten me.

Your explanation of the word domain is too restricted a context.

Merriam-Webster Collegiate? Dictionary

do?main
4 : a sphere of knowledge, influence, or activity <the domain of art>
5 : the set of elements to which a mathematical or logical variable is limited specifically : the set on which a function is defined

...and there are 8 other defintions that aren't really pertinent to my usage of the word.

Nevertheless, there are two schools of thought regarding instantaneous power...

One school, like you said in an earlier post, is that power is averaged over a time period, therefore power cannot exist in an instant. This is in the RMS power domain of thought.

The other school says that power varies with the current and voltage being input to a load. We can measure the variance in power simply by taking instaneous measures of voltage and current and determine instantaneous power based on the voltage and current properties for intervals both preceding and after the point of measure. This is in the instantaneous power domain of thought.

 

[ggunn]

Your explanation of the word domain is too restricted a context.

In the realm of mathematics and engineering, that's what "domain" means (it's meaning #5 in what you cited), and yes, it is very restrictive because it strives to be unambiguous. You are playing fast and loose with the terminology. I have never heard of anyone else in the engineering world speak of the "instantaneous domain" or the "RMS domain"; I think you are making up your own nomenclature. I don't know how you can expect what you say to be taken seriously if you do that.

I've told you what the time domain is; can you tell me what the "RMS domain" is? Not what it does, or what it allows you to do, but what, succinctly and unambiguously it is? Define it.

I don't claim to know everything. If you can point me to any serious electrical engineering reference, anywhere, that uses those terms in the sense that you are using them (and can make sense of what they mean), then I yield.

 

[Cold Fusion]

Your explanation of the word domain is too restricted a context....

...Nevertheless, there are two schools of thought regarding instantaneous power...

One school,..., is that power is averaged over a time period, therefore power cannot exist in an instant. This is in the RMS power domain of thought.

The other school says that power varies with the current and voltage being input to a load. We can measure the variance in power simply by taking instaneous measures of voltage and current and determine instantaneous power based on the voltage and current properties for intervals both preceding and after the point of measure. This is in the instantaneous power domain of thought.

Smart-
I highlighted a couple of the more interesting parts. You're ... ummm ... giving some unusual definitions of the normal math models - but certainly interesting.

therefore power cannot exist in an instant: Normally one would think that it could. Power is the first derivative of the energy transfer. That would usually be considered instantaneous.

determine instantaneous power based on the voltage and current properties for intervals both preceding and after the point of measure
This one just baffles me. if the power is instantaneous, why do we care what the voltage and current properties are for the pre and post intervals around the point of measure? Okay, I'm really confused where this one is going.:-?

As for the restrictive definition:
This is a math discussion - not english composition. I think gg is right - if you are going to use a non-standard definition, it would be good to let us know.

Just a thought.

cf

 

[Smart $]

(it's meaning #5 in what you cited)

...and I'm using the word in the context of definition #4

I think you are making up your own nomenclature.

That is correct. I'm not referencing any book. I write what is in my head.

That's the problem with types that so-called learned this in school. The school or prof decided what they learned, and for the sake of succeeding, they wrapped theirr heads around the concepts taught. Some free thinkers make it through this process, while many believe their course texts are the equivalent of a "bible", and there is no other acceptable documentation.

I've told you what the time domain is; can you tell me what the "RMS domain" is? Not what it does, or what it allows you to do, but what, succinctly and unambiguously it is? Define it.

You told me what your domain is. It is not the same as my usage of the word. The word has not less than 10 meanings according to only one dictionary. Show me where I have to use your meaning for the word. Otherwise, get your head out of the sand.

I don't claim to know everything....

It is good that you don't, but on the other hand you are attempting to play me small. So how is the air up there?

 

[Smart $]

...

therefore power cannot exist in an instant: Normally one would think that it could. Power is the first derivative of the energy transfer. That would usually be considered instantaneous.

Normally one would think? What is normally? When you say it, it likely means you assuming the majority of others think like you. That may or may not be true. Yet even if it were true, there are those that do not. AFAICT, I'm not one of them. So trying to convince me of something differnet than what I observed yet do not adhere to is a waste of your time... IMO.

determine instantaneous power based on the voltage and current properties for intervals both preceding and after the point of measure
This one just baffles me. if the power is instantaneous, why do we care what the voltage and current properties are for the pre and post intervals around the point of measure? Okay, I'm really confused where this one is going.:-?

If I tell you I had a reactive load for which I measured 56V and 3A at one instant, can you tell me the real and reactive power portions of the current? No you cannot. There is not enough information to do so. AT best, you can only tell me what the apparent power is at the point in time. Now as I supply enough information to determine the answer, I am actually telling you what happened before and after the point in time the measure was taken.

As for the restrictive definition:
This is a math discussion - not english composition. I think gg is right - if you are going to use a non-standard definition, it would be good to let us know.

Just a thought.

I have been. Coupled with that, I didn't see any guidelines that said I had to restrict my usage of the english language... even in what you and ggunn seem to be saying is limited to a math discussion. I embody knowledge as a whole, and use it as such. I shall not lessen myself for the likes of others. Sorry if you can't understand... but that is just the way it is.