Realistically, they may vary from 120?, as in your latest example... but we assumed ideal voltage parameters for the david's example, did we not???...They should be 120? apart with purely resistive loads. Since they are not, this is indicative the magnitudes are in error also.
You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Calculating Phase Currents Based on Measured Line Currents - Delta Heater Load
- Thread starter jakeself
- Start date
- Status
- Not open for further replies.
Seems to me:
Seems to me:
Haven't followed this closely, so someone may have said this already, but here is my approach:
Express the line to line voltages as,
Vab = 208V@0
Vbc = 208V@-120
Vca = 208V @-240
Measure Ia, Ib, and Ic and reference their angles to zero.
Write a set of equations for the REAL parts of Ia, Ib, and Ic, and reference their angles to zero.
Let Iab, Ibc, and Ica be the unknowns with their angles referenced to zero.
We now have three equations and three unknowns. Should be solvable, but I am too lazy to try.
You could do this for the imaginary parts as well, but once you know the real parts, it is easier to just compute the imaginary parts.
Any takers?
Seems to me:
Haven't followed this closely, so someone may have said this already, but here is my approach:
Express the line to line voltages as,
Vab = 208V@0
Vbc = 208V@-120
Vca = 208V @-240
Measure Ia, Ib, and Ic and reference their angles to zero.
Write a set of equations for the REAL parts of Ia, Ib, and Ic, and reference their angles to zero.
Let Iab, Ibc, and Ica be the unknowns with their angles referenced to zero.
We now have three equations and three unknowns. Should be solvable, but I am too lazy to try.
You could do this for the imaginary parts as well, but once you know the real parts, it is easier to just compute the imaginary parts.
Any takers?
The part in red is the real kicker. If we know the magnitude AND angle of the line currents, as you stated, we can determine phase currents using vector[-based?] math. Knowing the line currents' magnitude and not their angles throws water on the fire.Haven't followed this closely, so someone may have said this already, but here is my approach:
Express the line to line voltages as,
Vab = 208V@0
Vbc = 208V@-120
Vca = 208V @-240
Measure Ia, Ib, and Ic and reference their angles to zero.
Write a set of equations for the REAL parts of Ia, Ib, and Ic, and reference their angles to zero.
Let Iab, Ibc, and Ica be the unknowns with their angles referenced to zero.
We now have three equations and three unknowns. Should be solvable, but I am too lazy to try.
You could do this for the imaginary parts as well, but once you know the real parts, it is easier to just compute the imaginary parts.
Any takers?
As for takers, I see no one has commented on my solution in Post#73...
Any takers?
Just the formula to determine the angle of Iab relative to Ia@0? is:
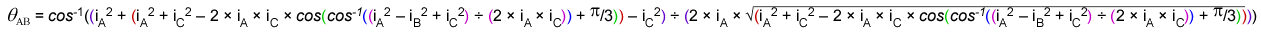
...where "i" denotes current magnitude only.
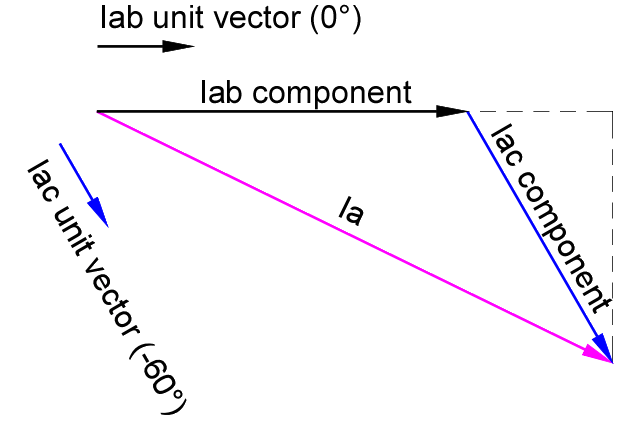
Well if you want to pursue it from known magnitudes and angles, I'll start the solution process with a graphic representation of how to determine Iab and Iac components of Ia...Smart,
It is implied that one would measure the angles of the line currents as well as their magnitudes. Otherwise, you have 6 unknowns and 3 equations.
Sure, but:
Sure, but:
You still must know the angles of Ia, Ib, and Ic in order to find the magnitudes of Iab and Iac.
My way is the classic method utilizing simultaneous equations and determinants. And, it can be "programmed" so to speak into a spreadsheet.
Sure, but:
Well if you want to pursue it from known magnitudes and angles, I'll start the solution process with a graphic representation of how to determine Iab and Iac components of Ia...

You still must know the angles of Ia, Ib, and Ic in order to find the magnitudes of Iab and Iac.
My way is the classic method utilizing simultaneous equations and determinants. And, it can be "programmed" so to speak into a spreadsheet.
That's what I said.You still must know the angles of Ia, Ib, and Ic in order to find the magnitudes of Iab and Iac.Well if you want to pursue it from known magnitudes and angles, I'll start the solution process with a graphic representation of how to determine Iab and Iac components of Ia...
...
Analogy: Its not how you play the game, but the final score which determines the winner.My way is the classic method utilizing simultaneous equations and determinants. And, it can be "programmed" so to speak into a spreadsheet.
And so far, you are just a spectator
...and while you're spectating, here's a spreadsheet solution, when magnitudes and angles are known, without using simultaneous equations and determinants...
https://docs.google.com/uc?id=0By0r...FlNTU1&export=download&authkey=CIrqqcEB&hl=en
https://docs.google.com/uc?id=0By0r...FlNTU1&export=download&authkey=CIrqqcEB&hl=en
I may have read this wrong for my first response... but if you are saying we must know Ib and Ic in addition to Ia, in order to determine Iab and Iac, no we don't.You still must know the angles of Ia, Ib, and Ic in order to find the magnitudes of Iab and Iac.
Given only Ia(r,θ) and standard voltage angles, we can determine Iab and Iac without any additional information.
mivey
Senior Member
I went through that exercise briefly and had no better luck but I may have fat-fingered the whole thing. I'll have to wait until I have time to concentrate and actually feel like it. The best I could tell, I am finding alternate solutions with the neutral point in various places. I'm tired of looking at it....without using simultaneous equations and determinants...
You're not giving me much to go on to help you through it...I went through that exercise briefly and had no better luck but I may have fat-fingered the whole thing. I'll have to wait until I have time to concentrate and actually feel like it. The best I could tell, I am finding alternate solutions with the neutral point in various places. I'm tired of looking at it.
Refer to graphic. Technically, Ia is actually Ian (where n is real or virtual)... so the neutral point will always be at the tail end of Ian.
If you have a delta connected, purely-resistive, unbalanced load. The voltage and current angles of the loads would be the standard ab@0?, bc@-120?, and ca@-240?... thus Iab(θ)@0? and Iac(θ)@-60? (i.e. the inverse angle of Ica(θ))@-240?). Once you know the magnitude and angle of Ian (the angle of which must be somewhere between 0? and -60?), a triangle is formed where the magnitude of the long side and angles of all three vectors are known, leaving only the magnitudes of the short sides to be calculated.
The rest is calculated on KCL where Ian+Ibn+Icn=0 (a requirement) and so forth. In determining Ibc and Ica, the graphic would be rotated in -120? increments from Iab's referengle of 0? (and annotations altered accordingly), and both Iba and Ica would be known after calculating Iab and Iac from Ian(r,θ).
If you still do not grasp it, I can provide if need be more graphics to illustrate the process...
If you are referring to the linked spreadsheet, let me know where you have issues...
Last edited:
mivey
Senior Member
I was referring to other solution methods. Plus, I am using these values:You're not giving me much to go on to help you through it...
For example, a wye-delta solution gets me this:Vab = 482.000000000<0.000000000?
Vbc = 479.000000000<-121.000000000?
Vca = 473.226244709<119.816136066?
which convert to:
Va = 278.282829749<-30.000000000?
Vb = 276.550778942<-151.000000000?
Vc = 273.217299771<89.816136066?
I also have resistances of:
Zab = 12.000000000<0.000000000?
Zbc = 8.000000000<0.000000000?
Zca = 4.800000000<0.000000000?
and so have currents of :
Iab = 40.166666667<0.000000000?
Ibc = 59.875000000<-121.000000000?
Ica = 98.588800981<119.816136066?
which produce measured values of:
Ia = 123.576152816<-43.803702128?
Ib = 87.611006252<-144.140179271?
Ic = 138.063536773<97.567541062?
Vxn / Ix = Zx =
Za = 2.229379700<14.016731618?
Zb = 3.182298527<-6.461016438?
Zc = 1.982771871<-8.363361485?
which transform to (not the original values):
Zab = 8.842812564<7.559163358?
Zbc = 7.864645049<-14.820929744?
Zca = 5.509627669<5.656818311?
which yield (Vxy / Zxy) but not the original values:
Iab = 54.507544578<-7.559163358?
Ibc = 60.905482326<-106.179070256?
Ica = 85.890784844<114.159317755?
which yield the original measured values of:
Ia = 123.576152816<-43.803702128?
Ib = 87.611006252<-144.140179271?
Ic = 138.063536773<97.567541062?
A matrix solution gets me this:
We know
[X]=[A^-1]=[1/Zxy]
where
[A]=
(Vab) (0) (-Vca)
(-Vab) (Vbc) (0)
(0) (-Vbc) (Vca)
[X]=
(1/Zab)
(1/Zbc)
(1/Zca)
=
(Ia)
(Ib)
(Ic)
so we have
[A11 A12 A13]
[A21 A22 A23]
[A31 A32 A33]
=
(482) (0) (235.296762118084-410.583137036312i)
(-482) (-246.703237881916-410.583137036312i) (0)
(0) (246.703237881916+410.583137036312i) (-235.296762118084+410.583137036312i)
and Det(A) = (2.90106981992722E-007i)
and [A^-1]
=
(-16143377761.7951-781184182261.172i) (-16143377761.7951-781184182261.172i) (-16143377761.7951-781184182261.172i)
(682165836520.497+390935228659.065i) (682165836520.497+390935228659.065i) (682165836520.497+390935228659.065i)
(-682165836520.497+409886586811.162i) (-682165836520.497+409886586811.162i) (-682165836520.497+409886586811.162i)
and
[B1] = 123.576152816<-43.803702128?
[B2] = 87.611006252<-144.140179271?
[B3] = 138.063536773<97.567541062?
thus
1/Zab = (0.3125+3.90625E-003i)
1/Zbc = (-9.375E-002+0.203125i)
1/Zca = (-0.1015625-0.171875i)
yielding
Zab = 3.199750029<-0.716159945?
Zbc = 4.469953893<-114.775140569?
Zca = 5.009027073<120.579226872?
which is not what we started with
With a second matrix calculation I got:
started the same as before but got these results instead
Det(A) = (.219915835176+6.53912549928i)
and [A^-1]
=
(448842180731-34641.9814791i) (448.840105042-34641.9814791i) (448.841044341-34641.9774967i)
(29647.333577+18340.8279498i) (29647.3335777+18340.8279498i) (29647.3307317+18340.8249057i)
(-30840.8278737+17147.3349568i) (-30840.8278737+17147.3349568i) (-30840.8248296+17147.3321108i)
and
[B1] = 123.576152816<-43.803702128?
[B2] = 87.611006252<-144.140179271?
[B3] = 138.063536773<97.567541062?
thus
1/Zab = (-3.8726777+2.258596882i)
1/Zbc = (0.22713485-4.582748959i)
1/Zca = (4.20774911+2.352134722i)
yielding
Zab = 0.223056013909<-149.748697294?
Zbc = 0.217942117499<87.1625703428?
Zca = 0.207445262005<-29.205221179?
which is such a crazy number I think I must have mis-calculated one of the steps.
I have not checked these calcs yet so help yourself if you feel froggy.
Last edited:
I believe this is at the heart of the problem.I was referring to other solution methods. Plus, I am using these values:
Vab = 482.000000000<0.000000000?
Vbc = 479.000000000<-121.000000000?
Vca = 473.226244709<119.816136066?
which convert to:
Va = 278.282829749<-30.000000000?
Vb = 276.550778942<-151.000000000?
Vc = 273.217299771<89.816136066?
I also have resistances of:
Zab = 12.000000000<0.000000000?
Zbc = 8.000000000<0.000000000?
Zca = 4.800000000<0.000000000?
and so have currents of :
Iab = 40.166666667<0.000000000?
Ibc = 59.875000000<-121.000000000?
Ica = 98.588800981<119.816136066?
which produce measured values of:
Ia = 123.576152816<-43.803702128?
Ib = 87.611006252<-144.140179271?
Ic = 138.063536773<97.567541062?
...
Line-to-line voltage angles can vary due to line-to-neutral voltage magnitudes and angles departing from nominal (i.e. either or a combination of both). However, no matter which, the neutral voltage point, be it virtual or real, must be common to all L-N vectors. If we take your L-L voltages and arrange the vectors head to tail in a triangle, and your L-N vectors heads to the triangle's vertices...
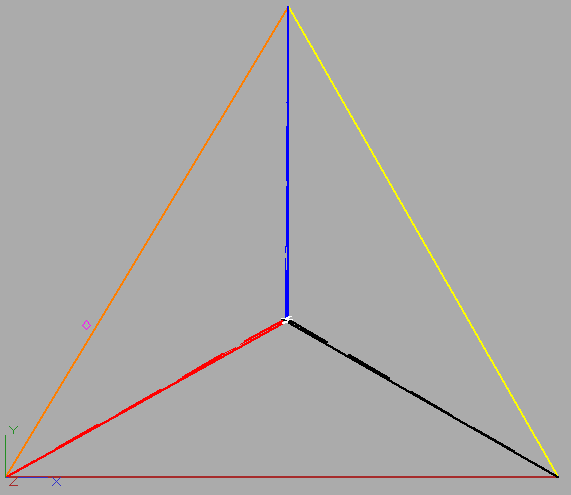
...your L-N vectors tails should coincide at a single neutral point... but they do not.
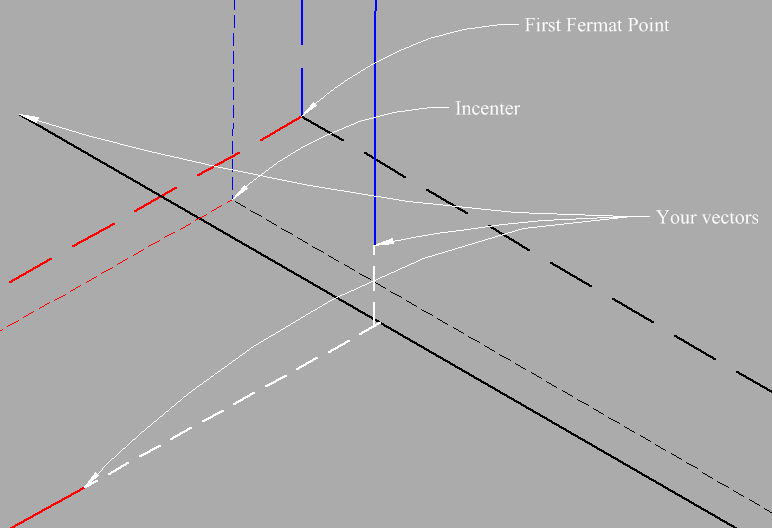
Even if we extended the two shorter ones (white dashed lines represent extentions in illustration), they don't even meet at a single point.
I believe you are assuming the line voltage source is a wye secondary. There is no way to know whether the L-L voltage angle shift from nominal is a result of voltage or angle deviation, or a combinaiton of both.
Regardless, the delta load will find its own virtual neutral voltage point (since there is no real neutral voltage point). I am uncertain how it does that at the moment (perhaps for all eternity too :roll
First Fermat Point vectors
Va=274.9563362159<-30.3946417627
Vb=281.5961211068<-150.3946417626
Vc=271.4745657892<-270.3946417626
Incenter vectorsVb=281.5961211068<-150.3946417626
Vc=271.4745657892<-270.3946417626
Va=275.2046453513<-30.0919319670
Vb=280.2151795898<-150.5000000000
Vc=272.6131289725<-270.5919319669
Vb=280.2151795898<-150.5000000000
Vc=272.6131289725<-270.5919319669
I'd try 'em myself, but my mind is distracted with another "real" project.
- Status
- Not open for further replies.

