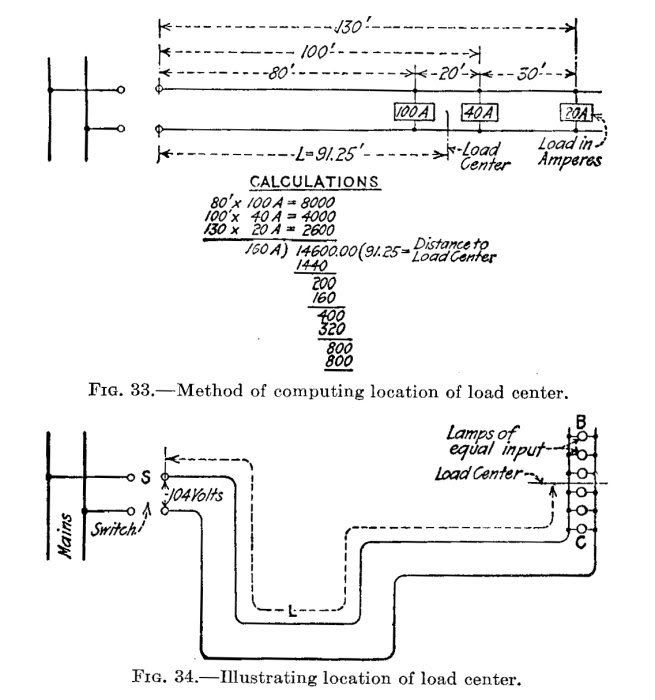
151023-2051 EDT
spraymax6:
Having read some of the posts I feel you have not been clear on the statement of the problem, nor of the assumptions you have made.
Using the assumption that the three phase loading is balanced. This is not quite correct, but probably sufficiently close for your real world purposes. Then there is no difference in the voltage drop along a hot wire between wye and delta loading. This means that we assume the voltage drop on the neutral is not significant.
But there is a big differencre if you use three separate neutrals for what otherwise would be a wye connection.
In your spread sheet you use a line to neutral voltage of 120 V, but in post 1 you stated 277 V. This is a huge error. Also in your spread sheet your cummulative current column is upside down. The largest line current occurs at the input of the distribution system, not the end. Anoher problem is that it is likely that current per fixture drops as you progress toward the end of the line. But again for practical reasons your constant current value per light is sufficiently good. You don't need high accuracy for your study. Also displayed values in your spread sheet have too many significant figures. Cut the displayed decimal places to possibly 2 or 1 places for current.
Fundamentally you need to know the voltage drop along one hot wire. Only if you use a separate neutral for each phase do you multiply by 2, and this is becuse there is voltage drop on two wires. In this case you probably should not call it a neutral. Back to assumption of a delta load, or a wye with zero neutral current, then voltage drop on that one hot wire is resistance of that wire times the current in that wire. There is no factor of 2 or 1.732 . the easy way to visualize that current is to assume a wye load and zero neutral current.
Don't just plug numbers into some equation. You need to know how the equation relates to your circuit.
.